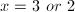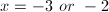All Algebra II Resources
Example Questions
Example Question #1 : Quadratic Equations And Inequalities
Solve the equation for 
Cross multiply.
Set the equation equal to zero.
Factor to find the roots of the polynomial.

Example Question #1 : How To Use The Grid Method For Foil
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Example Question #1 : Quadratic Equations And Inequalities
Solve the equation for 
1. Cross multiply:
2. Set the equation equal to 
3. Factor to find the roots:


Example Question #1 : Simplifying And Expanding Quadratics
If you were to solve 

When given a quadratic in the form 




To complete the square we want to add a number to each side which yields a polynomial on the left side of the equals sign that can be simplified into a squared binomial 



We add 
We then factor the left side of the equation into binomial squared form and combine like terms on the right:
Example Question #1 : Quadratic Equations And Inequalities
If you were to solve 

When given a quadratic in the form 




To complete the square we want to add a number to each side which yields a polynomial on the left side of the equation that can be simplified into a squared binomial 



We add 
We then factor the left side of the equation into binomial squared form and combine like terms on the right:
Example Question #1 : Quadratic Equations And Inequalities
Expand:
None of the other answers are correct.
Use the FOIL method, which stands for First, Inner, Outer, Last:
Example Question #3 : Quadratic Equations And Inequalities
Multiply:
Example Question #4 : Understanding Quadratic Equations
Multiply:
Example Question #171 : Intermediate Single Variable Algebra
Subtract:
When subtracting trinomials, first distribute the negative sign to the expression being subtracted, and then remove the parentheses:
Next, identify and group the like terms in order to combine them: 
Example Question #172 : Intermediate Single Variable Algebra
Evaluate the following:
When multiplying this trinomial by this binomial, you'll need to use a modified form of FOIL, by which every term in the binomial gets multiplied by every term in the trinomial. One way to do this is to use the grid method.
You can also solve it piece-by-piece the way it is set up. First, multiply each of the three terms in the trinomail by 

Finally, you can combine like terms after this multiplication to get your final simplified answer:
All Algebra II Resources