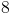The equation of a circle is , in which (h, k) is the center of the circle and r is its radius. Because the graph of the circle is centered at (2, -3), h and k are -2 and 3. Because the radius is 4, the right side of the equation is equal to 16.

The formula for a circle of radius , centered at the point  is given by the general equation:

In this case, the radius is the square root of , which is six, and the center is at 

The standard equation for a circle is:

Therefore, the radius is 6 and the center is located at (0,-2)

To find the center or the radius of a circle, first put the equation in the standard form for a circle:  , where  is the radius and  is the center.

From our equation, we see that it has not yet been factored, so we must do that now. We can use the formula  . 

, so .

 and , so  and .

Therefore, .

Because the constant, in this case 4, was not in the original equation, we need to add it to both sides:

Now we do the same for :

We can now find :

To find the center or the radius of a circle, first put the equation in standard form:  , where  is the radius and  is the center.

From our equation, we see that it has not yet been factored, so we must do that now. We can use the formula  . 

, so .

 and , so  and .

This gives .

Because the constant, in this case 9, was not in the original equation, we must add it to both sides:

Now we do the same for :

We can now find the center: (3, -9)

Remember that the "shifts" involved with circular functions are sort of like those found in parabolas. When you shift a parabola left or right, you have to think "oppositely". A right shift requires you to subtract from the x-component, and a left one requires you to add. Hence, this circle has no horizontal shift, but does shift 6 upward for the vertical component.

You can also remember the general formula for a circle with center at  and a radius of .

Comparing this to the given equation, we can determine the center point.

The center point is at (0,6) and the circle has a radius of 5.

Remember that the shifts for circles work in an opposite manner from what you might think. They are like the parabola's x-component.  Hence, a subtracted variable actually means a shift up or to the right, for the vertical and horizontal components respectively. Since the x-component has a "+5", it is shifted left 5. Since the y-component has a , it is shifted upward 12. Therefore, this circle has a center at .

You can also remember the general formula for a circle with center at  and a radius of .

Comparing this to the given equation, we can determine the center point.

The center point is at  and the circle has a radius of 6.

Remember that for the equation of a circle, the lone number to the right of the equals sign is the radius squared.

The general formula for a circle with center at  and a radius of  is:

Comparing this to the given equation, we can determine the radius.

The center point is at  and the circle has a radius of 9.

Remember that the "shifts" involved with circular functions are sort of like those found in parabolas. When you shift a parabola left or right, you have to think "oppositely". A right shift requires you to subtract from the x-component, and a left one requires you to add. Hence, this circle has a positive 3 horizontal shift, and a negative 2 vertical shift.

You can also remember the general formula for a circle with center at  and a radius of .

Comparing this to the given equation, we can determine the radius and center point.

The center point is at  and the circle has a radius of 7.

The question asks us for the sum of these components:

Remember that the "shifts" involved with circular functions are sort of like those found in parabolas. When you shift a parabola left or right, you have to think "oppositely". A right shift requires you to subtract from the x-component, and a left one requires you to add. Hence, this circle has a negative 5 horizontal shift, and a negative 22 vertical shift.

You can also remember the general formula for a circle with center at  and a radius of .

Comparing this to the given equation, we can determine the radius and center point.

The center point is at  and the circle has a radius of 11.

The question asks us for the sum of these components: