All Advanced Geometry Resources
Example Questions
Example Question #2 : Graphing
The figure above shows the graph of y = f(x). Which of the following is the graph of y = |f(x)|?
One of the properties of taking an absolute value of a function is that the values are all made positive. The values themselves do not change; only their signs do. In this graph, none of the y-values are negative, so none of them would change. Thus the two graphs should be identical.
Example Question #3 : Graphing
Below is the graph of the function 
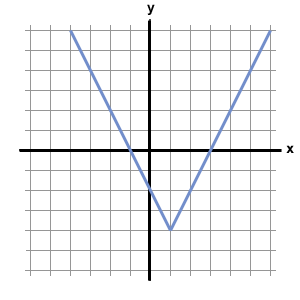
Which of the following could be the equation for 
First, because the graph consists of pieces that are straight lines, the function must include an absolute value, whose functions usually have a distinctive "V" shape. Thus, we can eliminate f(x) = x2 – 4x + 3 from our choices. Furthermore, functions with x2 terms are curved parabolas, and do not have straight line segments. This means that f(x) = |x2 – 4x| – 3 is not the correct choice.
Next, let's examine f(x) = |2x – 6|. Because this function consists of an abolute value by itself, its graph will not have any negative values. An absolute value by itself will only yield non-negative numbers. Therefore, because the graph dips below the x-axis (which means f(x) has negative values), f(x) = |2x – 6| cannot be the correct answer.
Next, we can analyze f(x) = |x – 1| – 2. Let's allow x to equal 1 and see what value we would obtain from f(1).
f(1) = | 1 – 1 | – 2 = 0 – 2 = –2
However, the graph above shows that f(1) = –4. As a result, f(x) = |x – 1| – 2 cannot be the correct equation for the function.
By process of elimination, the answer must be f(x) = |2x – 2| – 4. We can verify this by plugging in several values of x into this equation. For example f(1) = |2 – 2| – 4 = –4, which corresponds to the point (1, –4) on the graph above. Likewise, if we plug 3 or –1 into the equation f(x) = |2x – 2| – 4, we obtain zero, meaning that the graph should cross the x-axis at 3 and –1. According to the graph above, this is exactly what happens.
The answer is f(x) = |2x – 2| – 4.
Example Question #1 : Graphing
Which of the following could be a value of 

The graph is a down-opening parabola with a maximum of 
Example Question #5 : How To Graph A Function

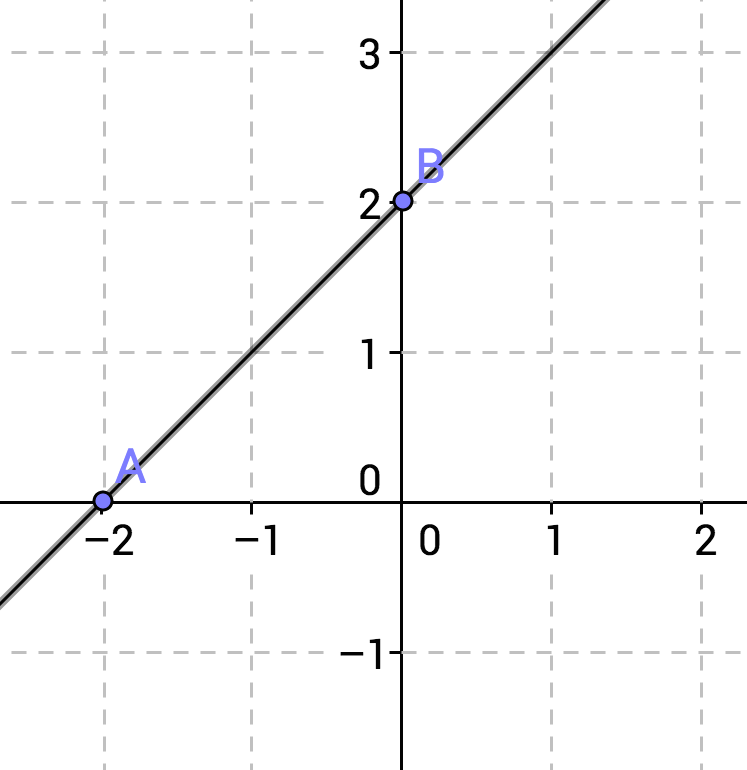
What is the equation for the line pictured above?
A line has the equation




The 


The slope can be found by selecting two points, for example, the y-intercept and the next point over that crosses an even point, for example, 
Now applying the slope formula,
which yields 
Therefore the equation of the line becomes:
Example Question #6 : How To Graph A Function
Which of the following graphs represents the y-intercept of this function?





Graphically, the y-intercept is the point at which the graph touches the y-axis. Algebraically, it is the value of 

Here, we are given the function 


So the y-intercept is at 
Example Question #2 : Graphing
Which of the following graphs represents the x-intercept of this function?





Graphically, the x-intercept is the point at which the graph touches the x-axis. Algebraically, it is the value of 

Here, we are given the function 


So the x-intercept is at 
Example Question #7 : How To Graph A Function
Which of the following represents 




A line is defined by any two points on the line. It is frequently simplest to calculate two points by substituting zero for x and solving for y, and by substituting zero for y and solving for x.
Let 
So our first set of points (which is also the y-intercept) is
Let 
So our second set of points (which is also the x-intercept) is 
Example Question #1 : How To Graph A Function
Suppose 
To obtain the graph of 


To the left
To the right
Upwards
Up and right
Downwards
Upwards
There are four shifts of the graph y = f(x):
y = f(x) + c shifts the graph c units upwards.
y = f(x) – c shifts the graph c units downwards.
y = f(x + c) shifts the graph c units to the left.
y = f(x – c) shifts the graph c units to the right.
Example Question #11 : How To Graph A Function
Which graph accurately represents the following function:






The first step in determining which graph is correct is finding the origin of the function. If both x and y are equal to 0, the coordinates of the origin would be 

Example Question #12 : How To Graph A Function
Which of the following graphs represents
Which of the following graphs represents the function 






The easiest way to determine which graph belongs to the equation is to find the x-intercept, the slope, and if necessary the y-intercept as well.
This equation is written in 
Therefore we know that the slope equals 

The x-intercept can be found by substituting 0 for y and solving the equation.
The x-intercept is 
The only graph that meets these standards is

Certified Tutor
Certified Tutor
All Advanced Geometry Resources








































