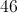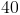All ACT Math Resources
Example Questions
Example Question #11 : Trigonometry
Josh is at the state fair when he decides to take a helicopter ride. He looks down at about a 35 ° angle of depression and sees his house. If the helicopter was about 250 ft above the ground, how far does the helicopter have to travel to be directly above his house?
sin 35 ° = 0.57 cos 35 ° = 0.82 tan 35 ° = 0.70
304.88 ft
142.50 ft
357.14 ft
438.96 ft
205.00 ft
357.14 ft
The angle of depression is the angle formed by a horizontal line and the line of sight looking down from the horizontal.
This is a right triangle trig problem. The vertical distance is 250 ft and the horizontal distance is unknown. The angle of depression is 35°. We have an angle and two legs, so we use tan Θ = opposite ÷ adjacent. This gives an equation of tan 35° = 250/d where d is the unknown distance to be directly over the house.
Example Question #12 : Trigonometry
Consider the triangle  where
where 

Note: The triangle is not necessarily to scale
None of the other answers
To solve this equation, it is best to remember the mnemonic SOHCAHTOA which translates to Sin = Opposite / Hypotenuse, Cosine = Adjacent / Hypotenuse, and Tangent = Opposite / Adjacent. Looking at the problem statement, we are given an angle and the side opposite of the angle, and we are looking for the side adjacent to the angle. Therefore, we will be using the TOA part of the mnemonic. Inserting the values given in the problem statement, we can write 

Example Question #4 : How To Find A Missing Side With Tangent
A piece of wire is tethered to a 

Begin by drawing out this scenario using a little right triangle:

Note importantly: We are looking for 
Using your calculator, solve for 
This is 
Thus, rounded, your answer is 

Example Question #5 : How To Find A Missing Side With Tangent

What is the value of 
Recall that the tangent of an angle is the ratio of the opposite side to the adjacent side of that triangle. Thus, for this triangle, we can say:
Solving for 

Example Question #6 : How To Find A Missing Side With Tangent

In the right triangle shown above, let 




First we need to find the value of 

Now we see at point 


Thus we get that

and plugging in our values and reducing yields:
Example Question #7 : How To Find A Missing Side With Tangent
In a given right triangle 




In right triangles, SOHCAHTOA tells us that 




Thus, 
Example Question #12 : Trigonometry
In a given right triangle 




In right triangles, SOHCAHTOA tells us that 




Thus, 
Example Question #9 : How To Find A Missing Side With Tangent
In a given right triangle 




In right triangles, SOHCAHTOA tells us that 




Thus, 
Example Question #13 : Trigonometry
What is the perimeter of the following figure?
The question asks for you to find the perimeter of the given figure. The figure has twelve sides total, of two varying lengths. One length is given to you, 4. The other length must be solved for using either the sine or tangent functions. However, one can arrive to answer more quickly by recognizing that the drawn triangle is actually a 3-4-5 triangle, where 3, 4, and 5 corresponds to each of the sides of the triangle. This is a pythagorean triple and this ratio should be easily remembered.
Thus if 3 is the missing side, and there are eight sides of length 3 and four sides of length 4, one can arrive to the answer:
Example Question #14 : Trigonometry
A man is setting up a laser on the ground, angling it toward the very top of a flag pole. If the flag pole is 

You can draw out your scenario like a triangle:

Now, you know that this means:
Using your calculator, you can utilize the inverse 
This rounds to 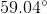
Certified Tutor
All ACT Math Resources