All Trigonometry Resources
Example Questions
Example Question #7 : Solving Word Problems With Trigonometry
From the top of a lighthouse that sits 105 meters above the sea, the angle of depression of a boat is 19o. How far from the boat is the top of the lighthouse?
110.53 meters
423.18 meters
318.18 meters
36.15 meters
318.18 meters
To solve this problem, we need to create a diagram, but in order to create that diagram, we need to understand the vocabulary that is being used in this question. The following diagram clarifies the difference between an angle of depression (an angle that looks downward; relevant to our problem) and the angle of elevation (an angle that looks upward; relevant to other problems, but not this specific one.) Imagine that the top of the blue altitude line is the top of the lighthouse, the green line labelled GroundHorizon is sea level, and point B is where the boat is.
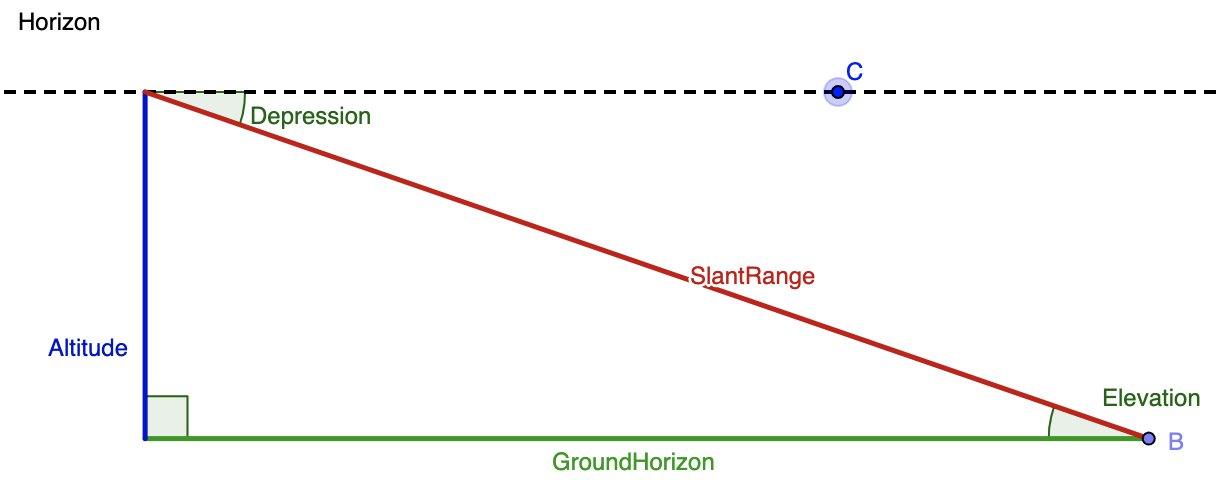
Merging together the given info and this diagram, we know that the angle of depression is 19o and and the altitude (blue line) is 105 meters. While the blue line is drawn on the left hand side in the diagram, we can assume is it is the same as the right hand side. Next, we need to think of the trig function that relates the given angle, the given side, and the side we want to solve for. The altitude or blue line is opposite the known angle, and we want to find the distance between the boat (point B) and the top of the lighthouse. That means that we want to determine the length of the hypotenuse, or red line labelled SlantRange. The sine function relates opposite and hypotenuse, so we'll use that here. We get:




Example Question #8 : Solving Word Problems With Trigonometry
Angelina just got a new car, and she wants to ride it to the top of a mountain and visit a lookout point. If she drives 4000 meters along a road that is inclined 22o to the horizontal, how high above her starting point is she when she arrives at the lookout?
1616.1 meters
3708.74 meters
1480 meters
10677.87 meters
9.37 meters
1480 meters
As with other trig problems, begin with a sketch of a diagram of the given and sought after information.

Angelina and her car start at the bottom left of the diagram. The road she is driving on is the hypotenuse of our triangle, and the angle of the road relative to flat ground is 22o. Because we want to find the change in height (also called elevation), we want to determine the difference between her ending and starting heights, which is labelled x in the diagram. Next, consider which trig function relates together an angle and the sides opposite and hypotenuse relative to it; the correct one is sine. Then, set up:


Therefore the change in height between Angelina's starting and ending points is 1480 meters.
Example Question #9 : Solving Word Problems With Trigonometry
Two buildings with flat roofs are 50 feet apart. The shorter building is 40 feet tall. From the roof of the shorter building, the angle of elevation to the edge of the taller building is 48o. How high is the taller building?
73.5 feet
77.2 feet
95.5 feet
40 feet
55.5 feet
95.5 feet
To solve this problem, let's start by drawing a diagram of the two buildings, the distance in between them, and the angle between the tops of the two buildings. Then, label in the given lengths and angle.
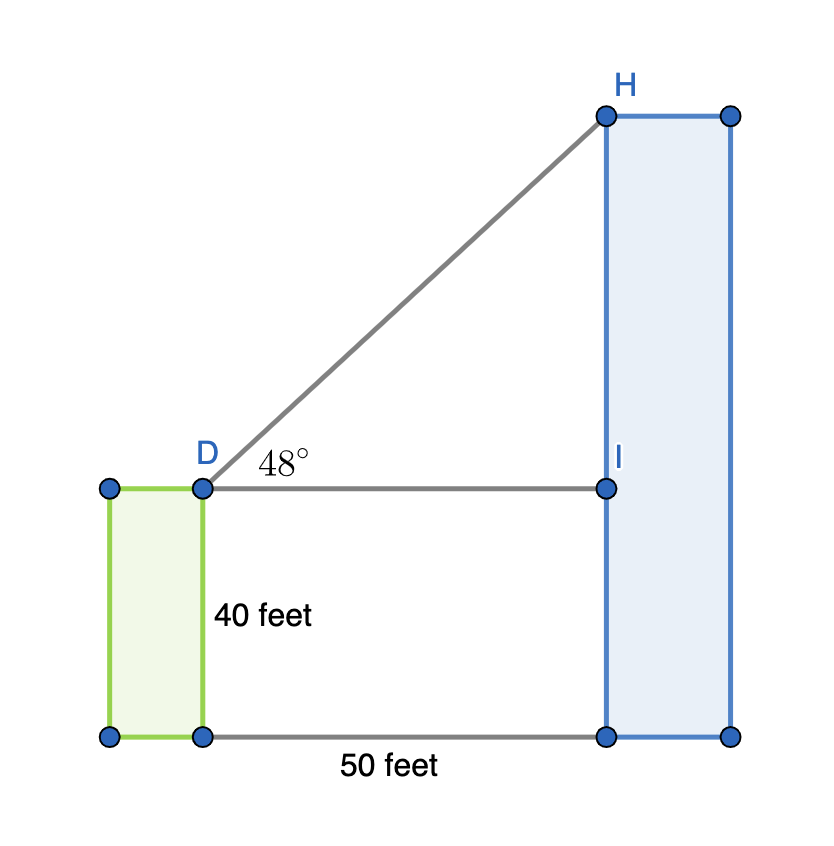
We are being asked to find the height of the taller building, but this diagram does not provide a triangle that has as one of its sides the entire height of the larger (rightmost and blue) building. However, we can instead find the distance 

Remember that this is not the full height of the larger building. To find that, we need to add 
Example Question #1 : Solving Word Problems With Trigonometry
Two buildings with flat roofs are 80 feet apart. The shorter building is 55 feet tall. From the roof of the shorter building, the angle of elevation to the edge of the taller building is 32o. How high is the taller building?
49.6 feet
129.6 feet
122.8 feet
97.4 feet
104.6 feet
104.6 feet
To solve this problem, let's start by drawing a diagram of the two buildings, the distance in between them, and the angle between the tops of the two buildings. Then, label in the given lengths and angle.
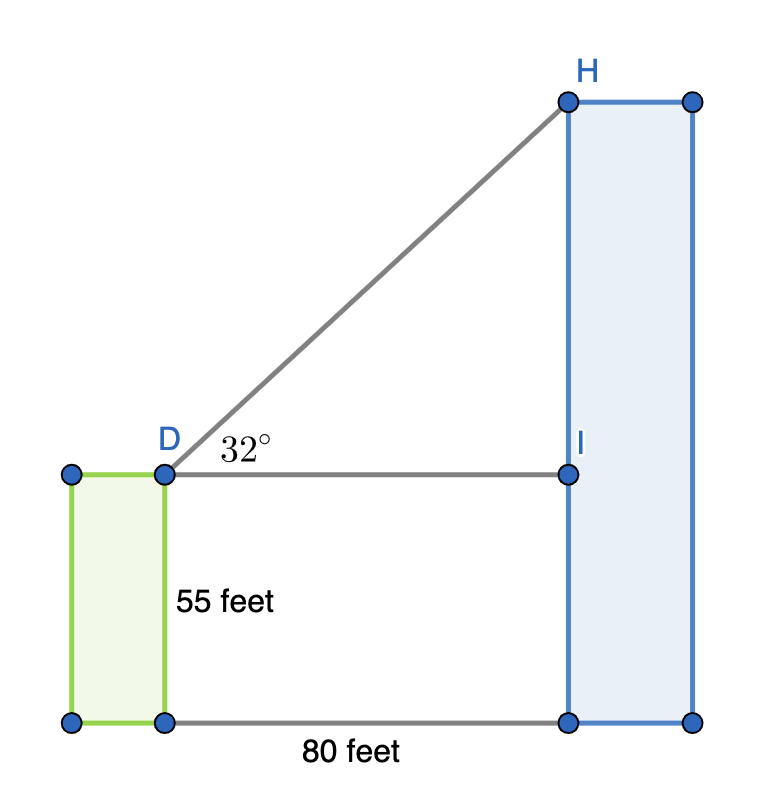
We are being asked to find the height of the taller building, but this diagram does not provide a triangle that has as one of its sides the entire height of the larger (rightmost and blue) building. However, we can instead find the distance 

Remember that this is not the full height of the larger building. To find that, we need to add 
Example Question #31 : Right Triangles
Two buildings with flat roofs are 330 feet apart. The shorter building is 31.4 feet tall. From the roof of the shorter building, the angle of elevation to the edge of the taller building is 61.4o. How high is the taller building?
933.9 feet
189.4 feet
603.9 feet
321.13 feet
635.3 feet
635.3 feet
To solve this problem, let's start by drawing a diagram of the two buildings, the distance in between them, and the angle between the tops of the two buildings. Then, label in the given lengths and angle.

We are being asked to find the height of the taller building, but this diagram does not provide a triangle that has as one of its sides the entire height of the larger (rightmost and blue) building. However, we can instead find the distance 

Remember that this is not the full height of the larger building. To find that, we need to add 
Example Question #11 : Solving Word Problems With Trigonometry
You need to build a diagonal support for the bleachers at the local sportsfield. The support needs to reach from the ground to the top of the bleacher. How the support should look is highlighted in blue below. The bleacher wall is 10 feet high and perpendicular to the ground. The owner would like the support to only stick out 3 feet from the bleacher at the bottom. What is the length of the support you need to build?

10.44 ft
109 ft
11.32 ft
20 ft
10.44 ft
It is important to recognize that the bleacher, the ground, and the support form a right triangle with the right angle formed by the intersection of the bleacher wall and the ground. We know the bottom of the support should only be 3ft from the bleacher wall on the ground and the bleacher wall is 10ft high. We will use the Pythagorean Theorem to solve for the length of the support, which is the hypotenuse of this right triangle. Our base of the triangle is 3 feet and the leg is 10 feet.
And so we need a support of 10.44 feet long.
Example Question #11 : Solving Word Problems With Trigonometry
An airplane takes off from point 

31,000 feet
35,601 feet
37,844 feet
38,000 feet
37,844 feet
We begin by drawing a picture. Let 

Now we will use our knowledge of right triangles. We know the trigonometric identity, 

And so the distance from point 
Example Question #12 : Solving Word Problems With Trigonometry
You are reading a billboard perpendicular to the ground walking downtown. You are standing about 500 ft from the base of the billboard and looking up at an angle of 67 degrees. About what height is the part of the billboard you are looking at standing at? Round to the nearest whole number.
1,178 feet
500 feet
5,325 feet
2,367 feet
1,178 feet
We begin by drawing a picture. Let point be where your eyes are looking on the billboard and point
be where you are standing on the ground.
We must solve for the height of the billboard. We have information of the adjacent side of the right triangle formed and we wish to know the height, which will be the opposite side from you. Using trigonometric identities, we will use . We can plug in 500 ft for our adjacent side and 67 degrees for our
.
And the height of the section of the billboard you are looking at is 1,178 ft above the ground.
Example Question #11 : Solving Word Problems With Trigonometry
You have a shadow that measures 66cm from your feet. You are 60cm tall. What is the sun’s angle of elevation? Round to the nearest degree.
The important thing to note for this problem is that we are working with similar triangles. Your shadow forms a right triangle with its three points being your feet, the top of your head, and the top of your shadow’s head. We have enough information to find the angle of your head’s elevation to your shadow on the ground. Just like your shadow forms a right triangle to your body, a similar right triangle will also be formed to the sun’s altitude. We know that this triangle is similar because it shares the angle opposite your body and it shares a right angle, so it’s third angle must also be equal. Since it shares the angle opposite your body, we must only solve for the angle of your head’s elevation and it will be the same as the sun’s elevation.
We do this by using . We can plug in 60cm for the opposite since that is your height, and 66cm for the adjacent since that is the length of the shadow. We are solving for
.
And so the sun is at an elevation of 48 degrees.
Example Question #1 : Use Special Triangles To Make Deductions
Which of the following is true about the right triangle below?

Since the pictured triangle is a right triangle, the unlabeled angle at the lower left is a right angle measuring 90 degrees. Since interior angles in a triangle sum to 180 degrees, the unlabeled angle at the upper left can be calculated by 180 - 60 - 90 = 30. The pictured triangle is therefore a 30-60-90 triangle. In a 30-60-90 triangle, the ratio between the hypotenuse and the shortest side length is 2:1. Therefore, C = 2A.
Certified Tutor
Certified Tutor
All Trigonometry Resources





































