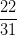Call Now to Set Up Tutoring:

(888) 888-0446

SSAT Upper Level Math : SSAT Upper Level Quantitative (Math)

Study concepts, example questions & explanations for SSAT Upper Level Math

Create An Account

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #21 : Lines

A line has the equation $\displaystyle 8x-7y=14$ . What is the slope of the line?

Possible Answers:

$-\frac{7}{8}$ $\displaystyle -\frac{7}{8}$

$\frac{8}{7}$ $\displaystyle \frac{8}{7}$

$\frac{7}{8}$ $\displaystyle \frac{7}{8}$

$-\frac{8}{7}$ $\displaystyle -\frac{8}{7}$

Correct answer:

$\frac{8}{7}$ $\displaystyle \frac{8}{7}$

Explanation:

Change the equation into the more familiar y=mx+b $\displaystyle y=mx+b$ form. The value of $\displaystyle m$ will be the slope.

$\displaystyle 8x-7y=14$

$\displaystyle 7y=8x-14$

$y=\frac{8}{7}x-2$ $\displaystyle y=\frac{8}{7}x-2$

Report an Error

Example Question #2 : How To Find Slope Of A Line

What is the slope of a line that passes through the points $(15, -2)\text{ and }(-16, 20)$ $\displaystyle (15, -2)\text{ and }(-16, 20)$ ?

Possible Answers:

$-\frac{22}{31}$ $\displaystyle -\frac{22}{31}$

$-\frac{31}{22}$ $\displaystyle -\frac{31}{22}$

$\frac{31}{22}$ $\displaystyle \frac{31}{22}$

$\frac{22}{31}$ $\displaystyle \frac{22}{31}$

Correct answer:

$-\frac{22}{31}$ $\displaystyle -\frac{22}{31}$

Explanation:

Use the following formula to find the slope:

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}$ $\displaystyle \text{Slope}=\frac{y_2-y_1}{x_2-x_1}$

Plug in the given points to find the slope.

$Slope=\frac{20-(-2)}{-16-15}=-\frac{22}{31}$ $\displaystyle Slope=\frac{20-(-2)}{-16-15}=-\frac{22}{31}$

Report an Error

Example Question #3 : How To Find Slope Of A Line

Find the slope of the line that passes through the points $(-9, 2)\text{ and }(-2, -2)$ $\displaystyle (-9, 2)\text{ and }(-2, -2)$

Possible Answers:

$\frac{7}{4}$ $\displaystyle \frac{7}{4}$

$\frac{4}{7}$ $\displaystyle \frac{4}{7}$

$-\frac{4}{7}$ $\displaystyle -\frac{4}{7}$

$-\frac{7}{4}$ $\displaystyle -\frac{7}{4}$

Correct answer:

$-\frac{4}{7}$ $\displaystyle -\frac{4}{7}$

Explanation:

Use the following formula to find the slope:

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}$ $\displaystyle \text{Slope}=\frac{y_2-y_1}{x_2-x_1}$

$\text{Slope}=\frac{-2-2}{-2-(-9)}=-\frac{4}{7}$ $\displaystyle \text{Slope}=\frac{-2-2}{-2-(-9)}=-\frac{4}{7}$

Report an Error

Example Question #4 : How To Find Slope Of A Line

Find the slope of the line that passes through the points $(-6, 2)\text{ and }(4,-4)$ $\displaystyle (-6, 2)\text{ and }(4,-4)$

Possible Answers:

$\displaystyle 5$

$-\frac{3}{5}$ $\displaystyle -\frac{3}{5}$

$\displaystyle -5$

$\frac{3}{5}$ $\displaystyle \frac{3}{5}$

Correct answer:

$-\frac{3}{5}$ $\displaystyle -\frac{3}{5}$

Explanation:

Use the following formula to find the slope:

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}$ $\displaystyle \text{Slope}=\frac{y_2-y_1}{x_2-x_1}$

$\text{Slope}=\frac{-4-2}{4-(-6)}=\frac{-6}{10}=-\frac{3}{5}$ $\displaystyle \text{Slope}=\frac{-4-2}{4-(-6)}=\frac{-6}{10}=-\frac{3}{5}$

Report an Error

Example Question #5 : How To Find Slope Of A Line

Find the slope of the line that passes through the points $(1,1)\text{ and }(-8,6)$ $\displaystyle (1,1)\text{ and }(-8,6)$ .

Possible Answers:

$\frac{9}{5}$ $\displaystyle \frac{9}{5}$

$-\frac{9}{5}$ $\displaystyle -\frac{9}{5}$

$-\frac{5}{9}$ $\displaystyle -\frac{5}{9}$

$\frac{5}{9}$ $\displaystyle \frac{5}{9}$

Correct answer:

$-\frac{5}{9}$ $\displaystyle -\frac{5}{9}$

Explanation:

Use the following formula to find the slope:

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}$ $\displaystyle \text{Slope}=\frac{y_2-y_1}{x_2-x_1}$

$\text{Slope}=\frac{6-1}{-8-1}=-\frac{5}{9}$ $\displaystyle \text{Slope}=\frac{6-1}{-8-1}=-\frac{5}{9}$

Report an Error

Example Question #6 : How To Find Slope Of A Line

Find the slope of the line that passes through the points $(2, -3)\text{ and }(-3, -3)$ $\displaystyle (2, -3)\text{ and }(-3, -3)$

Possible Answers:

$\frac{5}{6}$ $\displaystyle \frac{5}{6}$

$\displaystyle 0$

$\frac{6}{5}$ $\displaystyle \frac{6}{5}$

$\text{Undefined}$ $\displaystyle \text{Undefined}$

Correct answer:

$\displaystyle 0$

Explanation:

Use the following formula to find the slope:

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}$ $\displaystyle \text{Slope}=\frac{y_2-y_1}{x_2-x_1}$

$\text{Slope}=\frac{-3-(-3)}{-3-2}=\frac{0}{-5}=0$ $\displaystyle \text{Slope}=\frac{-3-(-3)}{-3-2}=\frac{0}{-5}=0$

Report an Error

Example Question #302 : Ssat Upper Level Quantitative (Math)

Find the slope of the line that passes through the points $\left(\frac{1}{3}, -\frac{4}{5}\right)$ $\displaystyle \left(\frac{1}{3}, -\frac{4}{5}\right)$ and $\left ( -6,10\right )$ $\displaystyle \left ( -6,10\right )$ .

Possible Answers:

$\frac{162}{95}$ $\displaystyle \frac{162}{95}$

$-\frac{95}{162}$ $\displaystyle -\frac{95}{162}$

$\frac{95}{162}$ $\displaystyle \frac{95}{162}$

$-\frac{162}{95}$ $\displaystyle -\frac{162}{95}$

Correct answer:

$-\frac{162}{95}$ $\displaystyle -\frac{162}{95}$

Explanation:

Use the following formula to find the slope:

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}$ $\displaystyle \text{Slope}=\frac{y_2-y_1}{x_2-x_1}$

Plug in the given points to find the slope.

$\text{Slope}=\frac{10-(-\frac{4}{5})}{-6-\frac{1}{3}}=\frac{\frac{54}{5}}{-\frac{19}{3}}=-\frac{162}{95}$ $\displaystyle \text{Slope}=\frac{10-(-\frac{4}{5})}{-6-\frac{1}{3}}=\frac{\frac{54}{5}}{-\frac{19}{3}}=-\frac{162}{95}$

Report an Error

Example Question #7 : How To Find Slope Of A Line

A line goes passes through the points $\left(-\frac{1}{2}, 5\right)\text{ and }\left(\frac{3}{2}, -1\right)$ $\displaystyle \left(-\frac{1}{2}, 5\right)\text{ and }\left(\frac{3}{2}, -1\right)$ . What is the slope of this line?

Possible Answers:

$\displaystyle 3$

$\displaystyle -3$

$-\frac{1}{2}$ $\displaystyle -\frac{1}{2}$

$\frac{1}{2}$ $\displaystyle \frac{1}{2}$

Correct answer:

$\displaystyle -3$

Explanation:

Use the following formula to find the slope of a line:

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}$ $\displaystyle \text{Slope}=\frac{y_2-y_1}{x_2-x_1}$

The slope of this line would be

$\frac{-1-5}{\frac{3}{2}-(-\frac{1}{2})}=\frac{-6}{2}=-3$ $\displaystyle \frac{-1-5}{\frac{3}{2}-(-\frac{1}{2})}=\frac{-6}{2}=-3$

Report an Error

Example Question #83 : Coordinate Plane

What is the slope of line 3 = 8y - 4x?

Possible Answers:

-2

-0.5

0.5

Correct answer:

0.5

Explanation:

Solve equation for y. y=mx+b, where m is the slope

Report an Error

Example Question #3 : How To Find The Slope Of A Line

Find the slope of the line 6X – 2Y = 14

Possible Answers:

-3

-6

Correct answer:

Explanation:

Put the equation in slope-intercept form:

y = mx + b

-2y = -6x +14

y = 3x – 7

The slope of the line is represented by M; therefore the slope of the line is 3.

Report an Error

View SSAT- Upper Level Tutors

Beverly
Certified Tutor

Wesleyan University, Bachelors, Mathematics. The University of Texas at Arlington, Masters, Linguistics.

View SSAT- Upper Level Tutors

Jessica
Certified Tutor

Flagler College-St Augustine, Bachelor of Education, Education of the Deaf and Hearing Impaired.

View SSAT- Upper Level Tutors

Danielle
Certified Tutor

Northwestern University, Bachelors, Journalism. Tulane University of Louisiana, Master of Science, Finance.

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Biology Tutors in Chicago, Calculus Tutors in Atlanta, Physics Tutors in Phoenix, MCAT Tutors in Atlanta, Algebra Tutors in Houston, MCAT Tutors in Boston, Spanish Tutors in Boston, English Tutors in Washington DC, GMAT Tutors in Seattle, English Tutors in Denver

Popular Courses & Classes

ISEE Courses & Classes in New York City, SAT Courses & Classes in Philadelphia, SSAT Courses & Classes in Dallas Fort Worth, MCAT Courses & Classes in Phoenix, GRE Courses & Classes in Los Angeles, ACT Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in Miami, Spanish Courses & Classes in Boston, SAT Courses & Classes in Miami, Spanish Courses & Classes in New York City

Popular Test Prep

SAT Test Prep in Seattle, LSAT Test Prep in Denver, GMAT Test Prep in Denver, GMAT Test Prep in Chicago, SSAT Test Prep in Philadelphia, GMAT Test Prep in Miami, GRE Test Prep in Philadelphia, SAT Test Prep in Denver, ISEE Test Prep in San Diego, MCAT Test Prep in Dallas Fort Worth

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SSAT Upper Level Math : SSAT Upper Level Quantitative (Math)

Study concepts, example questions & explanations for SSAT Upper Level Math

All SSAT Upper Level Math Resources

Example Questions

Example Question #21 : Lines

Example Question #2 : How To Find Slope Of A Line

Example Question #3 : How To Find Slope Of A Line

Example Question #4 : How To Find Slope Of A Line

Example Question #5 : How To Find Slope Of A Line

Example Question #6 : How To Find Slope Of A Line

Example Question #302 : Ssat Upper Level Quantitative (Math)

Example Question #7 : How To Find Slope Of A Line

Example Question #83 : Coordinate Plane

Example Question #3 : How To Find The Slope Of A Line

All SSAT Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: