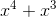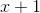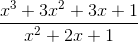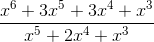Always look to reduce fractions. The  will cancel out from the denominator of left fraction with numerator of right fraction.

This leads to 

.

Now, multiply numerator with numerator and denominator with denominator.

Remember the distribute the  in the denominator which leads you to the answer.

Take care of the exponents first before solving the problem.

The numerator should be  and denominator is .

Then the  should cancel out leaving you with the right answer. 

Don't try to multiply everything out. Try to factor all the quadratics out into simple binomials. Remember, we need two numbers who are factors of the c term must add up the value of the b term.

With that, the fraction now looks like:

.

The , , and  all cancel leaving you with  as the final answer.

First, let's take care of the exponents. When dividing exponents of the same base, all you have to do is take the exponent from the numerator and subtract it with the denominator. Now we have  leftover in the numerator.

Let's take a look at the denominator of the left fraction. It may not be obvious but if you factor out , we are left with an expression: . We have a  in the numerator so now they can cancel out.

Lastly, lets focus on the quadratic in the denominator. Does it look familiar? It is . The numerator in the right fraction has .

Same rule applies with dividing exponents.

This should leave you with just  as the final answer.

There are two methods in solving this problem. 

Method 1:

Try to simplify the problem as  best as you can before you cross-multiply. Remember when breaking down the quadratic, find two terms which are factors of the c term that are also the sum of the b term. The equation should be:

.

The  cancel out and now we can cross-multiply. This new equation is now . Distribute the  and have like-terms on one side of the equation and the rest on the otherside of the equation. Answer should be . 

Method 2 (Not preferred):

If you don't know how to simplify the equation, that's ok. Lets just cross-multiply and create a quadratic equation. 

We can plug into quadratic equation , or factor. Regardless, we should have: . 

Solve individually:

WAIT, how come answer isn't Both  and . The reason this method is not preferred is because many people forget we need to check if these answers are valid with the original question. We will use the factored equation from method 1:   . Remember, if at any point a denominator is , the fraction is undefined. From the denominator, if  was either  or  ( which are the roots to that quadratic), the fraction is undefined. Since we have an answer of  and that's one of the values that makes the fraction undefined, we cross that out as a possible answer which leaves only  as the only possible answer. If you plug in  back into the question we get:

This answer is valid and correct.

Don't try to multiply both numerators and denominators and cross-multiply. It will be time-consuming and too much work. Instead, lets try to simplify. Lets factor the denominator of the left fraction by taking out a . We now have:

The and  cancel out leaving a  behind. If you don't believe it, just plug in any value for  say . One answer gives you , another  The relationship between these values is ratio is . With that simplification, lets cross-multiply:

Divide both sides by : 

Take the square root of both sides and remember when doing that, you need to account for the negative value of the root. This should lead you to two possible answers. There is no answer of  HOWEVER, to get the right answer, break the radical into:

 and multiply top and bottom by  to get the right answer, .

First, lets factor and reduce some terms. Remember, we need to find two terms that are factors of the c term that add up to the b term. This should look like this:

Remember, order of operations is crucial. *PEMDAS* Multiplication has priority over addition. So when cancelling, we should only have:

This should be simple with both fractions having the same denominator. We now have:

. Then cross-multiply.

 Then subtract  on both sides to get the answer. 

Make sure this value doesn't violate any undefined fraction. As you check, this answer is still good and is the right answer.

Lets just cross multiply and then square both sides.

 Creates the quadratic equation.

 Now factor out .

Set up two different equations.

These are the correct answers and don't violate the radical. 

Lets factor as this is an easier approach especially with the absolute value present. Remember, we need to find two terms that are factors of the c term that add up to the b term. We get:

The  cancels and now with the absolute vaue present, we need to split into two different equations. Remember, absolute value means there is a positive value and a negative value. So for the first equation:

Now the second equation:

Double check that these answers don't create undefined answers. In this case, it doesn't so  and  are the answers to this question. 

Multiply the numerator and denominator.   

Rewrite the complex fraction using a division sign.