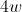SSAT Middle Level Math : Quadrilaterals
Study concepts, example questions & explanations for SSAT Middle Level Math
All SSAT Middle Level Math Resources
Example Questions
Example Question #54 : Plane Geometry
What is the total area of the surface of the cube shown in the above diagram?
A cube comprises six faces, each of which is a square. To find its total surface area, find the area of one face by squaring its sidelength:
Then multiply this by six:
Example Question #21 : Quadrilaterals
What is the total area of the surface of the cube shown in the above diagram?
A cube comprises six faces, each of which is a square. To find its total surface area, find the area of one face by squaring its sidelength:
Then multiply this by six:
Example Question #4 : How To Find The Area Of A Square
What is the total area of the surface of the cube shown in the above diagram?
A cube comprises six faces, each of which is a square. To find its total surface area, find the area of one face by squaring its sidelength:
Then multiply this by six:
Example Question #5 : Geometry
A square is 9 feet long on each side. How many smaller squares, each 3 feet on a side can be cut out of the larger square?
Each side can be divided into three 3-foot sections. This gives a total of 
Example Question #6 : How To Find The Area Of A Square
Order the following from least area to greatest area:
Figure A: A square with sides of length 3 feet each.
Figure B: A rectangle with length 30 inches and width 42 inches.
Figure C: A rectangle with length 2 feet and width 4 feet.
Figure A has area 
Figure B has dimensions 
Figure C has area 
From least area to greatest, the figures rank C, B, A.
Example Question #7 : How To Find The Area Of A Square
The length of one side of a square is 
The area of any quadrilateral is found by multiplying the length by the width. Because a square has four equal sides, the length and width are the same. For the square in this question, the length and width are 
Remember: area is always given in units2 .
Example Question #1 : How To Find The Area Of A Square
If a square has a side that is 3 yards long, what is the area in square feet?
The area of a square is found by multiplying the length of a side by itself.
If one side is 3 yards, this means one side is 9 feet since there are 3 feet in a yard.
Since every side is of equal length, you would multiply 9 feet by 9 feet to find the area.
This results in 81 square feet, which is the correct answer.
Example Question #63 : Plane Geometry
Note: Figure NOT drawn to scale.
Refer to the above diagram, which shows a square. Give the ratio of the area of the yellow region to that of the white region.
The correct answer is not given among the other choices.
The area of the entire square is the square of the length of a side, or
The area of the right triangle is half the product of its legs, or
The area of the yellow region is therefore the difference of the two, or
The ratio of the area of the yellow region to that of the white region is
Example Question #11 : How To Find The Area Of A Square
Find the area of a square with a width of 13cm.
To find the area of a square, we will use the following formula:
where l is the length and w is the width of the square.
Now, we know the width of the square is 13cm. Because it is a square, all sides are equal. Therefore, the length is also 13cm.
So, we can substitute. We get
Example Question #291 : Ssat Middle Level Quantitative (Math)
The width of a rectangle is half of its length. If the width is given as 
The sum of the widths is 
Certified Tutor
Certified Tutor
All SSAT Middle Level Math Resources
