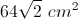We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

SAT Math : How to find the area of a square

Study concepts, example questions & explanations for SAT Math

Create An Account

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Find The Area Of A Square

ABCD and EFGH are squares such that the perimeter of ABCD is 3 times that of EFGH. If the area of EFGH is 25, what is the area of ABCD?

Possible Answers:

225

Correct answer:

225

Explanation:

Assign variables such that

One side of ABCD = a

and One side of EFGH = e

Note that all sides are the same in a square. Since the perimeter is the sum of all sides, according to the question:

4a = 3 x 4e = 12e or a = 3e

From that area of EFGH is 25,

e x e = 25 so e = 5

Substitute a = 3e so a = 15

We aren’t done. Since we were asked for the area of ABCD, this is a x a = 225.

Report an Error

Example Question #2 : How To Find The Area Of A Square

A square has an area of 36. If all sides are doubled in value, what is the new area?

Possible Answers:

132

108

144

Correct answer:

144

Explanation:

Let S be the original side length. S*S would represent the original area. Doubling the side length would give you 2S*2S, simplifying to 4*(S*S), giving a new area of 4x the original, or 144.

Report an Error

Example Question #3 : How To Find The Area Of A Square

Freddie is building a square pen for his pig. He plans to buy x feet of fencing to build the pen. This will result in a pen with an area of p square feet. Unfortunately, he only has enough money to buy one third of the planned amount of fencing. Which expression represents the area of the pen he can build with this limited amount of fencing?

Possible Answers:

p/9

p/3

p/6

Correct answer:

p/9

Explanation:

If Freddie uses x feet of fencing makes a square, each side must be x/4 feet long. The area of this square is (x/4)² = x²/16 = p square feet.

If Freddie uses one third of x feet of fencing makes a square, each side must be x/12 feet long. The area of this square is (x/12)² = x²/144 = 1/9(x²/16) = 1/9(p) = p/9 square feet.

Alternate method:

The scale factor between the small perimeter and the larger perimeter = 1 : 3. Since we're comparing area, a two-dimensional measurement, we can square the scale factor and see that the ratio of the areas is 1²: 3² = 1 : 9.

Report an Error

Example Question #4 : How To Find The Area Of A Square

If the diagonal of a square measures $16\sqrt{2} \ cm$ , what is the area of the square?

Possible Answers:

$64\sqrt{2}\ cm^{2}$

$512\ cm^{2}$

$128\ cm^{2}$

$256\ cm^{2}$

$32\sqrt{2}\ cm^{2}$

Correct answer:

$256\ cm^{2}$

Explanation:

This is an isosceles right triangle, so the diagonal must equal $\sqrt{2}$ times the length of a side. Thus, one side of the square measures $16\ cm$ , and the area is equal to $(16 \ cm)^{2} = 256\ cm^{2}$

Report an Error

Example Question #12 : Quadrilaterals

A square $A$ has side lengths of $z$ . A second square $B$ has side lengths of $2.25z$ . How many $A's$ can you fit in a single $B$ ?

Possible Answers:

$4$

$1$

$5.06$

$2.25$

$3$

Correct answer:

$5.06$

Explanation:

The area of $A$ is $n$ , the area of $B$ is $5.0625n$ . Therefore, you can fit 5.06 $A's$ in $B$ .

Report an Error

Example Question #5 : How To Find The Area Of A Square

The perimeter of a square is $12\ in.$ If the square is enlarged by a factor of three, what is the new area?

Possible Answers:

$48\ in.^{2}$

$9\ in.^{2}$

$36\ in.^{2}$

$27\ in.^{2}$

$81\ in.^{2}$

Correct answer:

$81\ in.^{2}$

Explanation:

The perimeter of a square is given by $P=4s=12$ so the side length of the original square is $3\ in.$ The side of the new square is enlarged by a factor of 3 to give $s=9\ in.$

So the area of the new square is given by $A = s^{2} = (9)^{2} = 81 in^{2}$ .

Report an Error

Example Question #11 : Squares

A half circle has an area of $18\pi$ . What is the area of a square with sides that measure the same length as the diameter of the half circle?

Possible Answers:

108

144

Correct answer:

144

Explanation:

If the area of the half circle is $18\pi$ , then the area of a full circle is twice that, or $36\pi$ .

Use the formula for the area of a circle to solve for the radius:

36π = πr²

r = 6

If the radius is 6, then the diameter is 12. We know that the sides of the square are the same length as the diameter, so each side has length 12.

Therefore the area of the square is 12 x 12 = 144.

Report an Error

Example Question #41 : Quadrilaterals

In square WXYZ, point Q is the midpoint of side WZ. If the area of quadrilateral WXYQ is $\frac{1}{12}$ , what is the length of one side of square WXYZ?

Possible Answers:

$\frac{1}{3}$

$\frac{1}{9}$

$\frac{1}{2}$

$\frac{1}{6}$

$\frac{1}{12}$

Correct answer:

$\frac{1}{3}$

Explanation:

Let equal the length of one side of the square. Drawing a diagram yields WXYQ is a trapezoid with two right angles, parallel bases of and $\frac{1}{2}s$ , and a height of .

Area of WXYQ = $\frac{s+\frac{1}{2} s}{2}\cdot s=\frac{\frac{3}{2} s}{2}\cdot s =\frac{3}{4} s^2=\frac{1}{12}$

$\frac{4}{3} \cdot \frac{3}{4} s^2=\frac{1}{12} \cdot \frac{4}{3}$

$s^2=\frac{1}{9}$

$s=\frac{1}{3}$

Report an Error

Example Question #7 : Squares

Four perfect circles with equal dimensions fit perfectly in a square with a length and width of $4\:cm$ . What is the area of the space inside the square that does not include the circles? (Use 3.14 for the value of $\pi$ .)

Varsity_sat_problems

Possible Answers:

$2.00\:cm^2$

$16.00\:cm^2$

$12.86\:cm^2$

$3.44\:cm^2$

$9.13\:cm^2$

Correct answer:

$3.44\:cm^2$

Explanation:

Solving for the area not occupied by circles involves subtracting the area of all four circles from the total area of the square, so let's start by calculating the area of the square. We are told that it has a length and width of $4\:cm$ , so we just need to multiply these together:

$Area_{square} = 4\:cm\cdot 4\:cm=16\:cm^{2}$

Now, we need to calculate the area of one of the circles. Since the four circles fit perfectly into the square, each one has a diameter of $2\:cm$ and a radius of $1\:cm$ , because 2+2=4 and the square's sides are each $4\:cm$ long.

Knowing this, we can calculate the area of one of the circles using the equation for the area of a circle, $A_{circle}=\pi r^2$ . Substituting in $1\:cm$ for the value of the radius of one of the circles, we get:

$Area_{circle}=\pi\cdot (1\:cm)^{2}$

$Area_{circle}=\pi\cdot 1\:cm^2$

$Area_{circle}=\pi\:cm^2$

Now, we can multiply our result by to calculate the area encompassed by the four circles together and subtract that value from the area of the square to find the answer:

$Area_{four\:circles}=4\cdot \pi\:cm^2$

$Area_{four\:circles}=4\pi\:cm^2$

$Area_{square}-Area_{four\:circles}=16\:cm^2-4\pi\:cm^2$

$Area_{square}-Area_{four\:circles}=16\:cm^2-4(3.14)\:cm^2$

$Area_{square}-Area_{four\:circles}=16\:cm^2-12.56\:cm^2$

$Area_{square}-Area_{four\:circles}= 3.44\:cm^2$

Report an Error

Example Question #1 : How To Find The Area Of A Square

Find the area of a square with side length 4.

Possible Answers:

Correct answer:

Explanation:

To solve, simply use the formula for the area of a square.

Substitute the side length of four into the following equation.

Thus,

A=s^2=4^2=16

Report an Error

View SAT Mathematics Tutors

Alexandra
Certified Tutor

Georgia State University, Bachelor of Fine Arts, Studio Art (Photography).

View SAT Mathematics Tutors

Bill
Certified Tutor

SUNY Empire State College, Bachelors, General Sciences, Theater.

View SAT Mathematics Tutors

Noah
Certified Tutor

Bard College, Bachelors, Philosophy and Creative Writing (Dual Major). Sarah Lawrence College, Master of Fine Arts, Creative ...

All SAT Math Resources

16 Diagnostic Tests 660 Practice Tests Question of the Day Flashcards Learn by Concept

SAT Math Tutors in Top Cities:

Atlanta SAT Math Tutors, Austin SAT Math Tutors, Boston SAT Math Tutors, Chicago SAT Math Tutors, Dallas Fort Worth SAT Math Tutors, Denver SAT Math Tutors, Houston SAT Math Tutors, Kansas City SAT Math Tutors, Los Angeles SAT Math Tutors, Miami SAT Math Tutors, New York City SAT Math Tutors, Philadelphia SAT Math Tutors, Phoenix SAT Math Tutors, San Diego SAT Math Tutors, San Francisco-Bay Area SAT Math Tutors, Seattle SAT Math Tutors, St. Louis SAT Math Tutors, Tucson SAT Math Tutors, Washington DC SAT Math Tutors

Popular Courses & Classes

SSAT Courses & Classes in Dallas Fort Worth, SSAT Courses & Classes in Philadelphia, MCAT Courses & Classes in Philadelphia, MCAT Courses & Classes in Seattle, ISEE Courses & Classes in Atlanta, SSAT Courses & Classes in New York City, GRE Courses & Classes in Chicago, GMAT Courses & Classes in Seattle, MCAT Courses & Classes in Denver, SSAT Courses & Classes in Chicago

Popular Test Prep

MCAT Test Prep in Seattle, SSAT Test Prep in Houston, MCAT Test Prep in Miami, GRE Test Prep in Houston, ACT Test Prep in Los Angeles, ISEE Test Prep in Washington DC, LSAT Test Prep in Chicago, ISEE Test Prep in Miami, ACT Test Prep in San Francisco-Bay Area, ISEE Test Prep in Atlanta

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SAT Math : How to find the area of a square

Study concepts, example questions & explanations for SAT Math

All SAT Math Resources

Example Questions

Example Question #1 : How To Find The Area Of A Square

Example Question #2 : How To Find The Area Of A Square

Example Question #3 : How To Find The Area Of A Square

Example Question #4 : How To Find The Area Of A Square

Example Question #12 : Quadrilaterals

Example Question #5 : How To Find The Area Of A Square

Example Question #11 : Squares

Example Question #41 : Quadrilaterals

Example Question #7 : Squares

Example Question #1 : How To Find The Area Of A Square

All SAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: