The triangle in question can be drawn as the following:

The lengths of the legs of the triangle are 12, the distance from the origin to , and 8, the distance from the origin to . The area of a right triangle is equal to half the product of the lengths of the legs, so set  in the formula:

Drawing points gives sides of a right triangle of 4, 5, and an unknown hypotenuse.

Using the pythagorean theorem we find that the hypotenuse is .

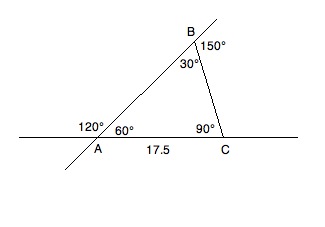
Consult the diagram above while reading the solution. Because of what we know about supplementary angles, we can fill in the inner values of the triangle. Angles A and B can be found by the following reductions:

A + 120 = 180; A = 60

B + 150 = 180; B = 30

Since we know A + B + C = 180 and have the values of A and B, we know:

60 + 30 + C = 180; C = 90

This gives us a 30:60:90 triangle. Now, since 17.5 is across from the 30° angle, we know that the other two sides will have to be √3 and 2 times 17.5; therefore, our perimeter will be as follows:

The figure shows a right triangle. The acute angles of a right triangle have measures whose sum is , so

Substituting  for :

This makes  a 45-45-90 triangle.

By the 45-45-90 Triangle Theorem, legs  and  are of the same length, so

.

Also by the 45-45-90 Triangle Theorem, the length of hypotenuse is equal to that of leg  multiplied by . Therefore, 

.

The perimeter of the triangle is 

The figure shows a right triangle. The acute angles of a right triangle have measures whose sum is , so

Substituting  for :

This makes  a 45-45-90 triangle. By the 45-45-90 Triangle Theorem, the length of leg  is equal to that of hypotenuse , the length of which is 12, divided by . Therefore,

Rationalize the denominator by multiplying both halves of the fraction by :

By the same reasoning, .

The perimeter of the triangle is 

This problem is solved using the Pythagorean theorem  .  In this formula  and  are the legs of the right triangle while  is the hypotenuse.

Using the labels of our triangle we have:

Using the Pythagorean theorem, x² + y² = h². And since it is a 45-45-90 triangle the two short sides are equal. Therefore 5² + 5² = h² .  Multiplied out 25 + 25 = h².

Therefore h² = 50, so h = √50 = √2 * √25 or 5√2.

The best thing to do here is to draw diagram and draw the appropiate triangle for what is being asked. It does not matter where you place your point on the base because any point will produce the same result.  We know that the center of the base of the cylinder is 3 inches away from the base (6/2). We also know that the center of the cylinder is 5 inches from the base of the cylinder (10/2). So we have a right triangle with a height of 5 inches and a base of 3 inches. So using the Pythagorean Theorem 3² + 5² = c². 34 = c², c = √(34).

The correct answer is 5. The pythagorean theorem states that a² + b² = c². So in this case 3² + 4² = C². So C² = 25 and C = 5. This is also an example of the common 3-4-5 triangle. 

We are told that the lengths form a series of consecutive even integers. Because even integers are two units apart, the side lengths must differ by two. In other words, the largest side length is two greater than the second largest, and the second largest length is two greater than the smallest length. 

The second largest length is equal to x. The second largest length must thus be two less than the largest length. We could represent the largest length as x + 2. 

Similarly, the second largest length is two larger than the smallest length, which we could thus represent as x – 2. 

To summarize, the lengths of the triangle (in terms of x) are x – 2, x, and x + 2.

In order to solve for x, we can make use of the fact that the triangle is a right triangle. If we apply the Pythagorean Theorem, we can set up an equation that could be used to solve for x. The Pythagorean Theorem states that if a and b are the lengths of the legs of the triangle, and c is the length of the hypotenuse, then the following is true:

a² + b² = c²

In this particular case, the two legs of our triangle are x – 2 and x, since the legs are the two smallest sides; therefore, we can say that a = x – 2, and b = x. Lastly, we can say c = x + 2, since x + 2 is the length of the hypotenuse. Subsituting these values for a, b, and c into the Pythagorean Theorem yields the following:

(x – 2)² + x² = (x + 2)²

The answer is (x – 2)² + x² = (x + 2)².