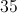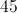Since the three angles of a triangle sum to 180, we know that 70 + x + y = 180. Subtract 70 from both sides and see that x + y = 110. Subtract x from both sides and see that y = 110 – x.

The sum of the interior angles, in degrees, of a regular polygon is given by the formula 180(n – 2), where n is the number of sides. The problem concerns a polygon with twelve sides, so we will let n = 12. The sum of the interior angles in this polygon would be 180(12 – 2) = 180(10) = 1800.

Because the polygon is regular (meaning its sides are all congruent), all of the angles have the same measure. Thus, if we divide the sum of the measures of the angles by the number of sides, we will have the measure of each interior angle. In short, we need to divide 1800 by 12, which gives us 150.

The answer is 150.

Angle FHI is the supplement of angle FHG, which is an interior angle in the octagon. When two angles are supplementary, their sum is equal to 180 degrees. If we can find the measure of each interior angle in the octagon, then we can find the supplement of angle FHG, which will give us the measure of angle FHI.

The sum of the interior angles in a regular polygon is given by the formula 180(n – 2), where n is the number of sides in the polygon. An octagon has eight sides, so the sum of the angles of the octagon is 180(8 – 2) = 180(6) = 1080 degrees. Because the octagon is regular, all of its sides and angles are congruent. Thus, the measure of each angle is equal to the sum of its angles divided by 8. Therefore, each angle in the polygon has a measure of 1080/8 = 135 degrees. This means that angle FHG has a measure of 135 degrees.

Now that we know the measure of angle FHG, we can find the measure of FHI. The sum of the measures of FHG and FHI must be 180 degrees, because the two angles form a line and are supplementary. We can write the following equation:

Measure of FHG + measure of FHI = 180

135 + measure of FHI = 180

Subtract 135 from both sides.

Measure of FHI = 45 degrees.

The answer is 45. 

An octagon contains six triangles, or 1080 degrees. This means with 8 angles, each angle is 135 degrees.

There are 360 degrees and 8 angles, so dividing leaves 45 degrees per angle.

The 4 angles of a quadrilateral add to 360

The 5 angles of a pentagon add to 540

The 6 angles of a hexagon add to 720

To solve, simply use the formula for the total degrees in a polygon, where n is the number of vertices.

In this particular case, a nonagon is a shape with nine sides and thus nine vertices.

Thus,

If one exterior angle is taken at each vertex of any convex polygon, the sum of their measures is . In a regular polygon - one with congruent sides and congruent interior angles, each exterior angle is congruent to one another. If the polygon has  sides, each exterior angle has measure .

Given the common measure , 

Multiplying both sides by :

and

Since  is equal to a number of sides, it is a whole number. Thus, we are looking for a value of  which, when we divide 360 by it, yields a non-whole result. We see that  is the correct choice, since'

A quick check confirms that 360 divided by 8, 10, 12, or 15 yields a whole result.

The measure of each interior angle of an -sided polygon can be calculated using the formula

Setting :

The correct choice is therefore .

The easiest way to work this is arguably to examine the exterior angles, each of which forms a linear pair with an interior angle. If an interior angle measures , then each exterior angle, which is supplementary to an interior angle, measures

The measures of the exterior angles of a polygon, one per vertex, total ; in a regular polygon, they are congruent, so if there are  such angles, each measures . Since the number of vertices is equal to the number of sides, if we set this equal to  and solve for , we will find the number of sides.

Multiply both sides by :

The polygon has 72 vertices and, thus, 72 sides.