All SAT Math Resources
Example Questions
Example Question #151 : Algebra
If –1 < n < 1, all of the following could be true EXCEPT:
(n-1)2 > n
16n2 - 1 = 0
n2 < 2n
|n2 - 1| > 1
n2 < n
|n2 - 1| > 1
Example Question #3 : How To Find The Solution To An Inequality With Multiplication
(√(8) / -x ) < 2. Which of the following values could be x?
-2
-1
-4
All of the answers choices are valid.
-3
-1
The equation simplifies to x > -1.41. -1 is the answer.
Example Question #31 : Inequalities
Solve for x
Example Question #32 : Inequalities
We have 
Example Question #4 : How To Find The Solution To An Inequality With Multiplication
Fill in the circle with either 




The rational expression is undefined.
None of the other answers are correct.
Let us simplify the second expression. We know that:
So we can cancel out as follows:
Example Question #2 : How To Find The Solution To An Inequality With Multiplication
What value must 

Add 
Multiply each side of the inequality by 
Multiply each side of the inequality by 
Divide each side of the inequality by 
You can now change the fraction on the right side of the inequality to decimal form.
The correct answer is 

Example Question #156 : Equations / Inequalities
Give the solution set of this inequality:
The set of all real numbers
The absolute value inequality

can be rewritten as the compound inequality

Solve each inequality separately, using the properties of inequality to isolate the variable on the left side:
Subtract 17 from both sides:
Divide both sides by 

which in interval notation is
The same steps are performed with the other inequality:
which in interval notation is ![(-\infty, -12.5 ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/787071/gif.latex)
The correct response is the union of these two sets, which is
![(-\infty, -12.5 ] \cup [21, \infty )](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/787072/gif.latex)
Example Question #3 : How To Find The Solution To An Inequality With Multiplication
Find the maximum value of 
First step is to rewrite
Next step is to find the vertices of the bounded region. We do this by plugging in the x bounds into the 
The vertices are
Now we plug each coordinate into 
So the maximum value is
Example Question #152 : Algebra
Each of the following is equivalent to
xy/z * (5(x + y)) EXCEPT:
5x² + y²/z
xy(5x + 5y)/z
5x²y + 5xy²/z
xy(5y + 5x)/z
5x² + y²/z
Choice a is equivalent because we can say that technically we are multiplying two fractions together: (xy)/z and (5(x + y))/1. We multiply the numerators together and the denominators together and end up with xy (5x + 5y)/z. xy (5y + 5x)/z is also equivalent because it is only simplifying what is inside the parentheses and switching the order- the commutative property tells us this is still the same expression. 5x²y + 5xy²/z is equivalent as it is just a simplified version when the numerators are multiplied out. Choice 5x² + y²/z is not equivalent because it does not account for all the variables that were in the given expression and it does not use FOIL correctly.
Example Question #1 : How To Find The Solution To An Inequality With Division
Let S be the set of numbers that contains all of values of x such that 2x + 4 < 8. Let T contain all of the values of x such that -2x +3 < 8. What is the sum of all of the integer values that belong to the intersection of S and T?
2
-3
-7
-2
0
-2
First, we need to find all of the values that are in the set S, and then we need to find the values in T. Once we do this, we must find the numbers in the intersection of S and T, which means we must find the values contained in BOTH sets S and T.
S contains all of the values of x such that 2x + 4 < 8. We need to solve this inequality.
2x + 4 < 8
Subtract 4 from both sides.
2x < 4
Divide by 2.
x < 2
Thus, S contains all of the values of x that are less than (but not equal to) 2.
Now, we need to do the same thing to find the values contained in T.
-2x + 3 < 8
Subtract 3 from both sides.
-2x < 5
Divide both sides by -2. Remember, when multiplying or dividing an inequality by a negative number, we must switch the sign.
x > -5/2
Therefore, T contains all of the values of x that are greater than -5/2, or -2.5.
Next, we must find the values that are contained in both S and T. In order to be in both sets, these numbers must be less than 2, but also greater than -2.5. Thus, the intersection of S and T consists of all numbers between -2.5 and 2.
The question asks us to find the sum of the integers in the intersection of S and T. This means we must find all of the integers between -2.5 and 2.
The integers between -2.5 and 2 are the following: -2, -1, 0, and 1. We cannot include 2, because the values in S are LESS than but not equal to 2.
Lastly, we add up the values -2, -1, 0, and 1. The sum of these is -2.
The answer is -2.
All SAT Math Resources

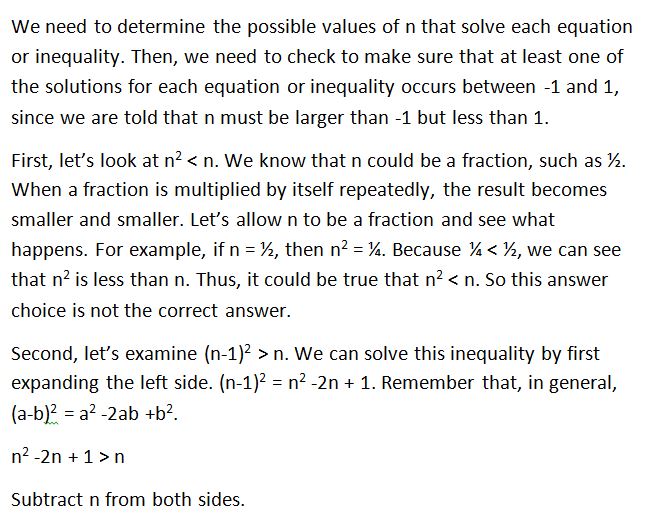







































![(-\infty, -12.5 ] \cup [21, \infty )](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/786962/gif.latex)
![[-21, 12.5]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/786964/gif.latex)
![(-\infty, -21 ] \cup [12.5, \infty )](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/786965/gif.latex)
![[-12.5, 21]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/786963/gif.latex)



































