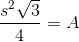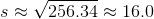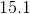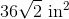We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : Tetrahedrons

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : How To Find The Length Of An Edge Of A Tetrahedron

Tetra_1

Refer to the above tetrahedron, or four-faced solid. The surface area of the tetrahedron is 444. Evaluate to the nearest tenth.

Possible Answers:

$s \approx 21.1$

$s \approx 314.0$

$s \approx 17.8$

$s \approx 16.0$

$s \approx 256.3$

Correct answer:

$s \approx 16.0$

Explanation:

The tetrahedron has four faces, each of which is an equilateral triangle with sidelength . Since the total surface area is 444, each triangle has area one fourth of this, or 111. To find , set A = 111 in the formula for the area of an equilateral triangle:

$\frac{s^{2} \sqrt{3} }{4} =A$

$\frac{s^{2} \sqrt{3} }{4} =111$

$s^{2} \sqrt{3} =444$

$s^{2}=\frac{444}{ \sqrt{3} } \approx \frac{444}{ 1.732 } \approx 256.34$

$s\approx \sqrt{256.34} \approx 16.0$

Report an Error

Example Question #1 : How To Find The Volume Of A Tetrahedron

Tetra_2

Note: Figure NOT drawn to scale.

The above triangular pyramid has volume 25. To the nearest tenth, evaluate .

Possible Answers:

$h \approx 7.2$

$h \approx 10.8$

$h \approx 8.8$

$h \approx 13.3$

Insufficient information is given to answer the problem.

Correct answer:

$h \approx 10.8$

Explanation:

We are looking for the height of the pyramid.

The base is an equilateral triangle with sidelength 4, so its area can be calculated as follows:

$B = \frac{s^{2} \sqrt{3} }{4}$

$B = \frac{4^{2} \sqrt{3} }{4}$

$B = \frac{16 \sqrt{3} }{4}$

$B = 4\sqrt{3}$

The height of a pyramid can be calculated using the fomula

$V = \frac{1}{3}Bh$

We set V = 25 and $B = 4\sqrt{3}$ and solve for :

$\frac{1}{3} \cdot 4\sqrt{3} \cdot h = 25$

$h = \frac{25 \cdot 3}{4 \sqrt{3}} = \frac{75}{4 \sqrt{3}} \approx \frac{75}{4 \cdot 1.7321} \approx 10.8$

Report an Error

Example Question #2 : How To Find The Volume Of A Tetrahedron

Tetra_1

Note: Figure NOT drawn to scale.

Give the volume (nearest tenth) of the above triangular pyramid.

Possible Answers:

55.4

27.7

18.5

22.6

15.1

Correct answer:

18.5

Explanation:

The height of the pyramid is h = 8 . The base is an equilateral triangle with sidelength 4, so its area can be calculated as follows:

$B = \frac{s^{2} \sqrt{3} }{4}$

$B = \frac{4^{2} \sqrt{3} }{4}$

$B = \frac{16 \sqrt{3} }{4}$

$B = 4\sqrt{3}$

The volume of a pyramid can be calculated using the fomula

$V = \frac{1}{3}Bh$

$V = \frac{1}{3} \cdot 4\sqrt{3} \cdot 8 = \frac{32\sqrt{3} }{3} \approx \frac{32 \cdot 1.7321}{3} \approx 18.5$

Report an Error

Example Question #1 : How To Find The Volume Of A Tetrahedron

A regular tetrahedron has an edge length of . What is its volume?

Possible Answers:

$\frac{64}{3\sqrt{2}}$

$\frac{32}{3\sqrt{2}}$

$\frac{32}{\sqrt{2}}$

$\frac{32}{3}$

Correct answer:

$\frac{32}{3\sqrt{2}}$

Explanation:

The volume of a tetrahedron is found with the equation $V=\frac{a^3}{6\sqrt{2}}$ , where represents the length of an edge of the tetrahedron.

Plug in 4 for the edge length and reduce as much as possible to find the answer:

$V=\frac{4^3}{6\sqrt{2}}$

$V=\frac{64}{6\sqrt{2}}$

$V=\frac{32}{3\sqrt{2}}$

The volume of the tetrahedron is $\frac{32}{3\sqrt{2}}$ .

Report an Error

Example Question #1 : How To Find The Surface Area Of A Tetrahedron

A regular tetrahedron has four congruent faces, each of which is an equilateral triangle.

A given tetrahedron has edges of length six inches. Give the total surface area of the tetrahedron.

Possible Answers:

$9 \sqrt{3}\textrm{ in}^{2}$

$36 \textrm{ in}^{2}$

$9 \sqrt{2}\textrm{ in}^{2}$

$36 \sqrt{2}\textrm{ in}^{2}$

$36 \sqrt{3}\textrm{ in}^{2}$

Correct answer:

$36 \sqrt{3}\textrm{ in}^{2}$

Explanation:

The area of an equilateral triangle is given by the formula

$A = \frac{s^{2}\sqrt{3}}{4}$

Since there are four equilateral triangles that comprise the surface of the tetrahedron, the total surface area is

$SA = 4 A = 4 \cdot \frac{s^{2}\sqrt{3}}{4} = s^{2}\sqrt{3}$

Substitute s = 6 :

$SA = s^{2}\sqrt{3} = 6^{2}\sqrt{3} = 36\sqrt{3}$ square inches.

Report an Error

Example Question #1 : How To Find The Surface Area Of A Tetrahedron

Tetra_1

Give the surface area of the above tetrahedron, or four-faced solid, to the nearest tenth.

Possible Answers:

114.3

69.3

84.9

Insufficient information is given to answer the question.

98.0

Correct answer:

84.9

Explanation:

The tetrahedron has four faces, each of which is an equilateral triangle with sidelength 7. Each face has area

$A = \frac{s^{2} \sqrt{3} }{4} = \frac{7^{2} \sqrt{3} }{4} = \frac{49 \sqrt{3} }{4} \approx \frac{49 \cdot 1.7321}{4} \approx 21.22$

The total surface area is four times this, or about $4 \cdot 21.22 = 84.88$ .

Rounded, this is 84.9.

Report an Error

View PSAT Mathematics Tutors

Rodolfo
Certified Tutor

The University of Texas at San Antonio, Bachelor of Science, Mathematics.

View PSAT Mathematics Tutors

Benjamin
Certified Tutor

Portland State University, Bachelor of Science, Computer Science.

View PSAT Mathematics Tutors

Alice
Certified Tutor

The University of Texas at Dallas, Bachelor of Science, Accounting.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

SAT Tutors in New York City, Statistics Tutors in Boston, Biology Tutors in Seattle, Reading Tutors in Chicago, MCAT Tutors in Los Angeles, Algebra Tutors in Miami, Calculus Tutors in Seattle, English Tutors in Denver, MCAT Tutors in Seattle, Spanish Tutors in Boston

Popular Courses & Classes

Spanish Courses & Classes in San Diego, ACT Courses & Classes in Denver, SAT Courses & Classes in Houston, SAT Courses & Classes in Seattle, SSAT Courses & Classes in Los Angeles, ISEE Courses & Classes in Chicago, MCAT Courses & Classes in Philadelphia, ISEE Courses & Classes in Phoenix, GMAT Courses & Classes in Atlanta, ISEE Courses & Classes in San Diego

Popular Test Prep

SAT Test Prep in Phoenix, GMAT Test Prep in Houston, SAT Test Prep in Los Angeles, ISEE Test Prep in Washington DC, SAT Test Prep in Philadelphia, ACT Test Prep in Miami, LSAT Test Prep in Philadelphia, ISEE Test Prep in Los Angeles, ACT Test Prep in Denver, MCAT Test Prep in Los Angeles

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : Tetrahedrons

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #1 : How To Find The Length Of An Edge Of A Tetrahedron

Example Question #1 : How To Find The Volume Of A Tetrahedron

Example Question #2 : How To Find The Volume Of A Tetrahedron

Example Question #1 : How To Find The Volume Of A Tetrahedron

Example Question #1 : How To Find The Surface Area Of A Tetrahedron

Example Question #1 : How To Find The Surface Area Of A Tetrahedron

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: