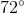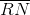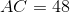How do you find the area of a hexagon?

There are several ways to find the area of a hexagon.

1. In a regular hexagon, split the figure into triangles.
2. Find the area of one triangle.
3. Multiply this value by six.

Alternatively, the area can be found by calculating one-half of the side length times the apothem.

Regular hexagons:

Regular hexagons are interesting polygons. Hexagons are six sided figures and possess the following shape:

In a regular hexagon, all sides equal the same length and all interior angles have the same measure; therefore, we can write the following expression.

One of the easiest methods that can be used to find the area of a polygon is to split the figure into triangles. Let's start by splitting the hexagon into six triangles. 

In this figure, the center point, , is equidistant from all of the vertices. As a result, the six dotted lines within the hexagon are the same length. Likewise, all of the triangles within the hexagon are congruent by the side-side-side rule: each of the triangle's share two sides inside the hexagon as well as a base side that makes up the perimeter of the hexagon. In a similar fashion, each of the triangles have the same angles. There are  in a circle and the hexagon in our image has separated it into six equal parts; therefore, we can write the following:

We also know the following:

Now, let's look at each of the triangles in the hexagon. We know that each triangle has two two sides that are equal; therefore, each of the base angles of each triangle must be the same. We know that a triangle has  and we can solve for the two base angles of each triangle using this information.

Each angle in the triangle equals . We now know that all the triangles are congruent and equilateral: each triangle has three equal side lengths and three equal angles. Now, we can use this vital information to solve for the hexagon's area. If we find the area of one of the triangles, then we can multiply it by six in order to calculate the area of the entire figure. Let's start by analyzing . If we draw, an altitude through the triangle, then we find that we create two  triangles. 

Let's solve for the length of this triangle. Remember that in  triangles, triangles possess side lengths in the following ratio:

Now, we can analyze  using the a substitute variable for side length, .

We know the measure of both the base and height of  and we can solve for its area.

Now, we need to multiply this by six in order to find the area of the entire hexagon.

We have solved for the area of a regular hexagon with side length, . If we know the side length of a regular hexagon, then we can solve for the area.

If we are not given a regular hexagon, then we an solve for the area of the hexagon by using the side length(i.e. ) and apothem (i.e. ), which is the length of a line drawn from the center of the polygon to the right angle of any side. This is denoted by the variable  in the following figure:

Alternative method:

If we are given the variables  and , then we can solve for the area of the hexagon through the following formula:

In this equation,  is the area,  is the perimeter, and  is the apothem. We must calculate the perimeter using the side length and the equation , where  is the side length.

Solution:

In the given problem we know that the side length of a regular hexagon is the following:

Let's substitute this value into the area formula for a regular hexagon and solve.

Simplify.

Round the answer to the nearest whole number.

The formula for the sum of the interior angles of any regular polygon is as follows:

where  is equal to the number of sides of the regular polygon.

Therefore, the sum of the interior angles for a regular pentagon is:

To find the measure of one interior angle of a regular pentagon, simply divide by the number of sides (or number of interior angles):

The measure of one interior angle of a regular pentagon is 108 degrees.

By angle addition,

 is one of two acute angles of isosceles right triangle , so .

To find  we examine .

 is an angle of a regular pentagon and has measure .

Also, since, in , sides , by the Isosceles Triangle Theorem, . 

Since the angles of a triangle must total  in measure, 

If , then  has short sides  and long side . Since

,

then, by the converse of the Pythagorean Theorem,  is a right triangle with right angle . Statement I is sufficient.

If  and  are both acute,we know nothing about ; every triangle has at least two acute angles regardless of type. Statement II tells us nothing.

 and  form a linear pair and are therefore supplementary. If one is a right angle, so is the other. Therefore, if   is a right angle, so is . Statement III is sufficient.

The correct response is Statement I and III only.

Two angles formed by a transversal line crossing two other lines are corresponding angles if, relative to the points of intersection, they are in the same position.  is formed by the intersection of transversal  and ; the angle in the same relative position where  intersects  is .

Two angles formed by a transversal line crossing two other lines are alternate interior angles if:

I) Both angles have their interiors between the lines crossed

II) The angles have their interiors on the opposite sides of the transversal.

Of the given choices, only  fits the description; the interior of each is between  and , and the interiors are on the opposite sides of .

Two angles are vertical angles if they share a vertex, anf if their union is a pair of intersecting lines. Of the five choices, only  fits both descriptions with .

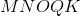
A diagonal of a polygon is a segment whose endpoints are nonconsecutive vertices of the polygon. Of the five choices, only  fits this description.

The area of a rhombus is found by

A = 1/2(d₁)(d₂)

where d₁ and d₂ are the lengths of the diagonals.  Substituting for the given values yields

24 = 1/2(d₁)(6)

24 = 3(d₁)

8 = d₁

Now, use the facts that diagonals are perpendicular in a rhombus, diagonals bisect each other in a rhombus, and the Pythagorean Theorem to determine that the two diagonals form 4 right triangles with leg lengths of 3 and 4.  Since 3² + 4² = 5², each side length is 5, so the perimeter is 5(4) = 20.

, so Quadrilateral  is a rhombus. Its diagonals are therefore perpendicular to each other, and the four triangles they form are right triangles. Therefore, the Pythagorean theorem can be used to determine the common sidelength of Quadrilateral .

We focus on . The diagonals are also each other's bisector, so 

By the Pythagorean Theorem, 

26 is the common length of the four sides of Quadrilateral , so its perimeter is .