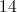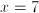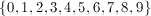PSAT Math : PSAT Mathematics
Study concepts, example questions & explanations for PSAT Math
All PSAT Math Resources
Example Questions
Example Question #131 : Integers
A company assigns employee numbers according to the following scheme:
1) Each number must comprise three letters, followed by four numerals, followed by one letter.
2) There are no restrictions on the numerals.
3) There cannot be repetition among the first three letters; however, the final letter can be any letter, even if that letter is among the first three.
Which of the following expressions is equal to the number of possible employee numbers?
The first three characters must be distinct letters, meaning that three letters will be selected from a set of 26. Also, order will be important, so the number of ways to choose this group of three will be 
The next four characters will be numerals, with no restrictions, so the number of ways to choose this group will be
The last character can be any of 26 letters.
By the multiplication principle, the number of ways to choose an employee number will be
Example Question #1 : How To Find Permutation Notation
A company assigns employee numbers according to the following scheme:
1) Each number must comprise two letters, followed by four numerals, followed by two letters.
2) There are no restrictions on the numerals.
3) There cannot be repetition between the first two letters.
4) There cannot be repetition between the last two letters.
5) A letter appearing as one of the first two letters can appear as one of the last two.
Which of the following expressions is equal to the number of possible employee numbers?
The first two characters must be distinct letters, meaning that two letters will be selected from a set of 26. Also, order will be important, so the number of ways to choose this group of two will be 
Similarly, since the last two characters will be chosen according to the same rule, with repetition allowed between the two groups, there will be 
The next four characters will be numerals, but there will be no restrictions, so the number of ways to choose this group will be 
By the multiplication principle, the number of ways to choose an employee number will be
Example Question #1 : How To Find The Number Of Integers Between Two Other Integers
A custom-made ruler is 
There will be 15 gaps of 
Example Question #1711 : Sat Mathematics
How many prime numbers are between 1 to 25?
A prime numbers is a number greater than 1 that can only be divided by 1 and itself. Simply count from 1 to 25 and see how many values fit the criteria.
2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20, 21, 22, 23, 24, 25
Prime numbers are underlined. Nine prime numbers are in this set interval.
Example Question #181 : Integers
Four consecutive odd integers sum to 40. How many of these numbers are prime?
Let x equal the smallest of the four numbers. Therefore:
Therefore the four odd numbers are 7, 9, 11, and 13. Since all are prime except 9, three of the numbers are prime.
Example Question #2 : How To Find The Number Of Integers Between Two Other Integers
Find the median of this set of numbers
To find the median of a set of numbers, we must first order them from smallest to greatest.
Next, we must find the number directly in the middle, which has an equal number of numbers to the right and to the left.
Therefore, our answer is 
Example Question #1 : How To Find The Missing Number In A Set
Which number completes the following series: 1, 2, 4, 8, 16, 32, 64, _?
Not enough information
16
64
128
15
128
All of the numbers in this series are 2n-1. The number that we are looking for is the eighth number. So 28–1 = 27 = 128.
Example Question #2 : How To Find The Missing Number In A Set
Alhough Danielle’s favorite flowers are tulips, she wants at least one each of three different kinds of flowers in her bouquet. Roses are twice as expensive as lilies and lilies are 25% of the price of tulips. If a rose costs $20 and Danielle only has $130, how many tulips can she buy?
4
3
2
5
1
2
She can only buy 2 tulips at $80, because if she bought 3 she wouldn’t have enough to afford the other 2 kinds of flowers. She has to spend at least 30 dollars (20 + 10) on 1 rose and 1 lily.
Example Question #1 : How To Find The Missing Number In A Set
Which of the following is not a rational number?
5
1.75
√2
0.111...
.001
√2
A rational number is a number that can be written in the form of a/b, where a and b are integers, aka a real number that can be written as a simple fraction or ratio. 4 of the 5 answer choices can be written as fractions and are thus rational.
5 = 5/1, 1.75 = 7/4, .001 = 1/1000, 0.111... = 1/9
√2 cannot be written as a fraction because it is irrational. The two most famous irrational numbers are √2 and pi.
Example Question #2 : Sets
Which set represents all the single-digits integers (0-9) that are either prime, a perfect square, or found in the number 68?
The prime digits are 2, 3, 5, and 7.
The perfect square digits are 0, 1, 4, and 9.
The only digits not represent in these two groups are 6 and 8, which are, coincidentally, found in the number 68.
Certified Tutor
All PSAT Math Resources
