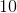The numerator, being a polynomial, is not restricting our domain. The domain is, however, restricted by the expression in the denominator, which must be nonzero. Furthermore, the radicand must be nonnegative. Combined, these facts mean that the radicand must be positive, and that the following inequality be solved:

 or, equivalently, .

In interval form, this is 

The domain of the product of two functions is the intersection of the domains of the individual functions.

The domain of  is restricted to all values of  that yield a nonzero denominator. Since this means that 

,

then, subsequently,

,

so the domain is the set of all real numbers except 4.

Similarly, the domain of  is restricted to all values of  that yield a nonzero denominator. This set is found to be the set of all real numbers except 7.

The intersection of these sets is the set of all real numbers except 4 and 7, or

.

The definition of  has two denominators of two fractions (one within the other), so we must exclude the values of  that make either denominator equal to zero.

One denominator is . Since it cannot be zero, we have 

,

and, subsequently,

.

The value 2 is excluded from the domain.

The other denominator is . If it is equal to zero, then

Therefore, this value is also excluded from the domain.

The correct domain is the set .

The numerator , being a polynomial, does not restrict the domain.

 appears as a radicand of a square root in the definition of , so  must be restricted to nonnegative numbers. Also,  is a denominator, which means 0 must also be excluded. Therefore, we are restricted so far to positive numbers.

There is one more denominator, which is ; it must be nonzero. We set this equal to zero to determine any additional value(s) that must be excluded:

Therefore, the domain is the set of all positive numbers except 9 - or

.

Given a fractional algebraic equation with variables in the numerator and denominator of one side and the other side equal to zero, we rely on a simple concept.  Zero divided by anything equals zero. That means we can focus in on what values make the numerator (the top part of the fraction) zero, or in other words,

The expression  is a difference of squares that can be factored as 

Solving this for  gives either  or .  That means either of these values will make our numerator equal zero.  We might be tempted to conclude that both are valid answers.  However, our statement earlier that zero divided by anything is zero has one caveat. We can never divide by zero itself.  That means that any values that make our denominator zero must be rejected.  Therefore we must also look at the denominator.

The left side factors as follows

This means that if  is  or , we end up dividing by zero.  That means that  cannot be a valid solution, leaving  as the only valid answer.  Therefore only #3 is correct. 

The absolute value will always be positive or 0, therefore all values of z will create a true statement as long as . Thus all values except for 2 will work.

If we can find the sum of , , and 10, we can determine their average. There is not enough information to solve for  or  individually, but we can find their sum, . 

Write out the average formula for the original three quantities.  Remember, adding together and dividing by the number of quantities gives the average: 

Isolate : 

Write out the average formula for the new three quantities: 

Combine the integers in the numerator:

Replace  with 27:

To find the excluded values of a algebraic fraction you need to find when the denominator is zero. To find when the denominator is zero you need to factor it. This denominator factors into 

so this is zero when x=4,7 so our answer is 

Because the number of days goes down as the number of children goes up, this problem type is inverse variation. We can solve this problem by the following steps:

20*6=24*x

120=24x

x=120/24

x=5

In this equation, x represents the total number of days that can be weathered by 24 students. This is down from the 6 days that 20 students could take shelter together. So the difference is 1 day less.

If  varies inversely as the cube of , then, if  are the initial values of the variables and  are the final values,

Substitute  and find :

This rounds to 2.3.