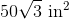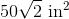All PSAT Math Resources
Example Questions
Example Question #1 : Cubes
What is the length of the diagonal of a cube with surface area of 294 in2?
None of the other answers
21
21√(2)
7√(3)
14
7√(3)
The first thing necessary is to determine the dimensions of the cube. This can be done using the surface area formula for cubes: A = 6s2, where s is the length of the cube. For our data, this is:
6s2 = 294
s2 = 49
(taking the square root of both sides) s = 7
The distance from corner to corner of the cube will be equal to the distance between (0,0,0) and (7,7,7). The distance formula for three dimensions is very similar to that of 2 dimensions (and hence like the Pythagorean Theorem):
d = √((x1 – x2)2 + (y1 – y2)2 + (z1 – z2)2)
Or for our simpler case:
d = √((x)2 + (y)2 + (z)2) = √( (s)2 + (s)2 + (s)2) = √( (7)2 + (7)2 + (7)2) = √( 49 + 49 + 49) = √(49 * 3) = 7√(3)
Example Question #2 : How To Find The Diagonal Of A Cube
A rectangular prism has a volume of 144 and a surface area of 192. If the shortest edge is 3, what is the length of the longest diagonal through the prism?
The volume of a rectangular prism is 
We are told that the shortest edge is 3. Let us call this the height.
We now have 

Now we replace variables by known values:
Now we have:
We have thus determined that the other two edges of the rectangular prism will be 4 and 12. We now need to find the longest diagonal. This is equal to:
If you do not remember how to find this directly, you can also do it in steps. You first find the diagonal across one of the sides (in the plane), by using the Pythagorean Theorem. For example, we choose the side with edges 3 and 4. This diagonal will be:
We then use a plane with one side given by the diagonal we just found (length 5) and the other given by the distance of the 3rd edge (length 12).
This diagonal is then 
Example Question #1 : Solid Geometry
The number of square units in the surface area of a cube is twice as large as the number of cubic units in its volume. What is the cube's volume, in cubic units?
36
216
9
108
27
27
The number of square units in the surface area of a cube is given by the formula 6s2, where s is the length of the side of the cube in units. Moreover, the number of cubic units in a cube's volume is equal to s3.
Since the number of square units in the surface area is twice as large as the cubic units of the volume, we can write the following equation to solve for s:
6s2 = 2s3
Subtract 6s2 from both sides.
2s3 – 6s2 = 0
Factor out 2s2 from both terms.
2s2(s – 3) = 0
We must set each factor equal to zero.
2s2 = 0, only if s = 0; however, no cube has a side length of zero, so s can't be zero.
Set the other factor, s – 3, equal to zero.
s – 3 = 0
Add three to both sides.
s = 3
This means that the side length of the cube is 3 units. The volume, which we previously stated was equal to s3, must then be 33, or 27 cubic units.
The answer is 27.
Example Question #1 : How To Find The Length Of An Edge Of A Cube
What is the surface area of a cube whose volume is 512 cubic feet?
In order to find the surface area of a cube, we need to solve for the length of each side, 
Recall the formula for volume:
Plug in what we know and solve for 
Now plug this value into the surface area formula:
Example Question #241 : Psat Mathematics
If the volume of a cube is 64 cubic inches, then it has an edge length of _______.
8 in
2 in
9 in
4 in
6 in
4 in
Example Question #1 : How To Find The Length Of An Edge Of A Cube
If the volume of a cube is 512 units, what is the length of one edge of the cube?
The volume of a cube is length x width x height. Since it's a cube, though, the length, width, and height are all equal, and equivalent to the length of one edge of the cube. Therefore, to find the lenght of an edge of the cube, just find the cube root of the volume. In this case, the cube root of 512 is equal to 8.
Example Question #4 : How To Find The Length Of An Edge Of A Cube
Find the length of an edge of a cube that has a volume of 






All the edges of a cube have the same length, and the volume of a cube is the length of an edge taken to the third power.
So if we take the edge of the cube to be of length x, then:
So the length of the edge of a cube with a volume of 125 is 5.
Example Question #242 : Geometry
A certain shipping company has cubic boxes. One of these boxes has a volume of 
The formula for the volume of a cube is
where 
Here, the volume is 729. To find the side length, take the cube root of both sides:
The cube root of 729 is 9, so the length of each side of the cube is 9 feet.
Example Question #6 : How To Find The Length Of An Edge Of A Cube
If a cube has a volume of 
The formula for the volume of a cube is 
This means one edge of the cube is 

Example Question #1 : How To Find The Surface Area Of A Polyhedron
A regular octahedron has eight congruent faces, each of which is an equilateral triangle.
A given octahedron has edges of length five inches. Give the total surface area of the octahedron.
The area of an equilateral triangle is given by the formula
Since there are eight equilateral triangles that comprise the surface of the octahedron, the total surface area is
Substitute 

Certified Tutor
All PSAT Math Resources



















![side=\sqrt[3]{64}=4](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/227989/gif.latex)



















![\sqrt[3]{729}=s](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/254145/gif.latex)






![\sqrt[3]614.125=8.5](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/369263/gif.latex)