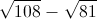PSAT Math : Arithmetic
Study concepts, example questions & explanations for PSAT Math
All PSAT Math Resources
Example Questions
Example Question #1 : How To Find A Fraction From A Percentage
Find the simplified fraction for
For any percent 
So we can write 
Then we simplify
So our simplified answer is
Example Question #171 : Arithmetic
You have 2 fair dice that each have 6 faces. On one roll, what's the probability that both dice land on an even number?
In order to find the probability of rolling two even numbers on 2 dice we need to find the probability of each dice having an even number on the roll and then multiply them together.
The probability of rolling an even number is
because 2, 4, and 6 are even numbers which goes in the numerator and there are 6 total numbers which goes in the denominator. After this we reduce the fraction and get one half. Then we need to muliply this by probability of the second dice having an even number.
Example Question #1 : Fractions And Percentage
What is 15% of a $16.73 bill?
We can re write 15% as a fraction and then use proportions to solve.
From here we cross multiply and divide to solve for 
Example Question #2 : How To Find Fractional Percentages
If a test has a total of 
To solve this problem we set up a ratio. We want to find 
In the case 
Example Question #1 : How To Divide Square Roots
Divide and simplify. Assume all integers are positive real numbers.
There are two ways to solve this problem. First you can divide the numbers under the radical. Then simplify.
Example 1
Example 2
Find the square root of both numerator and denominator, simplifying as much as possible then dividing out like terms.
Both methods will give you the correct answer of 
Example Question #1 : Basic Squaring / Square Roots
(√27 + √12) / √3 is equal to
(6√3)/√3
√3
5/√3
5
18
5
√27 is the same as 3√3, while √12 is the same as 2√3.
3√3 + 2√3 = 5√3
(5√3)/(√3) = 5
Example Question #1 : Basic Squaring / Square Roots
Simplify:
To simplfy, we must first distribute the square root.
Next, we can simplify each of the square roots.
Example Question #3 : Basic Squaring / Square Roots
Find the quotient:
Find the quotient:
There are two ways to approach this problem.
Option 1: Combine the radicals first, the reduce
Option 2: Simplify the radicals first, then reduce
Example Question #4 : Basic Squaring / Square Roots
Find the quotient:
Simplify each radical:
Rationalize the denominator:
Example Question #1 : How To Subtract Square Roots
Evaluate:
None of the available answers
Let us factor 108 and 81
Certified Tutor
All PSAT Math Resources
