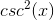Call Now to Set Up Tutoring:

(888) 888-0446

Precalculus : Pre-Calculus

Study concepts, example questions & explanations for Precalculus

Create An Account

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Limits

What is the

$\lim_{x \rightarrow 3}\frac{x^2-8x+15}{x^2-9}$ ?

Possible Answers:

$-\frac{5}{3}$

The limit does not exist

$-\frac{1}{3}$

Correct answer:

$-\frac{1}{3}$

Explanation:

We first need to simplify the function, we can do this by factoring the numerator and denominator.

$\frac{x^2-8x+15}{x^2-9}=\frac{(x-3)(x-5)}{(x-3)(x+3)}=\frac{(x-5)}{(x+3)}$

If we plug in 3 into the simplified function, we get:

$\frac{(3-5)}{(3+3)}=-\frac{2}{6}=-\frac{1}{3}$

Report an Error

Example Question #1 : Introductory Calculus

What is the

$\lim_{x \rightarrow 4}\frac{3x-5}{2x^2+10}$ ?

Possible Answers:

$-\frac{1}{2}$

$\frac{3}{2}$

$\frac{1}{6}$

Correct answer:

$\frac{1}{6}$

Explanation:

Substituting in for we get the following:

$\frac{3(4)-5}{2(4)^2+10}=\frac{12-5}{2(16)+10}=\frac{7}{32+10}$

$\frac{7}{42}=\frac{1}{6}$

Report an Error

Example Question #5 : Limits

Evaluate the following:

$\lim_{x\rightarrow\infty }\frac{6x^{4}-2x^{7}+8x^{5}}{2x^{2}-7x+3x^{5}}$

Possible Answers:

$+\infty$

limit does not exist

$-\infty$

$+\frac{8}{3}$

Correct answer:

$-\infty$

Explanation:

When evaluating limits at infinity there are three rules to keep in mind:

If the degree of the highest exponent in the numerator is equal to the degree of the highest exponent in the denominator, then the limit is equal to the ratio of the coefficient of the highest exponent in the numerator over the coefficient of the highest exponent in the denominator. Make sure to include signs.
If the degree of the highest exponent in the numerator is less than the degree of the highest exponent in the denominator, the limit = 0.
If the degree of the highest exponent in the numerator is greater than the degree of the higest exponent in the denominator, divide the highest power in the numerator by the highest power in the denominator and substitute for inifity. You will either subsitute for positive or negative infinity based on what the questions asks you to evaluate the limit at.

In this case, the degree is higher in the numerator than the denominator (rule #3). Hence, you need to divide the highest powers and evaluate.

$\frac{-x^{7}}{x^{5}}=-x^{2}$

Evaluate $\infty$ as x:

$-(\infty ^{2})=-\infty$

Answer: limit = $-\infty$

Report an Error

Example Question #6 : Limits

Evaluate the following:

$\lim_{x\rightarrow -\infty }\frac{6x^{3}+9x^{2}-12x^{4}}{3x^{4}-x^{2}+x}$

Possible Answers:

$+\infty$

$-\infty$

Correct answer:

Explanation:

When evaluating limits at infinity there are three rules to keep in mind:

If the degree of the highest exponent in the numerator is equal to the degree of the highest exponent in the denominator, then the limit is equal to the ratio of the coefficient of the highest exponent in the numerator over the coefficient of the highest exponent in the denominator. Make sure to include signs.
If the degree of the highest exponent in the numerator is less than the degree of the highest exponent in the denominator, the limit = 0.
If the degree of the highest exponent in the numerator is greater than the degree of the higest exponent in the denominator, divide the highest power in the numerator by the highest power in the denominator and substitute for inifity. You will either subsitute for positive or negative infinity based on what the questions asks you to evaluate the limit at.

In this case, the both the numerator and denominator have the highest degree of an exponent of 4 (rule #1). Hence, you need to compare the ratio of the coefficients.

$\frac{-12}{3}= -4$

Answer: limit =

Report an Error

Example Question #1 : Limits

What is the,

$\lim_{x\rightarrow \infty}\frac{2x^2}{\sqrt{4x^4+3x+10}}$ ?

Possible Answers:

$\infty$

$-\infty$

$\frac{1}{2}$

Correct answer:

Explanation:

The end behavior of the function $\frac{2x^2}{\sqrt{4x^4+3x+10}}$ follows the highest powers in both the numerator and denominator. Therefore, to find the limit we need to look only at the term: $\frac{2x^2}{\sqrt{4x^4}}$ as those are the highest powers in the numerator and demoninator.

Now we take the

$\lim_{x \rightarrow \infty}\frac{2x^2}{\sqrt{4x^4}}=\lim_{x \rightarrow \infty}\frac{2x^2}{2x^2}=1$ .

Thus the limit of our original function is also .

Report an Error

Example Question #1 : Trigonometric Identities

According to the trigonometric identities, 1+cot^2(x)=?

Possible Answers:

$\frac{sin(x)}{cos(x)}$

tan(x)

csc^2(x)

$\frac{1}{tan(x)}$

Correct answer:

csc^2(x)

Explanation:

The trigonometric identity 1+cot^2(x)=csc^2(x) , is an important identity to memorize.

Some other identities that are important to know are:

$\frac{sin(x)}{cos(x)}=tan(x)$

$cot(x)=\frac{1}{tan(x)}$

sin^2(x)+cos^2(x)=1

Report an Error

Example Question #1 : Sum And Difference Identities

Use the sum or difference identity to find the exact value: $sin165^\circ$

Possible Answers:

$\frac{1}{2}$

$\frac{\sqrt{6}-\sqrt{2}}{4}$

$\frac{\sqrt{2}-\sqrt{6}}{4}$

$\frac{\sqrt{6}+\sqrt{2}}{4}$

$\frac{\sqrt{6}}{4}$

Correct answer:

$\frac{\sqrt{6}-\sqrt{2}}{4}$

Explanation:

Again, here we break up the 165 into 135+30 and solve using the sin identity: $sin135\cdot cos30+cos135\cdot sin30\rightarrow \frac{\sqrt{2}}{2}\cdot \frac{\sqrt{3}}{2}+\frac{-\sqrt{2}}{2}\cdot \frac{1}{2}\rightarrow \frac{\sqrt{6}-\sqrt{2}}{4}$

Report an Error

Example Question #1 : Sum And Difference Identities

Is the following equation an identity? $sin(x-\pi)=sin(x)$

Possible Answers:

Yes it is an identity.

It cannot be determined from the given information.

No it is not an identity.

Correct answer:

No it is not an identity.

Explanation:

$sin(x-\pi)=sinx\rightarrow sinxcos\pi-cosxsin\pi=sinx\rightarrow sinx\cdot (-1)-cosx\cdot (0)\rightarrow -sinx-0=sinx\rightarrow -sinx\neq sinx$

and so this is again not an identity

Report an Error

Example Question #2 : Sum And Difference Identities

Is the following equation an identity? $\sin \left ( x-\pi \right )=\sin \left ( x \right )$ ?

Possible Answers:

No it is not an identity

The question cannot be answered based on the information provided.

Yes it is an identity

Correct answer:

No it is not an identity

Explanation:

$\sin \left ( x-\pi \right )=\sin x\rightarrow \sin x\cos \pi -\cos x\sin \pi = \sin x\rightarrow \sin x\cdot \left ( -1 \right )-\cos x\cdot \left ( 0 \right )\rightarrow -\sin x-0=\sin x\neq \sin x$

Therefore, this is not an identity.

Report an Error

Example Question #1 : Trigonometric Identities

Use the sum or difference identity to find the exact value: $cos255^\circ$

Possible Answers:

$\frac{1}{2}$

$\frac{2\sqrt{2}}{4}$

$\frac{\sqrt{2}-\sqrt{6}}{4}$

$\frac{-1}{2}$

$\frac{\sqrt{2}+\sqrt{6}}{4}$

Correct answer:

$\frac{\sqrt{2}-\sqrt{6}}{4}$

Explanation:

Using the identity, we can break up the 255 into and then solve: $cos300\cdot cos45+sin300\cdot sin45\rightarrow \frac{1}{2}\cdot \frac{\sqrt{2}}{2}+\frac{-\sqrt{3}}{2}\cdot \frac{\sqrt{2}}{2}\rightarrow \frac{\sqrt{2}-\sqrt{6}}{4}$

Report an Error

View Pre-Calculus Tutors

Cameo
Certified Tutor

University of Oklahoma Norman Campus, Bachelor of Science, Environmental Engineering.

View Pre-Calculus Tutors

Mayowa
Certified Tutor

Logan University, Bachelor of Science, Biology, General.

View Pre-Calculus Tutors

Ravi
Certified Tutor

Indian Institute of Technology - Mumbai, Bachelor of Technology, Mechanical Engineering. University of Cincinnati-Main Campus...

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

English Tutors in Atlanta, Algebra Tutors in San Diego, SSAT Tutors in Los Angeles, Biology Tutors in Miami, Biology Tutors in New York City, SSAT Tutors in Dallas Fort Worth, Physics Tutors in Miami, MCAT Tutors in Chicago, Calculus Tutors in Phoenix, English Tutors in New York City

Popular Courses & Classes

LSAT Courses & Classes in Philadelphia, SAT Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in Chicago, GMAT Courses & Classes in Atlanta, Spanish Courses & Classes in New York City, GRE Courses & Classes in New York City, GMAT Courses & Classes in Phoenix, SAT Courses & Classes in San Diego, ACT Courses & Classes in Atlanta, SSAT Courses & Classes in Atlanta

Popular Test Prep

GRE Test Prep in New York City, SAT Test Prep in Boston, SSAT Test Prep in Atlanta, SAT Test Prep in New York City, ISEE Test Prep in Seattle, SAT Test Prep in Los Angeles, ISEE Test Prep in Philadelphia, SAT Test Prep in San Francisco-Bay Area, MCAT Test Prep in Miami, GRE Test Prep in Denver

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Precalculus : Pre-Calculus

Study concepts, example questions & explanations for Precalculus

All Precalculus Resources

Example Questions

Example Question #1 : Limits

Example Question #1 : Introductory Calculus

Example Question #5 : Limits

Example Question #6 : Limits

Example Question #1 : Limits

Example Question #1 : Trigonometric Identities

Example Question #1 : Sum And Difference Identities

Example Question #1 : Sum And Difference Identities

Example Question #2 : Sum And Difference Identities

Example Question #1 : Trigonometric Identities

All Precalculus Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: