All Precalculus Resources
Example Questions
Example Question #2 : Rational Functions
Given the equation 
Change the equation into the y=x form.
When the degree of the exponent in the numerator is higher than the degree of the exponent in the denominator, there is no horizontal asymptote. Thus the answer is no horizontal asymptote.
Example Question #7 : Rational Functions
At what value(s) of 

Asymptotes can occur where the function is undefined, or when the denominator is equal to 

Now solving for when our denominator is zero gives us,
Therefore, there is a vertical asymptote at
Example Question #1 : Triangles
Let ABC be a right triangle with sides 






We are looking for 

So therefore, without figuring out 
Example Question #11 : Functions
Simplify the function below:
We need to use the following formulas:
a)
and
b)
We can simplify 
Example Question #1841 : High School Math
Simplify

Example Question #1842 : High School Math
Simplify

and

Example Question #12 : Functions
If 

1
We can write:
We can also write:
Now we can substitute the values of (sinx - cosx) and (sinx * cosx) in the obtained function:
Example Question #41 : Pre Calculus
Identifying a function.
Which of the following is a function.
The only relation listed that doesn't map more than one dependent variable value for some independent variable value is h(x). Another way of saying this is that each value in the domain of h corresponds to a distinct value in its range. h(x) is the only one-to-one relation, and so is the only option that is a function.
Example Question #16 : Functions
Evaluating a function.
Evaluate 

Plug in -3 for x in the function rule:

Example Question #17 : Functions
Finding inverse functions.
Given,
find

To find an inversefunction find the input in terms of the output. In other words, solve the function for the dependent variable.
First, set h(x) = y. Now solve for y.
Multiply the denominator on both sides
Distribute y
Rearrange to get only x terms on one side.
Factor out x on the left side.
Divide by (y-5) on both sides to get x by itself.
The final step is to recognize that the independent variable is now the dependent variable. To put this into proper notation switch x and y. The inverse function is

All Precalculus Resources



























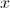



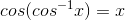







![[\cos(x)/\sin(x)]\times\sin(x)=\cos(x)](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/27160/gif.latex)











































