All Precalculus Resources
Example Questions
Example Question #821 : Pre Calculus
What is the solution to the following inequality?
First, we must solve for the roots of the cubic polynomial equation.
We obtain that the roots are 
Now there are four regions created by these numbers:
-
. In this region, the values of the polynomial are negative (i.e.plug in
and you obtain
-
. In this region, the values of the polynomial are positive (when
, polynomial evaluates to
)
-
. In this region the polynomial switches again to negative.
-
. In this region the values of the polynomial are positive
Hence the two regions we want are 

Example Question #2 : Solving Polynomial And Rational Inequalities
Solve the inequality.
First, subtract 

Then find the common denominator and simplify

Next, factor out the numerator

and set each of the three factor equal to zero and solve for 
The solutions are

Now plug in values between 
![\left(-1,\frac{1}{3}\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/462780/gif.latex)
![\left[\frac{1}{3},2\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/462781/gif.latex)

Note that 

![\left[\frac{1}{3},2\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/462782/gif.latex)
Thus, the solution to the inequality is
![(-\infty ,-1)\cup \left[\frac{1}{3},2\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/462783/gif.latex)
Example Question #3 : Solving Polynomial And Rational Inequalities
Solve and graph:
1) Multiply both sides of the equation by the common denominator of the fractions:
2) Simplify:
3) For standard notation, and the fact that inequalities can be read backwards:
For interval notation:

4) Graph:

Example Question #4 : Solving Polynomial And Rational Inequalities
Solve and graph:
Graph the rational expression,
1) Because 


2) As 









3) As 








4) The funtion y is exists over the allowed x-intervals:
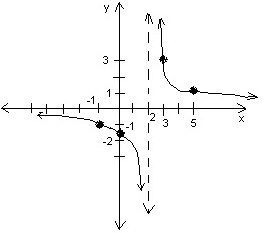
One approach for solving the inequality:
For
1) Determine where 


2) 


3) Then the solution is 
Another approach for solving the inequality:
1) Write 



2) 


3) Then the solution is 
Example Question #5 : Solving Polynomial And Rational Inequalities
Which of the following best describes the statement:
The undefined points of rational functions are vertical asymptotes.
The statement is always false
The statement is true in some cases and false in others
The statement is always true
The statement is always true
When solving for a point where the function will be undefined, you set the denominator equal to zero and solve for . This creates a vertical asymptote because when the denominator equals zero the function is undefined and we are solving for
. Say for example a function is undefined at
. So at all values of
where
this function is undefined creating a vertical asymptote.
Example Question #1 : Solve And Graph Rational Inequalities




We begin by finding the zeros of the equation using the numerator.
So we know that the function will equal zero when 


And so the function is undefined at 
And if we graph these points we see something like below (which is our answer). Note that the dotted blue line is the vertical asymptote at 

Example Question #2 : Solve And Graph Rational Inequalities
The zeros of the function are the values of 
We only need to solve for 
So the zeros of this function are 
To solve for the points at which this function will be undefined, we set the denominator equal to zero and solve for 
Example Question #1 : Solving Polynomial And Rational Inequalities
True or False: For the inequality 


True
False
False
This inequality wants all values where 


Example Question #9 : Solving Polynomial And Rational Inequalities
Which of the following is an accurate graph of the inequality 




We will first begin by solving for the zeros and undefined points of the inequality. We solve for the zeros by setting the numerator equal to zero.

And so the zeros of this function are at
Now we will solve for the undefined points by setting the denominator equal to zero. Since the denominator is 


For 






Certified Tutor
All Precalculus Resources









![\left(-\infty ,\frac{1}{3}\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/462779/gif.latex)


![\left(-1,\frac{1}{3}\right]\cup [2,\infty)](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/462778/gif.latex)
![(-\infty ,-1)\cup \left[\frac{1}{3},2\right]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/462777/gif.latex)
















![[-\infty, 2] \cup [5, +\infty ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/294387/gif.latex)
![[2, 5]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/477836/gif.latex)



























 , undefined:
, undefined: 




















