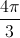All Precalculus Resources
Example Questions
Example Question #21 : Graphs And Inverses Of Trigonometric Functions
What is the reference angle for 
To find the reference angle, subtract 
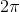
Example Question #3 : The Unit Circle And Radians
What is 
If you examine the unit circle, you'll see that that 
Example Question #33 : Trigonometry
What is 
If you examine the unit circle, you'll see that the value of 

Example Question #22 : Graphs And Inverses Of Trigonometric Functions
Which one of these is positive in quadrant III?
Cosine
Tangent
All trig functions
Sine
No trig functions
Tangent
The pattern for positive functions is All Student Take Calculus. In quandrant I, all trigonometric functions are positive. In quadrant II, sine is positive. In qudrant III, tangent is positive. In quadrant IV, cosine is positive.
Example Question #23 : Graphs And Inverses Of Trigonometric Functions
Find a coterminal angle for 
Coterminal angles are angles that, when drawn in the standard position, share a terminal side. You can find these angles by adding or subtracting 360 to the given angle. Thus, the only angle measurement that works from the answers given is 
Example Question #24 : Graphs And Inverses Of Trigonometric Functions
Which of the following angles is coterminal with 
Each angle given in the other choices is coterminal with 
Each angle given in the other choices is coterminal with 
For an angle to be coterminal with 







All four choices pass the test, so all four angles are coterminal with 
Example Question #1 : Trigonometry
What is 
To get rid of 


Example Question #2 : Trigonometry

In order to find 



Now to find the measure of the angle using the 
If you calculated the angle's measure to be 
Example Question #25 : Graphs And Inverses Of Trigonometric Functions
What is the amplitude of the following equation?
Based on the generic form 
Example Question #26 : Graphs And Inverses Of Trigonometric Functions
What is one possible length of side 



(Hint: There are two possible answers, but only one of them is listed.)
First we must set up our equation given the information.
Certified Tutor
Certified Tutor
All Precalculus Resources