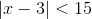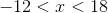Call Now to Set Up Tutoring:

(888) 888-0446

Precalculus : Functions

Study concepts, example questions & explanations for Precalculus

Create An Account

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Absolute Value Functions

Which of the following is a point on the following function?

$y=\left | x^2-5x-56\right |+27$

Possible Answers:

(14,36)

(-27,-27)

(0,0)

(5,83)

(5,-43)

Correct answer:

(5,83)

Explanation:

One way to approach this problem would be to plug in each answer and see what works. However, I would be a little more strategic and eliminate any options that don't make sense.

Our y value will never be negative, so eliminate any options with a negative y-value.

Try (0,0) really quick, since it's really easy

$y=\left | x^2-5x-56\right |+27 \rightarrow 0=\left|0^2-5(0)-56\right|+27=83 \rightarrow 0\neq 83$

The only point that makes sense is (5,83), therefore it is the correct answer

$y=\left | x^2-5x-56\right |+27 \rightarrow 83=\left|5^2-5(5)-56\right|+27=83 \rightarrow 83=83$

Report an Error

Example Question #3 : Special Functions

Evaluate: $\left | x-3\right |<15$

Possible Answers:

$x\leq18$

-12<x<18

-12,18

$-12\leq x\leq18$

x<18

Correct answer:

-12<x<18

Explanation:

Cancel the absolute value sign by separating the function $\left | x-3\right |<15$ into its positive and negative counterparts.

x-3<15

-(x-3)<15

Evaluate the first scenario.

x-3<15

x<18

Evaluate the second scenario.

-(x-3)<15

x-3>-15

x>-12

The correct answer is:

-12<x<18

Report an Error

Example Question #1 : Absolute Value Functions

If $y=\left |x^3-5x^2+5 \right |$ , then what is the value of when x=3 ?

Possible Answers:

-13

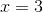
-7

Correct answer:

Explanation:

We evaluate for x=3

y=|(3)^3-5(3)^2+5|

y=|27-45+5|

y=|-13|

Since the absolute value of any number represents its magnitude from and is therefore always positive, the final answer would be y=13

Report an Error

Example Question #31 : Functions

Given f(x) and h(x) , evaluate f(h(7)) .

f(x)=5x^2-13x+8

h(x)=x^3-343

Possible Answers:

343

Correct answer:

Explanation:

We are given two functions and asked to find f(h(7)).

I would begin by finding h(7)

h(x)=x^3-343

h(7)=7^3-343=343-343=0

Now that we know h(7), we go ahead and plug it into f(x).

f(x)=5x^2-13x+8

$f(0)=5\cdot 0^2-13\cdot 0+8=8$

So our final answer is simply 8.

Report an Error

Example Question #32 : Functions

Solve the function. $\frac{10x^2-10}{x-1}=0$

Possible Answers:

$\pm \frac{1}{10}$

$\pm1$

Correct answer:

Explanation:

Determine the domain of $\frac{10x^2-10}{x-1}=0$ .

$x\neq1$

Multiply x-1 on both sides of the equation to cancel the denominator and divide the equation 10x^2-10=0 by ten.

x^2-1=0

x^2=1

$x= \pm1$

Since the domain states that $x\neq1$ , the only possible answer is x= -1 .

Report an Error

Example Question #3 : Evaluate Functions

Solve for : 9^x+1=4

Possible Answers:

$\frac{1}{4}$

$\frac{1}{3}$

$\frac{2}{3}$

$\frac{4}{9}$

$\frac{1}{2}$

Correct answer:

$\frac{1}{2}$

Explanation:

Rewrite 9^x+1=4 so that the exponential variable is isolated.

9^x=3

Reconvert 9^x=3 to a similar base. Use exponents to redefine the terms.

3^2^x=3^1

Since all terms are of the same base, use the property of log to eliminate the base on both sides of the equation.

2x=1

Solve for .

$x=\frac{1}{2}$

Report an Error

Example Question #1 : Evaluate Functions

Evaluate the following function when t=6

$f(t)=5^{3t-18}+5^t-t^2$

Possible Answers:

15590

15662

15589

Correct answer:

15590

Explanation:

Evaluate the following function when t=6

$f(t)=5^{3t-18}+5^t-t^2$

To evaluate this function, simply plug-in 6 for t and simplify:

$f(6)=5^{3(6)-18}+5^6-(6)^2=1+15625-36=15590$

So our answer is:

15590

Report an Error

Example Question #1 : Evaluate Functions

Determine the value of f(7) of the function

$f(x)=\sqrt{71-x}$

Possible Answers:

$f(7)=\sqrt{78}$

$f(7)\,\, \textsc{dne}$

f(7)=8

f(7)=10

Correct answer:

f(7)=8

Explanation:

In order to determine the value of f(7) of the function we set x=7. The value becomes

$f(7)=\sqrt{71-7}=\sqrt{64}=8$

As such

f(7)=8

Report an Error

Example Question #1 : Evaluate Functions

Find the difference quotient of the function f(x)=3x^2-2x-5 .

Possible Answers:

3h^2-2h-5

3(x+h)^2-2(x+h)-5

3h+6x-2

3h^2+3x^2-5

3h-3x-5

Correct answer:

3h+6x-2

Explanation:

Difference quotient equation is

$\frac{f(x+h)-f(x)}{h}$ and

f(x+h)=3(x+h)^2-2(x+h)-5 .

Now plug in the appropriate terms into the equation and simplify:

$\\ \frac{f(x+h)-f(x)}{h}\\ \\=\frac{3(x+h)^2-2(x+h)-5-(3x^2-2x-5)}{h}\\ \\=\frac{3(x^2+2xh+h^2)-2x-2h-5-3x^2+2x+5}{h}\\ \\=\frac{3x^2+6xh+3h^2-2x-2h-5-3x^2+2x+5}{h}\\ \\=\frac{6xh+3h^2-2h}{h}\\ \\=\frac{h(3h+6x-2)}{h}\\ \\=3h+6x-2$

Report an Error

Example Question #1 : Evaluate Functions

Find the value of the following function when x=2

$g(x)=\frac{2x^2-4x+5}{x^4-16}$

Possible Answers:

The function is undefined at x=2

Correct answer:

The function is undefined at x=2

Explanation:

Find the value of the following function when x=2

$g(x)=\frac{2x^2-4x+5}{x^4-16}$

To evaluate this function, plug in 2 for x everywhere it arises and simplify:

$g(x)=\frac{2(2)^2-4(2)+5}{(2)^4-16}=\frac{5}{0}=\mbox{undefined}$

So our answer must be undefined, because we cannot divide by

Report an Error

View Pre-Calculus Tutors

Jonathan
Certified Tutor

University of Maryland-Baltimore County, Bachelor of Science, Mathematics.

View Pre-Calculus Tutors

Joseph
Certified Tutor

Bank Street College of Education , Master's/Graduate, Early Childhood and Childhood General Education.

View Pre-Calculus Tutors

Andrew
Certified Tutor

University of Wisconsin-Stevens Point, Bachelor of Science, Mathematics. University of Wisconsin-Oshkosh, Master of Science, ...

All Precalculus Resources

12 Diagnostic Tests 380 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Spanish Tutors in Miami, Biology Tutors in Atlanta, Reading Tutors in Phoenix, Biology Tutors in New York City, French Tutors in Miami, GMAT Tutors in Miami, French Tutors in San Francisco-Bay Area, SSAT Tutors in Chicago, Physics Tutors in San Francisco-Bay Area, GRE Tutors in Phoenix

Popular Courses & Classes

ISEE Courses & Classes in Phoenix, GRE Courses & Classes in San Diego, MCAT Courses & Classes in Phoenix, ISEE Courses & Classes in Atlanta, LSAT Courses & Classes in Atlanta, SSAT Courses & Classes in Miami, ISEE Courses & Classes in Chicago, Spanish Courses & Classes in Atlanta, ACT Courses & Classes in Phoenix, Spanish Courses & Classes in Denver

Popular Test Prep

MCAT Test Prep in Miami, MCAT Test Prep in Philadelphia, SSAT Test Prep in Los Angeles, SSAT Test Prep in Miami, LSAT Test Prep in Philadelphia, GMAT Test Prep in Los Angeles, GMAT Test Prep in Atlanta, SSAT Test Prep in Seattle, GRE Test Prep in San Diego, LSAT Test Prep in Denver

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Precalculus : Functions

Study concepts, example questions & explanations for Precalculus

All Precalculus Resources

Example Questions

Example Question #1 : Absolute Value Functions

Example Question #3 : Special Functions

Example Question #1 : Absolute Value Functions

Example Question #31 : Functions

Example Question #32 : Functions

Example Question #3 : Evaluate Functions

Example Question #1 : Evaluate Functions

Example Question #1 : Evaluate Functions

Example Question #1 : Evaluate Functions

Example Question #1 : Evaluate Functions

All Precalculus Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: