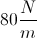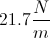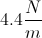MCAT Physical : Work, Energy, and Power
Study concepts, example questions & explanations for MCAT Physical
All MCAT Physical Resources
Example Questions
Example Question #2 : Kinetic Energy
Two children are playing with sleds on a snow-covered hill. Sam weighs 50kg, and his sled weighs 10kg. Sally weighs 40kg, and her sled weighs 12kg. When they arrive, they climb up the hill using boots. Halfway up the 50-meter hill, Sally slips and rolls back down to the bottom. Sam continues climbing, and eventually Sally joins him at the top.
They then decide to sled down the hill, but disagree about who will go first.
Scenario 1:
Sam goes down the hill first, claiming that he will reach a higher velocity. If Sally had gone first, Sam says they could collide.
Scenario 2:
Sally goes down the hill first, claiming that she will experience lower friction and thus reach a higher velocity. If Sam had gone first, Sally says they could collide.
Scenario 3:
Unable to agree, Sam and Sally tether themselves with a rope and go down together.
If the hill is frictionless, and after both Sally and Sam reach the bottom of the hill traveling at maximum velocity, who has lost more potential energy?
Sally, because she experiences less friction
Sally, because she is traveling faster
They have lost an equal amount of potential energy
Sam, because his mass is larger
Sam, because he is traveling more slowly
Sam, because his mass is larger
When they reach the bottom of the hill, Sam and Sally have both converted all of their potential energy into kinetic energy. We measured potential energy at 50m, so once they have lost 50m, the potential energy is 0, while the kinetic energy has reached maximum value. Because Sam has greater mass, he had more potential energy to convert to kinetic energy.
Example Question #1 : Kinetic Energy
Two children are playing with sleds on a snow-covered hill. Sam weighs 50kg, and his sled weighs 10kg. Sally weighs 40kg, and her sled weighs 12kg. When they arrive, they climb up the hill using boots. Halfway up the 50-meter hill, Sally slips and rolls back down to the bottom. Sam continues climbing, and eventually Sally joins him at the top.
They then decide to sled down the hill, but disagree about who will go first.
Scenario 1:
Sam goes down the hill first, claiming that he will reach a higher velocity. If Sally had gone first, Sam says they could collide.
Scenario 2:
Sally goes down the hill first, claiming that she will experience lower friction and thus reach a higher velocity. If Sam had gone first, Sally says they could collide.
Scenario 3:
Unable to agree, Sam and Sally tether themselves with a rope and go down together.
After reaching maximum velocity at the bottom of a frictionless hill identical to the one in the question, Sam deploys a parachute. How much energy must the parachute dissipate before Sam comes to a stop?
30000kJ
300kJ
300J
30J
30kJ
30kJ
The parachute must dissipate all of the kinetic energy that Sam has at the bottom of the hill, since there is no friction to slow him. He has 30kJ of energy, as per the equation PE = mgh.
PE = 60kg * 10m/s2 * 50m = 30,000J = KE at the bottom of the hill
Example Question #1 : Kinetic Energy
How much energy is required to a accelerate a 
From an energy stand-point, the block starts with zero kinetic energy and zero potential energy. At the end, the block still has zero potential energy, but has a non-zero kinetic energy. Assuming there is no friction, all energy added to the block have been converted to kinetic energy.
Using the change in velocity, we can solve for the energy used to move the block.
We are given our mass and the change in velocity, allowing us to solve for the change in kinetic energy.
Example Question #5 : Kinetic Energy
What is the kinetic energy of a 
Kinetic energy is given by the formula:
We are given the mass of the bullet and its velocity, allowing us to calculate kinetic energy from this formula.
Work is often expressed in Newton-meters, but energy is usually expressed as Joules, although the units are equivalent.
Example Question #6 : Kinetic Energy
Two children are playing on an icy lake. Child 1 weighs 50kg, and child 2 weighs 38kg. Child 1 has a backpack that weighs 10kg, and child 2 has a backpack that weighs 5kg.
Over the course of the afternoon, they collide many times. Four collisions are described below.
Collision 1:
Child 1 starts from the top of a ramp, and after going down, reaches the lake surface while going 
Collision 2:
Child 1 and child 2 are sliding in the same direction. Child 2, moving at 
Collision 3:
The two children collide while traveling in opposite directions at 
Collision 4:
The two children push off from one another’s back, and begin moving in exactly opposite directions. Child 2 moves with a velocity of 
In collision 1, imagine that child 2 was not present on the ice. How much energy would child 1 have to dissipate to the lake surface before he came to a stop? Ignore wind resistance.
The amount of kinetic energy that child 1 has after she reaches the lake surface is the amount of energy she will dissipate to the lake before coming to a complete stop.
Example Question #1 : Kinetic Energy
What is the kinetic energy of a 0.1kg projectile traveling at 
We can calculate the kinetic energy using the equation:
Use the given values for the mass and velocity to solve:
Example Question #1 : Potential Energy (Gravitational And Spring)
A spring in a pinball machine is compressed 
The energy in a spring is given by the equation 
The kinetic energy in the pinball is found using the equation 
Now that we have kinetic energy, we can find the equal energy that was stored in the spring to begin.
Example Question #2 : Potential Energy (Gravitational And Spring)
A 2kg mass is attached to a massless spring with force constant 100N/m. The system rests on a frictionless horizontal surface. If the spring is compressed 5cm, then released, what is the maximum velocity of the mass?
25cm/s
50cm/s
35.4cm/s
12.5cm/s
10.2cm/s
35.4cm/s
Use conservation of energy to compare the point of maximum compression and the point of maximum velocity. At maximum compression, all energy is spring potential energy, where 
Plugging in the given information for Es and solving for v gives the following series of calculations:
Example Question #3 : Potential Energy (Gravitational And Spring)
If a ball is attached to a spring that has a spring constant of 20N/m and is compressed 0.1m, what is the total energy of the oscillation?
The total energy of a simple harmonic oscillating system can be determined by the equation 
Example Question #221 : Mcat Physical Sciences
A massless spring rests horizontally on a table, with its right end attached to a 
Use conservation of energy to equate the initial spring potential energy and the final kinetic energy:
The initial situation is defined when the spring and block are compressed, and the final situation is defined to be when the block moves back through the equilibrium point. For a spring, the potential energy is maximal when the spring is compressed and the kinetic energy is maximal when the mass passes equilibrium. We can set up a equation based on these factors.
Use the equations for spring potential energy and for kinetic energy to incorporate the appropriate variables into the equation.
We are given values for the displacement, mass, and final velocity. Using these values, we can isolate the spring constant.
Certified Tutor
All MCAT Physical Resources
