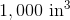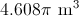Call Now to Set Up Tutoring:

(888) 888-0446

ISEE Upper Level Math : Solid Geometry

Study concepts, example questions & explanations for ISEE Upper Level Math

Create An Account

All ISEE Upper Level Math Resources

6 Diagnostic Tests 244 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #501 : Grade 8

The height of a cone and the radius of its base are equal. The circumference of the base is $10 \pi$ inches. Give its volume.

Possible Answers:

$\frac{5,000}{3} \pi \textrm{ in}^{3}$

$1,000 \textrm{ in}^{3}$

$\frac{125}{3} \pi \textrm{ in}^{3}$

$\frac{1,000}{3} \pi \textrm{ in}^{3}$

$125 \textrm{ in}^{3}$

Correct answer:

$\frac{125}{3} \pi \textrm{ in}^{3}$

Explanation:

A circle with circumference $10 \pi$ inches has as its radius

$r = \frac{C }{2\pi }=\frac{10\pi }{2\pi } = 5$ inches.

The height is also inches, so substitute h = r = 5 in the volume formula for a cone:

$V = \frac{1}{3} \pi r ^{2}h = \frac{1}{3} \pi \cdot 5 ^{2} \cdot 5 = \frac{125}{3} \pi$ cubic inches

Report an Error

Example Question #1 : How To Find The Volume Of A Cone

You are an architect designing a cone shaped structure. If the structure will be 30 ft tall and 10 feet wide at the base, what will the volume of the structure be?

Possible Answers:

$100 \pi ft^3$

$22.5 \pi ft^3$

250 ft^3

$250 \pi ft^3$

Correct answer:

$250 \pi ft^3$

Explanation:

You are an architect designing a cone shaped structure. If the structure will be 30 ft tall and 10 feet wide at the base, what will the volume of the structure be?

Begin by using the formula for volume of a cone:

$V=\pi r^2\frac{h}{3}$

Now, we simply need to plug in our knowns.

We know the height is 30 ft.

We know that the diameter is 10ft, however, we need the radius.

Divide 10 by 2 to get a radius of 5 ft.

Now, let's go....

$V=\pi (5ft)^2\frac{30ft}{3}=\pi25ft^2*10ft=250 \pi ft^3$

Report an Error

Example Question #3 : How To Find The Volume Of A Cone

Find the volume of a cone with the following measurements:

diameter = 12in
height = 6in

Possible Answers:

$72\pi \text{ in}^3$

$54\pi \text{ in}^3$

$36\pi \text{ in}^3$

$84\pi \text{ in}^3$

$48\pi \text{ in}^3$

Correct answer:

$72\pi \text{ in}^3$

Explanation:

To find the volume of a cone, we will use the following formula:

$V = \pi r^2 \frac{h}{3}$

where r is the radius and h is the height of the cone.

Now, we know the diameter of the cone is 12in. We also know the diameter is two times the radius. Therefore, the radius is 6in.

We know the height of the cone is 6in.

Knowing all of this, we can substitute into the formula. We get

$V = \pi \cdot (6\text{in})^2 \cdot \frac{6\text{in}}{3}$

$V = \pi \cdot 36\text{in}^2 \cdot 2\text{in}$

$V = \pi \cdot 72\text{in}^3$

$V = 72\pi \text{ in}^3$

Report an Error

Example Question #413 : Geometry

Find the volume of a cone with the following measurements:

Diameter: 14in
Height: 9in

Possible Answers:

$147\pi \text{ in}^3$

$441\pi \text{ in}^3$

$252\pi \text{ in}^3$

$63\pi \text{ in}^3$

$126\pi \text{ in}^3$

Correct answer:

$147\pi \text{ in}^3$

Explanation:

To find the volume of a cone, we will use the following formula:

$V = \pi r^2 \frac{h}{3}$

where r is the radius and h is the height of the cone.

Now, we know the diameter of the cone is 14in. We also know the diameter is two times the radius. Therefore, the radius is 7in.

We know the height of the cone is 9in.

Knowing all of this, we can substitute into the formula. We get

$V = \pi \cdot (7\text{in})^2 \cdot \frac{9\text{in}}{3}$

$V = \pi \cdot 49\text{in}^2 \cdot 3\text{in}$

$V = \pi \cdot 147\text{in}^3$

$V = 147\pi \text{ in}^3$

Report an Error

Example Question #414 : Geometry

Find the volume of a cone with the following measurements:

height: 12in
diameter: 6in

Possible Answers:

$24\pi \text{ in}^3$

$32\pi \text{ in}^3$

$36\pi \text{ in}^3$

$28\pi \text{ in}^3$

$48\pi \text{ in}^3$

Correct answer:

$36\pi \text{ in}^3$

Explanation:

To find the volume of a cone, we will use the following formula:

$V = \pi r^2 \frac{h}{3}$

where r is the radius and h is the height of the cone.

Now, we know the height of the cone is 12in. We also know the diameter of the cone is 6in. We know the diameter is two times the radius. Therefore, the radius is 3in.

So, we get

$V = \pi \cdot (3\text{in})^2 \cdot \frac{12\text{in}}{3}$

$V = \pi \cdot 9\text{in}^2 \cdot 4\text{in}$

$V = \pi \cdot 36\text{in}^3$

$V = 36\pi \text{ in}^3$

Report an Error

Example Question #101 : Solid Geometry

Find the volume of a cone with a base diameter of 2, and a height of 10.

Possible Answers:

$10\pi$

$\frac{10}{3}\pi$

$\frac{5}{3}\pi$

$\frac{40}{3}\pi$

$\frac{20}{3}\pi$

Correct answer:

$\frac{10}{3}\pi$

Explanation:

Write the formula to find the volume of a cone.

$V=\frac{1}{3}\pi r^2h$

The diameter is 2, which means the radius is 1. Substitute the known dimensions.

$V=\frac{1}{3}\pi (1)^2(10)$

The answer is: $\frac{10}{3}\pi$

Report an Error

Example Question #101 : Solid Geometry

A cone has height 240 centimeters; its base has radius 80 centimeters. Give its volume in cubic meters.

Possible Answers:

$0.768 \pi \textrm{ m}^{3}$

$1.536 \pi \textrm{ m}^{3}$

$0.512 \pi \textrm{ m}^{3}$

$4.608 \pi \textrm{ m}^{3}$

Correct answer:

$0.512 \pi \textrm{ m}^{3}$

Explanation:

Convert both dimensions from centimeters to meters by dividing by 100:

Height: 240 centimeters = $240 \div 100 = 2.4$ meters.

Radius: 80 centimeters = $80 \div 100 = 0.8$ meters.

Substitute r = 0.8, h = 2.4 in the volume formula:

$V = \frac{1}{3} \pi r^{2}h$

$V = \frac{1}{3} \cdot \pi \cdot 0.8 ^{2} \cdot 2.4$

$V = \frac{1}{3}\cdot 0.8 \cdot 0.8 \cdot 2.4 \cdot \pi$

$V =0.512 \pi$

Report an Error

Example Question #102 : Solid Geometry

What is the surface area of a cone with the following measurements?

r=4;d=8;h=10

Possible Answers:

$5+8\pi$

$5+64\pi$

$5+4\pi$

$5+16\pi$

Correct answer:

$5+16\pi$

Explanation:

The surface area of a cone can be found with the following equation:

$SA=\frac{1}{2}h+r^2\pi$

$SA=\frac{1}{2}(10)+4^2\pi=5+16\pi$

Report an Error

Example Question #1 : How To Find The Volume Of A Cylinder

The axle for a toy car has a length of 4 inches and a diameter of a quarter inch. What is the volume of the axle? Assume it is a cylinder.

Possible Answers:

$\frac{\pi}{4}in^3$

$16\pi in^3$

$\frac{\pi}{2}in^3$

$\frac{\pi}{16}in^3$

Correct answer:

$\frac{\pi}{16}in^3$

Explanation:

The axle for a toy car has a length of 4 inches and a diameter of a quarter inch. What is the volume of the axle? Assume it is a cylinder.

Use the following formula for volume of a cylinder

$V_{cylinder}=\pi r^2h$

Where r and h are our radius and height, respectively.

In this case, we first need to change our diameter to radius. Because our diameter is one quarter of an inch, our radius will be one eighth of an inch.

Plug it in to get:

$V_{cylinder}=\pi (\frac{1}{8})^2*4=\pi*\frac{1}{64}in^2*4in=\frac{4\pi}{64}in^3$

Simplify to get:

$\frac{4\pi}{64}in^3=\frac{\pi}{16}in^3$

Report an Error

Example Question #2 : Cylinders

You are visiting the drive-through at the bank. You put you money in a cylindrical tube with a height of 8 inches and a radius of 2 inches. What is the volume of the tube?

Possible Answers:

Not enough information

$16\pi in^3$

$64\pi in^3$

$32\pi in^3$

Correct answer:

$32\pi in^3$

Explanation:

You are visiting the drive-through at the bank. You put you money in a cylindrical tube with a height of 8 inches and a radius of 2 inches. What is the volume of the tube?

Begin with the formula for volume of a cylinder:

$V_{cylinder}=\pi r^2h$

We have r and h, which are our radius and height, respectively. Plug them in and solve

$V_{cylinder}=\pi (2in)^2*8in=\pi*32in^2$

Making our answer:

$32\pi in^2$

Report an Error

View Tutors

Joseph
Certified Tutor

Bank Street College of Education , Master's/Graduate, Early Childhood and Childhood General Education.

View Tutors

Gregory
Certified Tutor

Pennsylvania State University-Main Campus, Bachelor of Science, Chemical Engineering. Carnegie Mellon University, Doctor of P...

View Tutors

Robert
Certified Tutor

University of North Carolina at Chapel Hill, Bachelor in Arts, English. North Carolina Central University, Master of Arts, En...

All ISEE Upper Level Math Resources

6 Diagnostic Tests 244 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Physics Tutors in Phoenix, LSAT Tutors in Boston, ACT Tutors in Miami, Physics Tutors in Miami, Spanish Tutors in Los Angeles, Statistics Tutors in Denver, ISEE Tutors in San Francisco-Bay Area, Statistics Tutors in Boston, Biology Tutors in Phoenix, MCAT Tutors in Boston

Popular Courses & Classes

ACT Courses & Classes in Dallas Fort Worth, SAT Courses & Classes in Boston, Spanish Courses & Classes in San Francisco-Bay Area, MCAT Courses & Classes in Miami, GRE Courses & Classes in Washington DC, MCAT Courses & Classes in Phoenix, Spanish Courses & Classes in Miami, GMAT Courses & Classes in Atlanta, SAT Courses & Classes in San Diego, SAT Courses & Classes in Denver

Popular Test Prep

ISEE Test Prep in Seattle, SAT Test Prep in Miami, SAT Test Prep in Boston, MCAT Test Prep in Atlanta, GMAT Test Prep in Denver, SAT Test Prep in Dallas Fort Worth, LSAT Test Prep in Boston, MCAT Test Prep in Seattle, MCAT Test Prep in Philadelphia, ACT Test Prep in Boston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

ISEE Upper Level Math : Solid Geometry

Study concepts, example questions & explanations for ISEE Upper Level Math

All ISEE Upper Level Math Resources

Example Questions

Example Question #501 : Grade 8

Example Question #1 : How To Find The Volume Of A Cone

Example Question #3 : How To Find The Volume Of A Cone

Example Question #413 : Geometry

Example Question #414 : Geometry

Example Question #101 : Solid Geometry

Example Question #101 : Solid Geometry

Example Question #102 : Solid Geometry

Example Question #1 : How To Find The Volume Of A Cylinder

Example Question #2 : Cylinders

All ISEE Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: