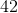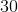All ISEE Upper Level Math Resources
Example Questions
Example Question #131 : Data Analysis And Probability
Over the course of seven games, Joanna scored the following number of points for her high school's varsity basketball team:

What is the range of the number of points she scored?
In order to find the range, you must first identify the smallest and the largest number of the set.
The smallest number of the set is 

Take the difference between these two to find the range.
Example Question #132 : Data Analysis And Probability
Use the following data set to answer the question:
Find the range.
To find the range of a data set, we will find the smallest number and the largest number. Then, we will find the difference of those two numbers.
So, given the set
we can see the smallest number is 3 and the largest number is 9. Now, we will find the difference.
Therefore, the range of the data set is 6.
Example Question #133 : Data Analysis And Probability
Use the following data set to answer the question:
Find the range.
To find the range of a data set, we will find the smallest number and the largest number. Then, we will find the difference of those two numbers.
So, given the set
we can see the smallest number is 2 and the largest number is 9. Now, we will find the difference.
Therefore, the range of the data set is 7.
Example Question #21 : How To Find Range
Determine the range:
The range is the difference between the largest and smallest numbers.
The largest number is:
The smallest number is:
Subtract both quantities.
The answer is:
Example Question #131 : Data Analysis And Probability
Define set 


For 




However, 


Example Question #131 : Data Analysis
Given a set 


None of the choices answer the question correctly.



For 






Also, 


This leaves 
If 

This is the correct choice.
Example Question #3 : How To Find The Missing Part Of A List
Examine the sequence:
What number goes into the circle?
Each element is obtained by adding a number to the previous one; the number added increases by 1 each time:


Example Question #4 : How To Find The Missing Part Of A List
A pair of fair dice are tossed. What is the probability that the product of the numbers of the faces is greater than or equal to ?
Out of a possible thirty-six rolls, the following result in a product of twenty or greater:
This is eight out of thirty-six, making the probability

Example Question #1 : How To Find The Missing Part Of A List
Ten students are running for student council; each member of the student body will choose four.
Two of the candidates are Kevin's brothers, Mickey and Steve. Kevin wants to vote for one, but not both, of his brothers. How many ways can Kevin fill out his ballot so that he can vote for exactly one of his brothers?
Kevin will choose three students from the eight who are not his brothers, and will do so without respect to order. This is the number of combinations of three from eight:
Kevin will also choose one of his two brothers. By the multiplication principle, Kevin has 
Example Question #6 : How To Find The Missing Part Of A List
Define
What is the natural domain of 
The only possible restriction of the domain here is the denominator 

This has no real solution, so the domain is the set of all real numbers, 
Certified Tutor
Certified Tutor
All ISEE Upper Level Math Resources






















![[-1,-3,-5,21,-21]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/920850/gif.latex)