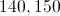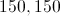All Intermediate Geometry Resources
Example Questions
Example Question #82 : Equilateral Triangles
An equilateral triangle is placed together with a semicircle as shown by the figure below.

Find the perimeter of the figure.

In order to find the perimeter of the entire figure, we will need to find the lengths of the segments highlighted in red.
Notice that the side length of the equilateral triangle is equal to the diameter of the semicircle.
Next, you should recall that the height of an equilateral triangle splits the triangle into two congruent 
Recall that the side lengths in a 


We can then set up the following to determine the length of the side of the equilateral triangle:
Rearrange the equation to solve for the length of the side.
Plug in the length of the height to find the length of the side.
Since the diameter of the semi-circle and the length of a side of the equilateral triangle are the same, we can write the following equation:
We have two sides of the equilateral triangle and the circumference of a semi-circle.
Plug in the length of the side to find the perimeter.
Example Question #11 : How To Find The Perimeter Of An Equilateral Triangle
An equilateral triangle is placed together with a semicircle as shown by the figure below.

Find the perimeter of the figure.

In order to find the perimeter of the entire figure, we will need to find the lengths of the segments highlighted in red.
Notice that the side length of the equilateral triangle is equal to the diameter of the semicircle.
Next, you should recall that the height of an equilateral triangle splits the triangle into two congruent 
Recall that the side lengths in a 


We can then set up the following to determine the length of the side of the equilateral triangle:
Rearrange the equation to solve for the length of the side.
Plug in the length of the height to find the length of the side.
Since the diameter of the semi-circle and the length of a side of the equilateral triangle are the same, we can write the following equation:
We have two sides of the equilateral triangle and the circumference of a semi-circle.
Plug in the length of the side to find the perimeter.
Example Question #84 : Equilateral Triangles
An equilateral triangle is placed together with a semicircle as shown by the figure below.

Find the perimeter of the figure.

In order to find the perimeter of the entire figure, we will need to find the lengths of the segments highlighted in red.
Notice that the side length of the equilateral triangle is equal to the diameter of the semicircle.
Next, you should recall that the height of an equilateral triangle splits the triangle into two congruent 
Recall that the side lengths in a 


We can then set up the following to determine the length of the side of the equilateral triangle:
Rearrange the equation to solve for the length of the side.
Plug in the length of the height to find the length of the side.
Since the diameter of the semi-circle and the length of a side of the equilateral triangle are the same, we can write the following equation:
We have two sides of the equilateral triangle and the circumference of a semi-circle.
Plug in the length of the side to find the perimeter.
Example Question #91 : Equilateral Triangles
An equilateral triangle is placed together with a semicircle as shown by the figure below.

Find the perimeter of the figure.

In order to find the perimeter of the entire figure, we will need to find the lengths of the segments highlighted in red.
Notice that the side length of the equilateral triangle is equal to the diameter of the semicircle.
Next, you should recall that the height of an equilateral triangle splits the triangle into two congruent 
Recall that the side lengths in a 


We can then set up the following to determine the length of the side of the equilateral triangle:
Rearrange the equation to solve for the length of the side.
Plug in the length of the height to find the length of the side.
Since the diameter of the semi-circle and the length of a side of the equilateral triangle are the same, we can write the following equation:
We have two sides of the equilateral triangle and the circumference of a semi-circle.
Plug in the length of the side to find the perimeter.
Example Question #91 : Equilateral Triangles
An equilateral triangle is placed together with a semicircle as shown by the figure below.

Find the perimeter of the figure.

In order to find the perimeter of the entire figure, we will need to find the lengths of the segments highlighted in red.
Notice that the side length of the equilateral triangle is equal to the diameter of the semicircle.
Next, you should recall that the height of an equilateral triangle splits the triangle into two congruent 
Recall that the side lengths in a 


We can then set up the following to determine the length of the side of the equilateral triangle:
Rearrange the equation to solve for the length of the side.
Plug in the length of the height to find the length of the side.
Since the diameter of the semi-circle and the length of a side of the equilateral triangle are the same, we can write the following equation:
We have two sides of the equilateral triangle and the circumference of a semi-circle.
Plug in the length of the side to find the perimeter.
Example Question #93 : Equilateral Triangles
An equilateral triangle is placed together with a semicircle as shown by the figure below.

Find the perimeter of the figure.

In order to find the perimeter of the entire figure, we will need to find the lengths of the segments highlighted in red.
Notice that the side length of the equilateral triangle is equal to the diameter of the semicircle.
Next, you should recall that the height of an equilateral triangle splits the triangle into two congruent 
Recall that the side lengths in a 


We can then set up the following to determine the length of the side of the equilateral triangle:
Rearrange the equation to solve for the length of the side.
Plug in the length of the height to find the length of the side.
Since the diameter of the semi-circle and the length of a side of the equilateral triangle are the same, we can write the following equation:
We have two sides of the equilateral triangle and the circumference of a semi-circle.
Plug in the length of the side to find the perimeter.
Example Question #94 : Equilateral Triangles
If an equilateral triangle has a height of 

Since the triangle is equilateral, all sides are the same length. Therefore, we only need to find the length of one side to determine the perimeter. We can do this by means of the Pythagorean Theorem. In the attached figure, the equilateral triangle has been divided into two right triangles, for which the Pythagorean Theorem can be performed:

With 

Now that we know the length of one side, we can solve for the total perimeter by summing like sides:
Example Question #731 : Plane Geometry
Given: 

True or false: The perimeter of 
True
False
False
The perimeter of an equilateral triangle - one with three sides of equal length - is equal to three times the length of one side. Therefore, 
One yard is equal to 36 inches, making the statement false.
Example Question #291 : Triangles
Locate 




Give the perimeter of the triangle.
A midsegment of a triangle - a segment whose endpoints are the midpoints of two sides - is, by the Triangle Midsegment Theorem, parallel to the third side, and is half the length of that side. Therefore,
Substitute and solve:
Since the lengths of the sides of an equilateral triangle are equal, the perimeter is three times this, or
Example Question #1 : Pentagons
The angles at 3 verticies of a pentagon are 60, 80 and 100. Which of the following could NOT be the measures of the other 2 angles?
The sum of the angles in a polygon is
For a pentagon, this equals 540. Since the first 3 angles add up to 240, the remaining 2 angles must add up to
All Intermediate Geometry Resources