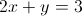Call Now to Set Up Tutoring:

(888) 888-0446

Intermediate Geometry : Intermediate Geometry

Study concepts, example questions & explanations for Intermediate Geometry

Create An Account

All Intermediate Geometry Resources

8 Diagnostic Tests 250 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #11 : How To Find The Slope Of A Line

Find the slope of the line that passes through the points:

(92, 14) and (100, 20)

Possible Answers:

$-\frac{1}{4}$

$\frac{3}{4}$

$\frac{1}{2}$

Correct answer:

$\frac{3}{4}$

Explanation:

Recall that the slope of a line also measures how steep the line is. Use the following equation to find the slope of the line:

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}$

Now, substitute in the information using the given points.

$\text{Slope}=\frac{20-14}{100-92}$

Simplify.

$\text{Slope}=\frac{6}{8}$

Solve.

$\text{Slope}=\frac{3}{4}$

Report an Error

Example Question #1432 : Intermediate Geometry

Find the slope of the line that passes through the points:

(-10, -14) and (34, 12)

Possible Answers:

$\frac{1}{2}$

$\frac{13}{22}$

$\frac{5}{11}$

$-\frac{7}{11}$

Correct answer:

$\frac{13}{22}$

Explanation:

Recall that the slope of a line also measures how steep the line is. Use the following equation to find the slope of the line:

$\text{Slope}=\frac{y_2-y_1}{x_2-x_1}$

Now, substitute in the information using the given points.

$\text{Slope}=\frac{12-(-14)}{34-(-10)}$

Simplify.

$\text{Slope}=\frac{26}{44}$

Solve.

$\text{Slope}=\frac{13}{22}$

Report an Error

Example Question #11 : Graph Proportional Relationships, Interpreting The Unit Rate As The Slope: Ccss.Math.Content.8.Ee.B.5

What is the slope m of the line below?

y - 2= 2(x-4)

Possible Answers:

m = 2

m=4

m=-2

m=-4

m=-8

Correct answer:

m = 2

Explanation:

To answer this question you should realize that the equation for the line is given in point - slope form. The standard point slope form of a line is given below:

y-y_1 = m(x-x_1)

m represents the slope of the line so all we have to do is recognize the correct line form and we automatically know that the slope is m = 2

Report an Error

Example Question #1433 : Intermediate Geometry

Give the slope of the line of the equation 8y = 1

Possible Answers:

$\textup{The slope is undefined}$

$\frac{1}{8}$

Correct answer:

Explanation:

If we divide both sides by 8, as follows:

8y = 1

$\frac{8y}{8} = \frac{1}{8}$

$y = \frac{1}{8}$

we see that the equation is equivalent to one of the form y = b . This is a horizontal line, and its slope is 0.

Report an Error

Example Question #11 : How To Find The Slope Of A Line

Give the slope of the line of the equation $- \frac{2}{3}x = 4$ .

Possible Answers:

$\textup{The slope is undefined}$

$- \frac{3}{2}$

$- \frac{2}{3}$

Correct answer:

$\textup{The slope is undefined}$

Explanation:

If we multiply both sides of the equation by $-\frac{3}{2}$ , as follows:

$- \frac{2}{3}x = 4$

$- \frac{3}{2} \cdot \left (- \frac{2}{3}x \right )=- \frac{3}{2} \cdot 4$

x = 6

we see that the equation is equivalent to one of the form x = a . This is a vertical line, which has undefined slope.

Report an Error

Example Question #1 : How To Find The Equation Of A Perpendicular Line

What line is perpendicular to $y=\frac{-1}{2}x-3$ through (1,5) ?

Possible Answers:

x+3y=1

x-y=5

2x+y=3

3x-y=4

2x-y = -3

Correct answer:

2x-y = -3

Explanation:

$y=\frac{-1}{2}x-3$ is given in the slope-intercept form. So the slope is $\frac{-1}{2}$ and the y-intercept is .

If the lines are perpendicular, then $m_{1}\cdot m_{2}=-1$ so the new slope must be

Next we substitute the new slope and the given point into the slope-intercept form of the equation to calculate the intercept. So the equation to solve becomes 5=2(1) +b so b=3

So the equation of the perpendicular line becomes y=2x+3 or in standard form 2x-y=-3

Report an Error

Example Question #1 : How To Find The Equation Of A Perpendicular Line

Which line below is perpendicular to 5x+6y=18 ?

Possible Answers:

$y=\frac{5}{6}x+\frac{6}{5}$

$y=-\frac{5}{6}x+\frac{6}{5}$

$y=\frac{5}{6}x+2$

$y=-\frac{6}{5}x+8$

$y = \frac{6}{5}x + 3$

Correct answer:

$y = \frac{6}{5}x + 3$

Explanation:

The definition of a perpendicular line is one that has a negative, reciprocal slope to another.

For this particular problem, we must first manipulate our initial equation into a more easily recognizable and useful form: slope-intercept form or y=mx+b .

5x+6y=18

6y=-5x+18

$y=-\frac{5}{6}x+6$

According to our y=mx+b formula, our slope for the original line is $-\frac{5}{6}$ . We are looking for an answer that has a perpendicular slope, or an opposite reciprocal. The opposite reciprocal of $-\frac{5}{6}$ is $\frac{6}{5}$ . Flip the original and multiply it by .

Our answer will have a slope of $\frac{6}{5}$ . Search the answer choices for $\frac{6}{5}$ in the position of the y=mx+b equation.

$\dpi{100} y = \frac{6}{5}x + 3$ is our answer.

(As an aside, the negative reciprocal of 4 is $-\frac{1}{4}$ . Place the whole number over one and then flip/negate. This does not apply to the above problem, but should be understood to tackle certain permutations of this problem type where the original slope is an integer.)

Report an Error

Example Question #1 : How To Find The Equation Of A Perpendicular Line

Find the equation of the line perpendicular to $y=\frac{-1}{3}x+2$ .

Possible Answers:

$y=\frac{1}{3}x+2$

y=-3x+2

y=3x+2

y=3x-2

y=-3x-2

Correct answer:

y=3x+2

Explanation:

The definition of a perpendicular line is a line with a negative reciprocal slope and identical intercept.

Therefore we need a line with slope 3 and intercept 2.

This means the only fitting line is y=3x+2 .

Report an Error

Example Question #2 : Perpendicular Lines

Which one of these equations is perpendicular to:

y=x+2

Possible Answers:

y=-x^2

y=-2x+5

y=2

y=-x+1

y=x

Correct answer:

y=-x+1

Explanation:

To find the perpendicular line to

y=x+2

we need to find the negative reciprocal of the slope of the above equation.

So the slope of the above equation is since changes by when is incremented.

The negative reciprocal is:

$reciprocal=\frac{-1}{slope}$

$reciprocal=\frac{1}{-1}$

reciprocal=-1

So we are looking for an equation with a .

Only y=-x+1 satisfies this condition.

Report an Error

Example Question #2 : How To Find The Equation Of A Perpendicular Line

Suppose a line is represented by a function f(x) = 2x+4 . Find the equation of a perpendicular line that intersects the point (1,2) .

Possible Answers:

f(x)=2x

$f(x)= -\frac{1}{2}x+1\frac{1}{2}$

$f(x)= -\frac{1}{2}x$

$f(x)= \frac{1}{2}x-1\frac{1}{2}$

$f(x)= -\frac{1}{2}x+2\frac{1}{2}$

Correct answer:

$f(x)= -\frac{1}{2}x+2\frac{1}{2}$

Explanation:

Determine the slope of the function f(x) = 2x+4 . The slope is: m1=2

The slope of a perpendicular line is the negative reciprocal of the original slope. Determine the value of the slope perpendicular to the original function.

$m2 = - \frac{1}{m1} = -\frac{1}{2}$

Plug in the given point and the slope to the slope-intercept form to find the y-intercept.

y=mx+b

$2=\left(-\frac{1}{2}\right)(1)+b$

$b=2\frac{1}{2}$

Substitute the slope of the perpendicular line and the new y-intercept back in the slope-intercept equation, y=mx+b .

The correct answer is: $f(x)= -\frac{1}{2}x+2\frac{1}{2}$

Report an Error

View Tutors

Linda
Certified Tutor

Pomona College, Bachelor of Science, Political Science and Government.

View Tutors

Tracy
Certified Tutor

Clarion University of Pennsylvania, Bachelor of Science, Physics.

View Tutors

Chukwudi
Certified Tutor

University of Nigeria, Nsukka., Bachelor of Science, Biochemistry. African University of Science and Technology., Master of S...

All Intermediate Geometry Resources

8 Diagnostic Tests 250 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

GRE Tutors in Seattle, LSAT Tutors in San Diego, Computer Science Tutors in Seattle, Statistics Tutors in Houston, Spanish Tutors in Philadelphia, ISEE Tutors in Denver, GRE Tutors in Miami, Biology Tutors in San Diego, Computer Science Tutors in Phoenix, GMAT Tutors in Chicago

Popular Courses & Classes

SAT Courses & Classes in New York City, GRE Courses & Classes in Miami, MCAT Courses & Classes in Boston, SSAT Courses & Classes in Washington DC, ISEE Courses & Classes in Miami, MCAT Courses & Classes in Houston, LSAT Courses & Classes in Boston, ACT Courses & Classes in Miami, GRE Courses & Classes in San Francisco-Bay Area, GMAT Courses & Classes in Denver

Popular Test Prep

SAT Test Prep in Miami, MCAT Test Prep in Boston, MCAT Test Prep in Houston, ACT Test Prep in Washington DC, MCAT Test Prep in Denver, LSAT Test Prep in Miami, ACT Test Prep in Chicago, GRE Test Prep in Los Angeles, ACT Test Prep in Miami, MCAT Test Prep in New York City

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Intermediate Geometry : Intermediate Geometry

Study concepts, example questions & explanations for Intermediate Geometry

All Intermediate Geometry Resources

Example Questions

Example Question #11 : How To Find The Slope Of A Line

Example Question #1432 : Intermediate Geometry

Example Question #11 : Graph Proportional Relationships, Interpreting The Unit Rate As The Slope: Ccss.Math.Content.8.Ee.B.5

Example Question #1433 : Intermediate Geometry

Example Question #11 : How To Find The Slope Of A Line

Example Question #1 : How To Find The Equation Of A Perpendicular Line

Example Question #1 : How To Find The Equation Of A Perpendicular Line

Example Question #1 : How To Find The Equation Of A Perpendicular Line

Example Question #2 : Perpendicular Lines

Example Question #2 : How To Find The Equation Of A Perpendicular Line

All Intermediate Geometry Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: