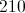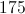Call Now to Set Up Tutoring:

(888) 888-0446

HSPT Math : Concepts

Study concepts, example questions & explanations for HSPT Math

Create An Account

All HSPT Math Resources

6 Diagnostic Tests 130 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #236 : Geometric Measurement: Understanding Concepts Of Area And Relating Area To Multiplication And To Addition

If the formula to find the area of the white side of the rectangle below is $9\times2$ , what is the formula to find that area of the purple side?

Screen shot 2015 11 04 at 3.51.11 pm

Possible Answers:

$9\times6$

$9\times8$

$8\times9$

$6\times9$

$9\times7$

Correct answer:

$9\times7$

Explanation:

Both the purple side and the white side have the same length, $9\ units$ . The width of the purple side is $7\ units$ . Thus the area formula for the purple side of the rectangle is $9\times7$ .

Report an Error

Example Question #1291 : Concepts

If the formula to find the area of the white side of the rectangle below is $9\times4$ , what is the formula to find that area of the purple side?

Screen shot 2015 11 04 at 3.56.07 pm

Possible Answers:

$8\times7$

$9\times7$

$9\times6$

$9\times5$

$8\times6$

Correct answer:

$9\times5$

Explanation:

Both the purple side and the white side have the same length, $9\ units$ . The width of the purple side is $5\ units$ . Thus the area formula for the purple side of the rectangle is $9\times5$ .

Report an Error

Example Question #1771 : Common Core Math: Grade 3

If the formula to find the area of the white side of the rectangle below is $9\times5$ , what is the formula to find that area of the purple side?

Screen shot 2015 11 04 at 4.41.07 pm

Possible Answers:

$9\times5$

$8\times4$

$9\times4$

$8\times5$

$9\times6$

Correct answer:

$9\times4$

Explanation:

Both the purple side and the white side have the same length, $9\ units$ . The width of the purple side is $4\ units$ . Thus the area formula for the purple side of the rectangle is $9\times4$ .

Report an Error

Example Question #240 : Geometric Measurement: Understanding Concepts Of Area And Relating Area To Multiplication And To Addition

If the formula to find the area of the white side of the rectangle below is $9\times7$ , what is the formula to find that area of the purple side?

Screen shot 2015 11 04 at 4.45.34 pm

Possible Answers:

$9\times2$

$7\times3$

$9\times3$

$7\times2$

$9\times9$

Correct answer:

$9\times2$

Explanation:

Both the purple side and the white side have the same length, $9\ units$ . The width of the purple side is $2\ units$ . Thus the area formula for the purple side of the rectangle is $9\times2$ .

Report an Error

Example Question #3 : How To Find The Area Of A Rectangle

What is the area of the figure below?

Possible Answers:

47in^2

24in^2

35in^2

59in^2

52in^2

Correct answer:

59in^2

Explanation:

To find the area of the figure above, we need to split the figure into two rectangles.

12.5

Using our area formula, $A=l\times w$ , we can solve for the area of both of our rectangles

$A=7\times 5$ $A=6\times 4$

A=35in^2 A=24in^2

To find our final answer, we need to add the areas together.

35in^2+24in^2=59in^2

Report an Error

Example Question #237 : Geometric Measurement: Understanding Concepts Of Area And Relating Area To Multiplication And To Addition

If the formula to find the area of the white section of the rectangle below is $9\times3$ , what is the formula to find that area of the purple section?

Screen shot 2015 11 04 at 3.54.29 pm

Possible Answers:

$9\times8$

$9\times7$

$7\times8$

$9\times6$

Correct answer:

$9\times6$

Explanation:

Both the purple section and the white section have the same length, $9\ units$ . The width of the purple section is $6\ units$ . Thus the area formula for the purple section of the rectangle is $9\times6$ .

Report an Error

Example Question #1291 : Concepts

What is the perimeter of a figure with seven sides, four of which measure 9 inches, two of which measure 10 inches, and one of which measures a foot?

Possible Answers:

$31 \; in$

$68 \; in$

$20 \; in$

$57 \; in$

$58 \; in$

Correct answer:

$68 \; in$

Explanation:

Add the side measures, in inches (changing one foot to 12 inches):

9+9+9+9+10+10+12= 68

Report an Error

Example Question #1 : Perimeter Of A Triangle

What is the perimeter of a right triangle with hypotenuse and a leg of length ?

Possible Answers:

It cannot be determined from the information given.

210

175

126

119

Correct answer:

210

Explanation:

Using the Pythagorean Theorem, the length of the second leg can be determined.

a^2+b^2=c^2

We are given the length of the hypotenuse and one leg.

a=35, c=91

$b^2=c^2-a^2\rightarrow b=\sqrt{c^2-a^2}$

$\sqrt{91^{2}-35^{2}} = \sqrt{8,281-1,225} = \sqrt{7,056} = 84$

The perimeter of the triangle is the sum of the lengths of the sides.

P=35+84+91 = 210

Report an Error

Example Question #2 : Properties Of Triangles

Which of these polygons has the same perimeter as a right triangle with legs 6 feet and 8 feet?

Possible Answers:

A regular hexagon with sidelength one yard.

A regular octagon with sidelength one yard.

None of the other responses is correct.

A regular decagon with sidelength one yard.

A regular pentagon with sidelength one yard.

Correct answer:

A regular octagon with sidelength one yard.

Explanation:

A right triangle with legs 6 feet and 8 feet has hypotentuse 10 feet, as this is a right triangle that confirms to the well-known Pythagorean triple 6-8-10. The perimeter is therefore 6 + 8 + 10 = 24 feet, or 8 yards.

We are looking for a polygon with this perimeter. Each choice is a polygon with all sides one yard long, so we want the polygon with eight sides - the regular octagon is the correct choice.

Report an Error

Example Question #1292 : Concepts

The perimeter of the following trapezoid is equal to 23 cm. Solve for . (Figure not drawn to scale.)

Possible Answers:

x=4

x=12

x=8

x=2

Correct answer:

x=4

Explanation:

The perimeter is equal to the sum of all of the sides.

$P=23\: cm$

$P=23\: cm=5+6+x+2x$

23=11+3x

23-11=11+3x-11

12=3x

$\frac{12}{3}=\frac{3x}{3}$

x=4

Report an Error

View Tutors

Usman
Certified Tutor

New York University, Bachelor in Arts, Political Science and Government.

View Tutors

Kevin
Certified Tutor

St. Johns University, Bachelors, Math/Coaching.

View Tutors

Andrew
Certified Tutor

University of South Florida-Main Campus, Bachelors, Biomedical Sciences. New York Medical College, PHD, Doctor of Medicine.

All HSPT Math Resources

6 Diagnostic Tests 130 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

SSAT Tutors in Philadelphia, ISEE Tutors in Phoenix, English Tutors in Dallas Fort Worth, Spanish Tutors in Phoenix, French Tutors in Los Angeles, SAT Tutors in Boston, Statistics Tutors in Dallas Fort Worth, Reading Tutors in Philadelphia, English Tutors in Houston, Computer Science Tutors in Chicago

Popular Courses & Classes

ISEE Courses & Classes in Los Angeles, MCAT Courses & Classes in San Diego, SSAT Courses & Classes in Miami, SSAT Courses & Classes in Dallas Fort Worth, Spanish Courses & Classes in Atlanta, GRE Courses & Classes in Boston, SSAT Courses & Classes in New York City, GRE Courses & Classes in Denver, ISEE Courses & Classes in San Francisco-Bay Area, LSAT Courses & Classes in Boston

Popular Test Prep

SAT Test Prep in Houston, ACT Test Prep in Seattle, ISEE Test Prep in Dallas Fort Worth, GRE Test Prep in Boston, MCAT Test Prep in Houston, ISEE Test Prep in Seattle, ACT Test Prep in Dallas Fort Worth, ISEE Test Prep in New York City, GRE Test Prep in Denver, ISEE Test Prep in Los Angeles

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

HSPT Math : Concepts

Study concepts, example questions & explanations for HSPT Math

All HSPT Math Resources

Example Questions

Example Question #236 : Geometric Measurement: Understanding Concepts Of Area And Relating Area To Multiplication And To Addition

Example Question #1291 : Concepts

Example Question #1771 : Common Core Math: Grade 3

Example Question #240 : Geometric Measurement: Understanding Concepts Of Area And Relating Area To Multiplication And To Addition

Example Question #3 : How To Find The Area Of A Rectangle

Example Question #237 : Geometric Measurement: Understanding Concepts Of Area And Relating Area To Multiplication And To Addition

Example Question #1291 : Concepts

Example Question #1 : Perimeter Of A Triangle

Example Question #2 : Properties Of Triangles

Example Question #1292 : Concepts

All HSPT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: