To find the ratio we must know the equation for the circumference of a circle. In this equation, is the circumference and is the diameter.

Once we know the equation, we can solve for the ratio of the diameter to circumference by solving the equation for . We do this by dividing both sides by .

Then we divide both sides by the circumference.

We now know that the ratio of the diameter to circumference is equal to .

To find the ratio we must know the equation for the circumference of a circle is

Once we know the equation we can solve for the ratio of the diameter to circumference by solving the equation for 

we divide both sides by the circumference giving us 

We now know that the ratio of the diameter to circumference is equal to .

The formula for the area of a circle is , and the formula for circumference is . If we solve for C in terms of r, we get
.

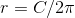
We can then substitute this value of r into the formula for the area:

The circumference of any circle is 

So the ratio of its circumference to its radius r, is

The distance between each notch on the clock is 6 degrees because there are 360 degrees on the clock, and there are 60 notches total. The minute hand is at notch #20, and so it is 120 degrees from the top. The hour hand is a little past notch #40 because the time is a little past hour 8. Thus, the hour hand is a little past 240 degrees from the top, going clockwise ( degrees).

In each hour, the hour hand goes 5 notches, or 30 degrees.  Because it is now 20 minutes past the hour, a third of an hour has passed.  One third of 30 degrees is 10 degrees.  Thus, the hour hand is 10 degrees past notch #40.  The hour hand is 250 degrees from the top, going clockwise.  The angle between the two hands is thus 130 degrees.  

This is a proportion problem

  so there are  in  percent of a circle

First note that a clock is a circle made of 360 degrees, and that each number represents an angle and the separation between them is 360/12 = 30. And at 2:00, the minute hand is on the 12 and the hour hand is on the 2.  The correct answer is 2 * 30 = 60 degrees.

The interior angle of a sector is equal the the angle of the sector. If the entire circumference was the sector, it would equal . Additionally, if it were 12:00, the angle would be equal to . We can solve the problem by setting up a proportion. 2:00 will be two-twelfths of the circle past the 12:00 mark, and will be at an angle of .

Cross multiply and solve for .

At four o’clock the minute hand is on the 12 and the hour hand is on the 4.  The angle formed is 4/12 of the total number of degrees in a circle, 360.

4/12 * 360 = 120 degrees

A clock is a circle, and a circle always contains 360 degrees. Since there are 60 minutes on a clock, each minute mark is 6 degrees.

The minute hand on the clock will point at 15 minutes, allowing us to calculate it's position on the circle.

Since there are 12 hours on the clock, each hour mark is 30 degrees.

We can calculate where the hour hand will be at 8:00.

However, the hour hand will actually be between the 8 and the 9, since we are looking at 8:15 rather than an absolute hour mark. 15 minutes is equal to one-fourth of an hour. Use the same equation to find the additional position of the hour hand.

We are looking for the angle between the two hands of the clock. The will be equal to the difference between the two angle measures.