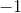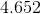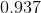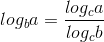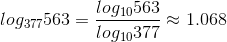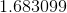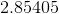\(\displaystyle We\ can\ use\ the\ general\ rule:\ log_{b}(a)=x\\ when\ rewritten\ in\ exponential\ form\ we\ get: b^{x}=a\)

\(\displaystyle In\ this\ case\ we\ have\ log_{3}(x)=2\)

\(\displaystyle This\ can\ be\ rewritten\ in\ exponential\ form\ as: 3^2=x\)

\(\displaystyle We\ know\ that\ 3^2=9;\ so\ x=9\)

\(\displaystyle When\ condensing\ logs\ we\ must\ remember\ the\ log\ properties:\)

\(\displaystyle log(x)+log(y)=log(xy): When\ adding;\ we\ multiply.\)

\(\displaystyle log(x)-log(y)=log\left (\frac{x}{y} \right ): When\ subtracting;\ we\ divide.\)

\(\displaystyle GIVEN: Rewrite\ as\ one\ log:\ log(a)-(\left log(b)+log(c) \right)\\\)

\(\displaystyle This\ is\ rewritten\ as:\ log \left (\frac{a}{bc} \right )\\ because\ the\ negative\ will\ be\ distributed\ to\ log(b)\ and\ log(c)\)

Recall a few properties of logarithms:

1.When adding logarithms of like base, we multiply the inside.

2.When subtracting logarithms of like base, we divide the inside.

3. When multiplying a logarithm by a number, we can raise the inside to that power.

So we begin with this:

\(\displaystyle 5log_35x+log_3\frac{1}{4x^3}-log_3x^2\)

I would start with 3 to simplify the first log.

\(\displaystyle log_3(5x)^5+log_3\frac{1}{4x^3}-log_3x^2\)

\(\displaystyle log_33125x^5+log_3\frac{1}{4x^3}-log_3x^2\)

Next, use rule 1 on the first two logs.

\(\displaystyle log_3\left(3125x^5\cdot\frac{1}{4x^3}\right)-log_3x^2\)

\(\displaystyle log_3\left(\frac{3125x^2}{4}\right)-log_3x^2\)

Then, use rule 2 to combine these two.

\(\displaystyle log_3\left(\frac{3125x^2}{4x^2}\right)=log_3\left(\frac{3125}{4}\right)\approx 6.06\)

So our answer is 6.06.

In order to evaluate the unknown variable, it is necessary to change the base. Looking at the right side of the equation, 27 is equivalent to three cubed.

\(\displaystyle 3^3=27\)

Therefore, converting the right side of the equation to a base of 3 will allow setting both the left and right side of the exponential terms equal to each other.

\(\displaystyle 3^{3x+3}=27^{2x+2}\)

\(\displaystyle 3^{3x+3}=3^{3(2x+2)}\)

Log both sides to drop the exponents by log properties, and divide the log based 3 on both sides to cancel this term.  

Solve for x.

\(\displaystyle ln\: 3^(^3^x^+^3^)=ln\:3^3^(^2^x^+^2^)\)

\(\displaystyle 3x+3=3(2x+2)\)

\(\displaystyle 3x+3=6x+6\)

\(\displaystyle -3=3x\)

\(\displaystyle x=-1\)

The simplest way to evaluate a logarithm that doesn't have base 10 is with change of base formula:

\(\displaystyle log_ba=\frac{log_ca}{log_cb}\)

So we have

\(\displaystyle log_{377}(563)\)

\(\displaystyle log_{377}563=\frac{log_{10}563}{log_{10}377}\approx1.068\)

In order to solve a logarithm, we must first rewrite it in log form: 

\(\displaystyle 3^x=23\ written\ in\ log\ form\ is: log_{3}(23)=x\)

To solve for x, we must use the Change of Base: 

\(\displaystyle log_{3}(23)=\frac{log(23)}{log(3)}\)

\(\displaystyle \frac{log(23)}{log(3)}=2.85405\)

This means that: 

\(\displaystyle 3^{2.85405}=23\)

We begin with the following:

\(\displaystyle 343=7^{15t-12}\)

This can be rewritten as

\(\displaystyle 7^3=7^{15t-12}\)

Recall that if you have two exponents with equal bases, you can simply set the exponents equal to eachother. Do so to get the following:

\(\displaystyle 3=15t-12\)

Solve this to get t.

\(\displaystyle 15=15t\)

\(\displaystyle t=1\)

We need to make the bases equal before attempting to solve for \(\displaystyle x\). Since \(\displaystyle 16=4^2\) we can rewrite our equation as

\(\displaystyle 4^{2x}=4^{2^{2x-6}}\)

    Remember: the exponent rule \(\displaystyle (x^n)^m=x^{n\cdot m}\)

\(\displaystyle 4^{2x}=4^{2(2x-6)}\)

Now that our bases are equal, we can set the exponents equal to each other and solve for \(\displaystyle x\). 

\(\displaystyle 2x=2(2x-6)\)

\(\displaystyle 2x=4x-12\)

\(\displaystyle 12=2x\)

\(\displaystyle x=6\)

The first step is to make sure we don't have a zero on one side which we can easily take care of: 

\(\displaystyle 7^{2y}=3^{y+2}\)

Now we can take the logarithm of both sides using natural log:

\(\displaystyle \ln 7^{2y}=\ln 3^{y+2}\)

Note: we can apply the Power Rule here \(\displaystyle \ln (x^y)=y\ln (x)\)

\(\displaystyle 2y \ln 7=(y+2)\ln3\)

\(\displaystyle 2y \ln 7=y\ln3+2\ln3\)

\(\displaystyle 2y \ln 7-y\ln3=2\ln3\)

\(\displaystyle y(2 \ln 7-\ln3)=2\ln3\)

\(\displaystyle y=\frac{2\ln3}{2 \ln 7-\ln3}\)

Before beginning to solve for \(\displaystyle x\), we need \(\displaystyle e\) to have a coefficient of \(\displaystyle 1\): 

\(\displaystyle e^{(4x-5)}=8\)

Now we can take the natural log of both sides:

\(\displaystyle \ln e^{(4x-5)}=\ln8\)

Note: \(\displaystyle \ln (e)=1\)

\(\displaystyle 4x-5=\ln8\)

\(\displaystyle 4x=\ln8+5\)

\(\displaystyle x=\frac{\ln8+5}{4}\)