All GED Math Resources
Example Questions
Example Question #231 : 2 Dimensional Geometry
Which of the following can be the measures of the three angles of an acute isosceles triangle?
For the triangle to be acute, all three angles must measure less than 


In an isosceles triangle, at least two angles are congruent, so we can eliminate 
The degree measures of the three angles of a triangle must total 180, so, since 


Example Question #51 : Triangles

Note: Figure NOT drawn to scale.
Refer to the above triangle. Evaluate 
The degree measures of a triangle total 
Example Question #59 : Triangles

Note: Figure NOT drawn to scale.
Refer to the above figure. Evaluate 
The degree measures of the interior angles of a triangle total 

Three angles with measures 
Example Question #2 : Angles And Triangles
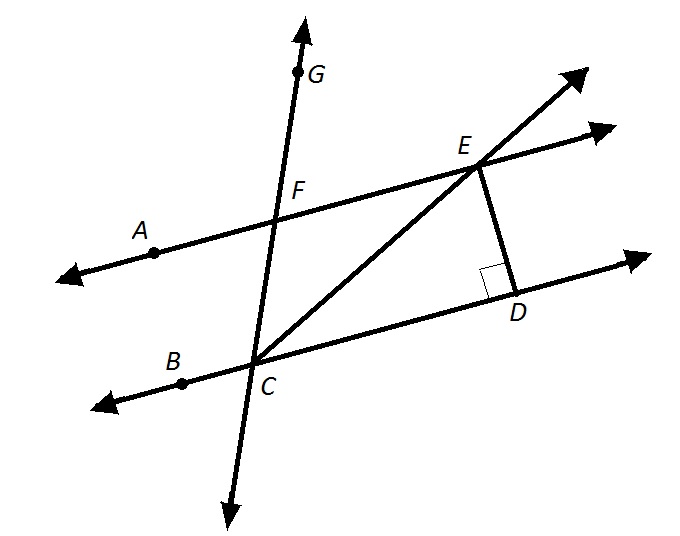
Figure drawn to scale.
Refer to the above diagram.
Which of the following is a valid description of 
Equiangular
Obtuse
Right
Acute
Obtuse
One of the angles of 


Example Question #3 : Angles And Triangles

Refer to the above diagram.
Which of the following is a valid description of 
Equiangular
Right
Acute
Obtuse
Right
One of the angles of 


Example Question #61 : Triangles
Which of the following follows from the fact that 
A congruency statement about two triangles implies nothing about the relationship between two angles of one of the triangles, so 
Also, letters in the same position between the two triangles refer to corresponding - and subsequently, congruent - angles. Therefore, 
Of the given choices, only 
Example Question #5 : Angles And Triangles
An exterior angle of an isosceles triangle measures 
Insufficient information is given to answer this question.
Insufficient information is given to answer this question.
The triangle has an exterior angle of 

Case 1: Two angles have measure 
![\left [180 - (50 + 50 ) \right ] ^{\circ } = 80 ^{\circ }](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/222438/gif.latex)

Case 2: One angle has measure 


The given information is therefore inconclusive.
Example Question #6 : Angles And Triangles
An exterior angle of an isosceles triangle measures 
Insufficient information is given to answer this question
The triangle has an exterior angle of 

Case 1: Two angles have measure 
![\left [180 - (50 + 50 ) \right ] ^{\circ } = 80 ^{\circ }](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/222425/gif.latex)

Case 2: One angle has measure 


In both cases, the least of the degree measures of the angles will be 
Example Question #8 : Angles And Triangles
An exterior angle of an isosceles triangle measures 
Insufficient information is given to answer this question.
The triangle has an exterior angle of 

Since this is an obtuse angle, its other two angles must be acute. By the Isosceles Triangle Theorem, an isosceles triangle must have two congruent angles - the acute angles are those. Since the sum of their measures is the same as their remote exterior angle - 

Example Question #241 : 2 Dimensional Geometry
An exterior angle of an isosceles triangle measures 
Insufficient information is given to answer this question.
The triangle has an exterior angle of 

Since this is an obtuse angle, its other two angles must be acute. Therefore, this angle is the one of greatest measure.
All GED Math Resources



















































