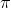All Common Core: High School - Functions Resources
Example Questions
Example Question #5 : Symmetry And Periodicity Of Trigonometric Functions: Ccss.Math.Content.Hsf.Tf.B.4
Find the following trigonometric exact value.
This question tests one's ability to recognize and use the unit circle to calculate the exact trigonometric value.
For the purpose of Common Core Standards, "Use the unit circle to explain symmetry (odd and even) and periodicity of trigonometric functions." falls within the Cluster A of "Extend the domain of trigonometric functions using the unit circle" concept (CCSS.MATH.CONTENT.HSF-TF.A.4).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Recall the unit circle.

Step 2: Identify the 

Step 3: Calculate the exact value of 
Recall that,

therefore,

Example Question #6 : Symmetry And Periodicity Of Trigonometric Functions: Ccss.Math.Content.Hsf.Tf.B.4
Find the following trigonometric exact value.
This question tests one's ability to recognize and use the unit circle to calculate the exact trigonometric value.
For the purpose of Common Core Standards, "Use the unit circle to explain symmetry (odd and even) and periodicity of trigonometric functions." falls within the Cluster A of "Extend the domain of trigonometric functions using the unit circle" concept (CCSS.MATH.CONTENT.HSF-TF.A.4).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Recall the unit circle.

Step 2: Identify the 

Step 3: Calculate the exact value of 
Recall that,

therefore,

Example Question #7 : Symmetry And Periodicity Of Trigonometric Functions: Ccss.Math.Content.Hsf.Tf.B.4
Find the following trigonometric exact value.
This question tests one's ability to recognize and use the unit circle to calculate the exact trigonometric value.
For the purpose of Common Core Standards, "Use the unit circle to explain symmetry (odd and even) and periodicity of trigonometric functions." falls within the Cluster A of "Extend the domain of trigonometric functions using the unit circle" concept (CCSS.MATH.CONTENT.HSF-TF.A.4).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Recall the unit circle.

Step 2: Identify the 

Step 3: Calculate the exact value of 
Recall that,

therefore,

Example Question #8 : Symmetry And Periodicity Of Trigonometric Functions: Ccss.Math.Content.Hsf.Tf.B.4
Find the following trigonometric exact value.
This question tests one's ability to recognize and use the unit circle to calculate the exact trigonometric value.
For the purpose of Common Core Standards, "Use the unit circle to explain symmetry (odd and even) and periodicity of trigonometric functions." falls within the Cluster A of "Extend the domain of trigonometric functions using the unit circle" concept (CCSS.MATH.CONTENT.HSF-TF.A.4).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Recall the unit circle.

Step 2: Identify the 

Step 3: Calculate the exact value of 
Recall that,

therefore,

Example Question #9 : Symmetry And Periodicity Of Trigonometric Functions: Ccss.Math.Content.Hsf.Tf.B.4
Find the following trigonometric exact value.
This question tests one's ability to recognize and use the unit circle to calculate the exact trigonometric value.
For the purpose of Common Core Standards, "Use the unit circle to explain symmetry (odd and even) and periodicity of trigonometric functions." falls within the Cluster A of "Extend the domain of trigonometric functions using the unit circle" concept (CCSS.MATH.CONTENT.HSF-TF.A.4).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Recall the unit circle.

Step 2: Identify the 

Step 3: Calculate the exact value of 
Recall that,

therefore,

Example Question #10 : Symmetry And Periodicity Of Trigonometric Functions: Ccss.Math.Content.Hsf.Tf.B.4
Find the following trigonometric exact value.
This question tests one's ability to recognize and use the unit circle to calculate the exact trigonometric value.
For the purpose of Common Core Standards, "Use the unit circle to explain symmetry (odd and even) and periodicity of trigonometric functions." falls within the Cluster A of "Extend the domain of trigonometric functions using the unit circle" concept (CCSS.MATH.CONTENT.HSF-TF.A.4).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Recall the unit circle.

Step 2: Identify the 

Step 3: Calculate the exact value of 
Recall that,

therefore,

Example Question #11 : Symmetry And Periodicity Of Trigonometric Functions: Ccss.Math.Content.Hsf.Tf.B.4
Find the following trigonometric exact value.
This question tests one's ability to recognize and use the unit circle to calculate the exact trigonometric value.
For the purpose of Common Core Standards, "Use the unit circle to explain symmetry (odd and even) and periodicity of trigonometric functions." falls within the Cluster A of "Extend the domain of trigonometric functions using the unit circle" concept (CCSS.MATH.CONTENT.HSF-TF.A.4).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Recall the unit circle.

Step 2: Identify the 

Step 3: Calculate the exact value of 
Recall that,

therefore,

Example Question #42 : Trigonometric Functions
Find the following trigonometric exact value.
This question tests one's ability to recognize and use the unit circle to calculate the exact trigonometric value.
For the purpose of Common Core Standards, "Use the unit circle to explain symmetry (odd and even) and periodicity of trigonometric functions." falls within the Cluster A of "Extend the domain of trigonometric functions using the unit circle" concept (CCSS.MATH.CONTENT.HSF-TF.A.4).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Recall the unit circle.

Step 2: Identify the 

Step 3: Calculate the exact value of 
Recall that,

therefore,

Example Question #1 : Model Periodicity, Amplitude, Frequency, And Midline Of Trigonometric Functions: Ccss.Math.Content.Hsf Tf.B.5
What is the period of the following function?
This question is testing one's ability to identify the periodicity of a trigonometric function. This requires the understanding of trigonometric functions and their graphical and algebraic characteristics.
For the purpose of Common Core Standards, "Choose trigonometric functions to model periodic phenomena with specified amplitude, frequency, and midline" falls within the Cluster B of "Model Periodic Phenomena with Trigonometric Functions" concept (CCSS.MATH.CONTENT.HSF-TF.B.5).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Write the general form of trigonometric shifts.
where
Step 2: Algebraically identify the period.
Given the function
identify the variables of the general form.

Step 3: Graph the trigonometric function to verify.

The graph above verifies that the period of this function is 


Example Question #2 : Model Periodicity, Amplitude, Frequency, And Midline Of Trigonometric Functions: Ccss.Math.Content.Hsf Tf.B.5
What is the amplitude of the following function?
This question is testing one's ability to identify the periodicity of a trigonometric function. This requires the understanding of trigonometric functions and their graphical and algebraic characteristics.
For the purpose of Common Core Standards, "Choose trigonometric functions to model periodic phenomena with specified amplitude, frequency, and midline" falls within the Cluster B of "Model Periodic Phenomena with Trigonometric Functions" concept (CCSS.MATH.CONTENT.HSF-TF.B.5).
Knowing the standard and the concept for which it relates to, we can now do the step-by-step process to solve the problem in question.
Step 1: Write the general form of trigonometric shifts.
where
Step 2: Algebraically identify the amplitude.
Given the function
identify the variables of the general form.

Step 3: Graph the trigonometric function to verify.

The graph above verifies that the amplitude of this function is two because the range of the function on the graph goes from negative two to positive two meaning the distance from zero at its highest peak or lowest valley is two.
Certified Tutor
Certified Tutor
All Common Core: High School - Functions Resources