All College Chemistry Resources
Example Questions
Example Question #1 : Buffers
Which of the following acid and base pairs are capable of acting as a buffer?





In this question, we're presented with a variety of acid/base pairs and we're asked to identify which one could act as a buffer.
Remember that a buffer is a pair of acid and its conjugate base that acts to resist substantial changes in pH. In order for a buffer to work, the acid base pair needs to exist in equilibrium. This way, when the pH of the solution changes, the equilibrium of the acid/base reaction will shift, such that the pH will not change drastically.
To have an acid/base pair in equilibrium, we'll need to look for a pair that contains a weak acid. Acids like 



Example Question #2 : Acid Base Reactions
You are in chemistry lab performing a titration. You were given 15 mL of an aqueous solution with an unknown concentration of acetic acid, 


The endpoint was determined at 10 mL of sodium hydroxide, 

4.75
8.45
9.25
7
4.75
At the half end point, the 
Since the endpoint of the titration is that there are 10 mL of 0.1 M NaOH added, that means that there are 0.001 moles of acetic acid.
When 5 mL of NaOH is added, there are 0.0005 moles of acetic acid and 0.0005 moles of acetate formed.

Therefore pH= pKa
Example Question #1 : Acid Base Reactions
Determine which combination of solutions would create a buffer solution.
5 mL of 0.4 M NaOH + 10 mL of 0.2 M ammonium
25 mL of 0.4 M NaOH + 50 mL of 0.2 M ammonium
50 mL of 0.4 M NaOH + 50 mL of 0.8 M ammonium
10 mL of 0.4 M NaOH + 10 mL of 0.2 M ammonium
50 mL of 0.4 M NaOH + 50 mL of 0.8 M ammonium
For all the other options there is no ammonium leftover with which to serve as the weak acid in the buffer system, the ammonium is all used up and converted to ammonia. However in the correct answer choice, there is enough ammonium leftover after the reaction with the sodium hydroxide.
Example Question #1 : Buffers
Determine the pH of an aqueous solution of 0.01 M acetic acid, 
2.87
7
0.161
3.45
2.87
Since acetic acid is a weak acid, it has a Ka that is rather small, we have to do a RICE table to determine the equilibrium amount of hydronium, H3O+ to then determine the pH.
R
I 0.1 M - 0 0
C -x +x +x
E 0.1 -x x x
So first we need to change our pKa to a Ka
where 


![\frac{[CH_{}3COO^{}-][H_{}3O^{}+]}{[CH_{}3COOH]}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/1006241/gif.latex)
If we assume that x is very small compared to 0.1...
Where
(note: when solving using the quadratic we come up with the same answer)
So if
Example Question #2 : Acid Base Reactions
Determine which solution(s) will yield a buffer solution.
I. 10 mL of 0.5 M HCl + 20 mL of 0.5 M acetate
II. 10 mL of 0.5 M HCl + 10 mL of 0.5 M acetate
III. 10 mL of 0.5 M HCl + 10 mL of 1.0 M acetate
IV. 10 mL of 0.5 M HCl + 10 mL of 1.5 M acetate
I and III
I, III, and IV
III and IV
I only
I, II, III, and IV
I, III, and IV
These answers are correct because the two components needed to create a buffer solution are a weak acid and its conjugate base, or a weak base and its conjugate acid. In these cases, the first reaction to occur upon addition of the strong acid is the formation of the conjugate acid, acetic acid.
If the amount of initial 

Example Question #2 : Buffers
Which combination(s) would create a buffer solution?
I. Weak acid
II. Weak acid's conjugate base
III. Strong acid
IV. Strong base
V. Weak base
VI. Weak base's conjugate acid
I and II; V and VI
None of these will combine to form a buffer solution
I and II; III and IV; V and VI
III and IV only
I and II; V and VI
A buffer solution is formed from the equilibrium of a weak acid and its conjugate base, or from a weak base and its conjugate acid. It's ability to "buffer" the pH or keep it from changing in large amounts in from the switching between these two forms weak and its conjugate.
Example Question #1 : Buffers
Determine which of these solution combinations form a buffer.
10 mL of 0.5 M NaOH + 10 mL of 0.5 M ammonium
10 mL of 0.5 M NaOH + 10 mL of 0.5 M ammonia
10 mL of 0.5 M NaOH + 20 mL of 0.5 M ammonium
10 mL of 0.5 M NaOH + 10 mL of 0.5 M HCl
10 mL of 0.5 M NaOH + 20 mL of 0.5 M ammonium
First to go through why the other ones are wrong:
Strong base + strong acid neutralizes and does not form a buffer solution
Strong base + weak base does not form a buffer - would need an acid
Strong base + weak acid = all weak acid converted to conjugate base
The correct answer is:
Strong base + weak acid = half converted to conjugate base with half leftover as weak acid, with all the components for a buffer
Example Question #1 : Titrations
The titration of a 




Start by writing the chemical equation for this acid-base reaction.
Next, find the number of moles of 
At the equivalence point of a titration, the moles of acid and the moles of base are the same.
Finally, find the molarity of the solution.
Make sure to round the answer to three significant figures.
Example Question #9 : Acid Base Reactions
A 



Start by finding the initial number of moles of sodium hydroxide by using the given volume and molarity. Since sodium hydroxide is a strong base, it will dissociate completely into 
Next, find the number of moles of the hydrochloric acid added by using the given volume and molarity. Since hydrochloric acid is a strong acid, all of the 
Now, as the acid is added, it will neutralize some of the base as shown by the following equation:
Since the reactants are found in a 
Now, divide this number of moles by the total volume of the solution to find the concentration of the base.
Next, find the 
Finally, find the 
Your answer should have 
Example Question #1 : Acid Base Reactions
![]()
Determine the volume in mL of 





Determine the moles of 
At the equivalence point of the titration:
Convert the moles of 
Convert the liters to milliliters:
All College Chemistry Resources

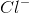
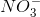







![x= 0.00133 = [H_{}3O^{}+]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/1006245/gif.latex)
![pH = -log [H_{}3O^{}+] =-log(0.0013) = 2.87](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/1006246/gif.latex)

















![[\text{OH}]^-=\frac{7.5\times 10^{-4}\text{ moles}}{0.075\text{L}+0.045\text{L}}=0.00625\text{ M}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/902579/gif.latex)















