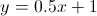We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Calculus 1 : Equation of Line

Study concepts, example questions & explanations for Calculus 1

Create An Account

All Calculus 1 Resources

10 Diagnostic Tests 438 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #1 : Equation Of Line

What is the equation of the line tangent to f(x) = 4x³ – 2x² + 4 at x = 5?

Possible Answers:

None of the other answers

y = 85x + 24

y = 280x – 946

y = 44x + 245

y = 220x – 550

Correct answer:

y = 280x – 946

Explanation:

First, take the derivative of f(x). This is very easy:

f'(x) = 12x² – 4x

Now, the slope of the tangent line at x = 5 is f'(5). Evaluated, this is: f'(5) = 12 * 5 * 5 – 4 * 5 = 300 – 20 = 280

Now, we must find the intersection point on the original line:

f(5) = 4 * 5³ – 2 * 5² + 4 = 500 – 50 + 4 = 454

Therefore, the point of tangent intersection is (5,454).

Using the point-slope form of linear equations, we can find the line:

(y – 454) = 280(x – 5)

y – 454 = 280x – 1400

y = 280x – 946

Report an Error

Example Question #1 : Lines

Find the equation of the line tangent to y=x^2-x+3 at the point (1,3) .

Possible Answers:

y=x+2

y=0.5x+2

y=2x+2

y=0.5x+1

Correct answer:

y=x+2

Explanation:

The equation of the tangent line will have the form $y-y_{0}=m(x-x_{0})$ , where is the slope of the line and $(x_{0},y_{0})=(1,3)$ .

To find the slope, we need to evaluate the derivative at x=1 :

$\frac{dy}{dx}=\frac{d(x^2-x+3)}{dx}=2x-1\Rightarrow m=f'(1)=2*1-1=1$

Now that we have the slope, we can determine the equation of the tangent line using the point-slope formula:

$y-y_{0}=m(x-x_{0})\Rightarrow y-3=1(x-1)\Rightarrow y=x+2.$

Report an Error

Example Question #1 : Equation Of Line

Find the equation of the line tangent to f(x) at x=0 .

$f(x)=e^{3x} +x^2$

Possible Answers:

y=3

$y=3e^{3x} +2x$

y=3x+2

y=x^2 +2

y=3x+1

Correct answer:

y=3x+1

Explanation:

To find the equation of the line at that point, you need two things: the slope at that point and the y adjustment of the function, in the form y=mx+b , where m is the slope and is the y adjustment. To get the slope, find the derivative of f(x) and plug in the desired point for , giving us an answer of for the slope.

$f'(x)=3e^{3x} + 2x$

f'(0)=3

To find the y adjustment pick a point 0 in the original f(x) function. For simplicity, let's plug in x=0 , which gives us a y of 1, so an easy point is (0,1) . Next plug in those values into the equation of a line, y=mx + b . The new equation with all parameters plugged in is

1=(3)(0)+b

Now you simply solve for , which is .

Final equation of the line tangent to f(x) at x=0 is y=3x+1

Report an Error

Example Question #3 : Equation Of Line

Find the equation of the line tangent to f(x) at x=2 .

f(x)= ln(2x+1) +3

Possible Answers:

y=3x

$y=\frac{2}{5}x +3$

y=2x +3

$y=\frac{2}{5}x$

$y=\frac{2}{3}x$

Correct answer:

$y=\frac{2}{5}x +3$

Explanation:

To get the slope, find the derivative of f(x) and plug in the desired point for , giving us an answer of $\frac{2}{5}$ for the slope.

$f'(x)=\frac{2}{2x+1}$

$f'(2)=\frac{2}{5}$

Remember that the derivative of $ln(u) = \frac{u'}{u}$ .

To find the adjustment pick a point (for example) in the original f(x) function. For simplicity, let's plug in x=0 , which gives us a of , so an easy point is (0,3) . Next plug in those values into the equation of a line, y=mx + b . The new equation with all parameters plugged in is

$3=\frac{2}{5}(0)+b$

The coefficient in front of the is the slope.

Now you simply solve for , which is .

Final equation of the line tangent to f(x) at x=2 is $y=\frac{2}{5}x +3$ .

Report an Error

Example Question #231 : Graphing Functions

Find the equation of the line tangent to y=sin(2x) at $\frac{\pi }{2}$ .

Possible Answers:

y=-2x+1

y=2cos(2x)

$y=-2x+\pi$

$y=2x-\pi$

y=0

Correct answer:

$y=-2x+\pi$

Explanation:

The equation of the tangent line is y=mx+b. To find , the slope, calculate the derivative and plug in the desired point.

y'=2cos(2x)

$y'=2cos\left(2\cdot \frac{\pi}{2}\right)$

$y'=2cos(\pi)$

$y'\left(\frac{\pi}{2} \right )=-2$

The next step is to choose a coordinate on the original function. We can choose any value and calculate its value.

Let's choose $\frac{\pi }{2}$ .

$y=sin\left(2\cdot \frac{\pi}{2}\right)=sin(\pi)=0$

The value at this point is .

Plugging in those values we can solve for .

$0=(-2)\left(\frac{\pi }{2}\right)+b$

Solving for we get = $\pi$ .

$y=-2x+\pi$

Report an Error

Example Question #3 : Equation Of Line

A function, , is given by

f(x)=x^2-sin(x) .

Find the line tangent to at $x=\frac{\pi}{2}$ .

Possible Answers:

$y=\frac{\pi}{2} x-\frac{\pi^2}{4}$

$y=\pi x-\frac{\pi^2}{4}-1$

$y=2\pi x-\frac{\pi^2}{2}+1$

$y=\pi x-1$

$y=\pi x-\frac{\pi^2}{2}$

Correct answer:

$y=\pi x-\frac{\pi^2}{4}-1$

Explanation:

First we need to find the slope of at $x=\frac{\pi}{2}$ . To do this we need the derivative of . To take the derivative we need to use the power rule for the first term and recognize that the derivative of sine is cosine.

$\frac{df}{dx}=2x-cos(x)$

At,

$x=\frac{\pi}{2}, \frac{df}{dx}=\pi$

Now we need to know

$f\left(\frac{\pi}{2}\right)=\frac{\pi^2}{4}-1$ .

Now we have a slope, $m=\pi$ and a point $(x_1,y_1)=\left(\frac{\pi}{2},\frac{\pi^2}{4}-1\right)$

so we can use the point-slope formula to find the equation of the line.

y-y_1=m(x-x_1)

Plugging in and rearranging we find

$y=\pi x-\frac{\pi^2}{4}-1$ .

Report an Error

Example Question #5 : Equation Of Line

Let f(x)=2x^4+e^x-ex .

Find the equation for a line tangent to f(x) when x=1 .

Possible Answers:

y-1=2(x-2)

y-2=8(x-1)

y+8=2(x+1)

y-8=2(x-1)

8y=2(x-1)

Correct answer:

y-2=8(x-1)

Explanation:

First, evaluate f(x) when x=1 .

$f(1)=2(1)^4+e^1-e(1)=2+0\textbf{=2}$

Thus, we need a line that contains the point (1,2)

Next, find the derivative of f(x) and evaluate it at x=1 .

To find the derivative we will use the power rule,

$f(x)=x^n \rightarrow f'(x)=nx^{n-1}$ .

f'(x)=8x^3+e^x-e

f'(1)=8(1)^3+e^1-e=8+0=8

This indicates that we need a line with a slope of 8.

In point-slope form, $y-y_{1}=m(x-x_{1})$ , a line with the point (1,2) and a slope of 8 will be:

y-2=8(x-1)

Report an Error

Example Question #4 : Equation Of Line

What is the equation of the line tangent to $f(x)=\arctan (1-x^3)$ at x=-1 ? Round to the nearest hundreth.

Possible Answers:

y=0.2x

y=-0.6x+0.51

y=0.2x+1.31

y=-0.6

y=-0.6x

Correct answer:

y=-0.6x+0.51

Explanation:

The tangent line to f(x) at x=-1 must have the same slope as f(x) .

Applying the chain rule we get

$f'(x)=\frac{1}{1+(1-x^3)^2}(-3x^2)$ .

Therefore the slope of the line is,

$f'(-1)=-\frac{3}{5}=-0.6$ .

In addition, the tangent line touches the graph of f(x) at x=-1 . Since f(-1)=1.11 , the point (-1, 1.11) lies on the line.

Plugging in the slope and point we get y=-0.6x+0.51 .

Report an Error

Example Question #232 : Graphing Functions

Find the equation of the tangent line, where

f(x)=x^4-x^2+x-5 , at x=1 .

Possible Answers:

y=3x+1

y=3x+7

y=3x-1

y=3x-7

y=x

Correct answer:

y=3x-7

Explanation:

In order to find the equation of the tangent line at x=1 , we first find the slope.

To do this we need to find f'(x) .

f(x)=x^4-x^2+x-5

f'(x)=4x^3-2x+1

Since we have found f'(x) , now we simply plug in 1.

f'(1)=4(1)^3-2(1)+1=3

Now we need to plug in 1, into f(x) , to find a point that the tangent line touches.

f(1)=(1)^4-(1)^2+1-5=-4

Now we can use point-slope form to figure out what the equation of the tangent line is at x=1 .

Remember that point-slope for is

y-y_0=m(x-x_0)

where x_0 and y_0 is the point where the tangent line touches f(x) , and is the slope of the tangent line.

In our case, x_0=1 , y_0=-4 , and m=3 .

y+4=3(x-1)

y+4=3x-3

Thus our tangent line equation at x=1 is

y=3x-7 .

Report an Error

Example Question #5 : Equation Of Line

Find the equation of the tangent line of

f(x)=x^3+x , at x=1 .

Possible Answers:

y=4x+2

y=4x

y=2x+7

y=-4x-2

y=4x-2

Correct answer:

y=4x-2

Explanation:

In order to find the equation of the tangent line at x=1 , we first find the slope.

To do this we need to find f'(x) using the power rule $f(x)=x^n \rightarrow f'(x)=nx^{n-1}$ .

f(x)=x^3+x

f'(x)=3x^2+1

Since we have found f'(x) , now we simply plug in 1.

f'(1)=3(1)^2+1=4

Now we need to plug in 1, into f(x) , to find a point that the tangent line touches.

f(1)=(1)^3+1=2

Now we can use point-slope form to figure out what the equation of the tangent line is at x=1 .

Remember that point-slope for is

y-y_0=m(x-x_0)

where x_0 and y_0 is the point where the tangent line touches f(x) , and is the slope of the tangent line.

In our case, x_0=1 , y_0=2 , and m=4 .

y-2=4(x-1)

y-2=4x-4

Thus our tangent line equation at x=1 is

y=4x-2 .

Report an Error

View Calculus Tutors

Gary
Certified Tutor

SUNY at Albany, Bachelor of Science, Economics. Pace University-New York, Masters in Business Administration, Analysis and Fu...

View Calculus Tutors

Leonard
Certified Tutor

Florida State University, Bachelor of Science, Environmental Science.

View Calculus Tutors

Jonathan
Certified Tutor

University of Maryland-Baltimore County, Bachelor of Science, Mathematics.

All Calculus 1 Resources

10 Diagnostic Tests 438 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Chemistry Tutors in Chicago, ACT Tutors in Chicago, SSAT Tutors in Houston, Computer Science Tutors in San Diego, SSAT Tutors in Atlanta, Physics Tutors in San Francisco-Bay Area, ISEE Tutors in Miami, Biology Tutors in Boston, Reading Tutors in Philadelphia, Reading Tutors in Chicago

Popular Courses & Classes

ISEE Courses & Classes in Seattle, GMAT Courses & Classes in Boston, GMAT Courses & Classes in Denver, SSAT Courses & Classes in Boston, GMAT Courses & Classes in Houston, MCAT Courses & Classes in Miami, SSAT Courses & Classes in Los Angeles, ACT Courses & Classes in Miami, ISEE Courses & Classes in Boston, LSAT Courses & Classes in Seattle

Popular Test Prep

GRE Test Prep in Atlanta, GRE Test Prep in Dallas Fort Worth, ACT Test Prep in Miami, MCAT Test Prep in Phoenix, SAT Test Prep in Boston, LSAT Test Prep in Philadelphia, LSAT Test Prep in New York City, GMAT Test Prep in San Francisco-Bay Area, LSAT Test Prep in San Francisco-Bay Area, ISEE Test Prep in Boston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Calculus 1 : Equation of Line

Study concepts, example questions & explanations for Calculus 1

All Calculus 1 Resources

Example Questions

Example Question #1 : Equation Of Line

Example Question #1 : Lines

Example Question #1 : Equation Of Line

Example Question #3 : Equation Of Line

Example Question #231 : Graphing Functions

Example Question #3 : Equation Of Line

Example Question #5 : Equation Of Line

Example Question #4 : Equation Of Line

Example Question #232 : Graphing Functions

Example Question #5 : Equation Of Line

All Calculus 1 Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: