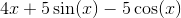If p(t) gives the position of a planet as a function of time, find the function which models the planet's velocity.

Velocity is the first derivative of position.

Therefore, all we need to do to solve this problem is to find the first derivative.

We can do this via the power rule and the rule for differentiating sine.

1) 

2) 

So, we these rules in mind, we get:

So our final answer is:

If p(t) gives the position of a planet as a function of time, find the planet's velocity when t=0.

Velocity is the first derivative of position. Therefore, all we need to do to solve this problem is to find the first derivative of p(t) and then plug in 0 for t and solve.

We can do this via the power rule and the rule for differentiating sine and cosine.

1) 

2) 

So, we these rules in mind, we get:

So our velocity function is:

Now, plug in 0 and simplify.

So, our answer is -11. We are not given any units, so we do not need to worry about them.

The rate of change of a function is given by the derivative of that function. So, to find the rate of change of production, we must take the first derivative of the function for production, which is equal to

found using the following rules:

, 

The rate of change of the concrete flow is given by the first derivative of the concrete flow function:

and was found using the following rules:

, , 

To determine the speed of the car, we must take the first derivative of the position function, which gives us the rate of change of the position of the car - in other words, speed. 

The derivative was found using the following rule:

, 

Evaluating the derivative function at t=5, we get our speed as

To determine which code the scientists will use, we must find the velocity and acceleration of the particle, given by the first and second derivatives of the position function, respectively, evaluated at the given point. 

The velocity and acceleration functions, therefore, are

The derivatives were found using the following rules:

, 

Evaluated at t=2, we find that

When the velocity is positive, but the acceleration is negative, the code the scientists use is .

To determine the velocity of the particle, we must take the first derivative of the position function - in other words, the rate of change of the position is the velocity:

The derivative was found using the following rule:

To find the velocity at the given point, we simply plug in the value into the velocity function:

The acceleration of a particle is its change in velocity; therefore, to find its acceleration at a particular point in time, it is necessary to take the derivative of the velocity function and evaluate it for that value.  In short, we want . 

Differentiate :

Apply the sum rule:

Apply the chain rule by setting :

This is the acceleration function; substitute 6 for :

The acceleration function of a particle with respect to time is the derivative  of the velocity function of the particle. 

Find this derivative by first using the product rule:

Apply the product rule:

Evaluate :

 feet per second squared.

To determine the velocity function - the rate of change of position - we must take the derivative of the position function:

The derivative was found using the following rules:

, 

To find the velocity at a certain value of x - the instantaneous rate of change of position - we simply plug in the given value of x into the velocity function: