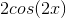All AP Calculus AB Resources
Example Questions
Example Question #1 : Analysis Of Curves, Including The Notions Of Monotonicity And Concavity
Find the intervals on which 
To find the intervals where the function is increasing, we need to find the points at which its slope changes from positive to negative and vice versa. The first derivative, which is the slope at any point, will help us.
First, we find the derivative of 
Also, the constant multiple rule will apply to the coefficients of each term. The constant multiple rule simply says that any constant factor of a term will "carry" to the derivative of that term. For example:
Lastly, the derivative of a constant is zero. This will result in the last term, 
Applying these rules, we find the derivative
Where the derivative is positive (blue line in graph), the tangent line to the original function is angled up. Where the derivative is negative (red line in graph), the slope of the tangent line is angled down.

The points where the tangent line's slope transitions from negative to positive or vice versa, are called the critical points. At these points, the tangent line becomes a horizontal line with a slope of zero (green line in graph). In other words, the "critical points" occur when the derivative is zero. These points will be the endpoints of our intervals of increasing and decreasing. To find the critical points, we will set the derivative equal to zero and solve for x. In this problem factoring is the best method:



Now that we have found the critical points, we need to know whether the original function is increasing or decreasing in the intervals between them. We will do so by testing a point in each interval and determining whether the derivative is positive or negative at that point. This is called the first derivative line test.
For the interval 

(Note: I will use the factored form of the derivative, but we could also use the polynomial version. Both will give the same result)
Since the derivative is negative at this point, we can conclude that the derivative is negative for the whole interval. Thus, the original function is decreasing on 
For the interval 

Since the derivative is positive at this point, we can again conclude that the derivative is positive for the whole interval. Thus, the original function is increasing on 
For the interval 

Since the derivative is negative at this point, we can again conclude that the derivative is negative for the whole interval. Thus, the original function is decreasing on 
Lastly, for the interval 

Since the derivative is positive at this point, we know that the derivative is positive for the whole interval. Thus the original function is increasing on 
From these 4 results, we now know the answer. The function is increasing on the intervals 
Example Question #41 : Second Derivatives
On what intervals is the function 
The question is asking when the derivative is negative and the second derivative is positive. First, taking the derivative, we get
Solving for the zero's, we see 



Thus, we can see that the derivative is only negative on the interval 
Repeating the process for the second derivative,
The reader can verify that this equation hits 0 at -4/3. Thus, the intervals to test for the second derivative are

Because we want the interval where the second derivative is positive and the first derivative is negative, we need to take the intersection or overlap of the two intervals we got:

If this step is confusing, try drawing it out on a number line -- the first interval is from -3 to 1/3, the second from -4/3 to infinity. They only overlap on the smaller interval of -4/3 to 1/3.
Thus, our final answer is
Example Question #42 : Second Derivatives
On a closed interval, the function 



Two or more of the other answers are correct.




If 



For 


For 


Thus, the only correct answer is that 
Example Question #541 : Derivatives
If
and 
then find 
We see the answer is 
Example Question #61 : Derivative As A Function
Practicing the chain rule level 1 B!
Find the derivative of the function
To understand why the answer is

you must understand that the derivative of

is actually

Next, you must understand that the derivative of
is actually












This means in order to differentiate the equation, you must use the chain rule. The chain rule tells us that in order to differentiate a composition function, you must differentiate all of the component composite functions in the reverse order that they are applied to the variable, one step at a time and multiply the results together.
the derivative of
is
Step 1: Only look at the outermost function f(x) first, then differentiate it
Step 2: Look at the next function g(x), keep it inside the other function f'(x).
Step 3: Differentiate the the next function g(x) but multiply it by f'(g(x))
So, substitute f'(x), g(x) and g'(x) for the expressions you found before:
And now you have found the correct answer.
-------------------------------------------------------------------------------------------
To sum it up, you multiply the derivative of the outermost functions by the derivative if the inner function while keeping the next inner functions the the same in every step until you are out of functions to find the derivative of. This also applies to more complicated functions. For instance,
If we have
then it's derivative would be
Notice how the factors become less complicated as you differentiate it or as you look from left to right.
If you are unsure of the pattern, look at the pattern in these equations

Example Question #62 : Derivative As A Function
Practicing the chain rule level 1 A!
Find the derivative of the function
To understand why the answer is

you must understand that the derivative of

is actually

Next, you must understand that the derivative of
is actually












This means in order to differentiate the equation, you must use the chain rule. The chain rule tells us that in order to differentiate a composition function, you must differentiate all of the composite functions in the reverse order that they are applied to the variable, one step at a time and multiply the results together.
the derivative of
is
Step 1: Only look at the outermost function f(x) first, then differentiate it
Step 2: Look at the next function g(x), keep it inside the other function f'(x).
Step 3: Differentiate the the next function g(x) but multiply it by f'(g(x))
So, substitute f'(x), g(x) and g'(x) for the expressions you found before:
And now you have found the correct answer.
-------------------------------------------------------------------------------------------
To sum it up, you multiply the derivative of the outermost functions by the derivative if the inner function while keeping the next inner functions the the same in every step until you are out of functions to find the derivative of. This also applies to more complicated functions. For instance,
If we have
then it's derivative would be
Notice how the factors become less complicated as you differentiate it or as you look from left to right.
If you are unsure of the pattern, look at the pattern in these equations

Example Question #63 : Derivative As A Function
Practicing the chain rule level 1 C!
Find the derivative of the function
To understand why the answer is

you must understand that the derivative of

is actually

Next, you must understand that the derivative of
is actually












This means in order to differentiate the equation, you must use the chain rule. The chain rule tells us that in order to differentiate a composition function, you must differentiate all of the component composite functions in the reverse order that they are applied to the variable, one step at a time and multiply the results together.
the derivative of
is
Step 1: Only look at the outermost function f(x) first, then differentiate it
Step 2: Look at the next function g(x), keep it inside the other function f'(x).
Step 3: Differentiate the the next function g(x) but multiply it by f'(g(x))
So, substitute f'(x), g(x) and g'(x) for the expressions you found before:
And now you have found the correct answer.
-------------------------------------------------------------------------------------------
To sum it up, you multiply the derivative of the outermost functions by the derivative if the inner function while keeping the next inner functions the the same in every step until you are out of functions to find the derivative of. This also applies to more complicated functions. For instance,
If we have
then it's derivative would be
Notice how the factors become less complicated as you differentiate it or as you look from left to right.
If you are unsure of the pattern, look at the pattern in these equations

Example Question #64 : Derivative As A Function
Practicing the chain rule level 1 D!
Find the derivative of the function
To understand why the answer is

you must understand that the derivative of

is actually

Next, you must understand that the derivative of
is actually












This means in order to differentiate the equation, you must use the chain rule. The chain rule tells us that in order to differentiate a composition function, you must differentiate all of the component composite functions in the reverse order that they are applied to the variable, one step at a time and multiply the results together.
the derivative of
is...
Step 1: Only look at the outermost function f(x) first, then differentiate it
Step 2: Look at the next function g(x), keep it inside the other function f'(x).
Step 3: Differentiate the the function g(x) but multiply its derivative by f'(g(x))
So, substitute f'(x), g(x) and g'(x) for the expressions you found before:
And now you have found the correct answer.
-------------------------------------------------------------------------------------------
To sum it up, you multiply the derivative of the outermost functions by the derivative if the inner function while keeping the next inner functions the same in every step until you are out of functions to find the derivative of. This also applies to more complicated functions. For instance,
If we have
then it's derivative would be
Notice how the factors become less complicated as you differentiate it or as you look from left to right.
If you are unsure of the pattern, look at the pattern in these equations

Example Question #65 : Derivative As A Function
Practicing the chain rule level 2 A!
Find the derivative of the function
To understand why the answer is

you must understand that the derivative of

is actually

Next, you must understand that the derivative of
is actually












This means in order to differentiate the equation, you must use the chain rule. The chain rule tells us that in order to differentiate a composition function, you must differentiate all of the composite functions in the reverse order that they are applied to the variable one step at a time and multiply the results together.
the derivative of
is...
Step 1: Only look at the outermost function f(x) first, then differentiate it
Step 2: Look at the next function g(x), keep it inside the other function f'(x).
Step 3: Differentiate the the function g(x) but multiply its derivative by f'(g(x))
So, substitute f'(x), g(x) and g'(x) for the expressions you found before:
And now you have found the correct answer.
-------------------------------------------------------------------------------------------
To sum it up, you multiply the derivative of the outermost functions by the derivative if the inner function while keeping the next inner functions the same in every step until you are out of functions to find the derivative of. This also applies to more complicated functions. For instance,
If we have
then it's derivative would be
Notice how the factors become less complicated as you differentiate it or as you look from left to right.
If you are unsure of the pattern, look at the pattern in these equations

Example Question #66 : Derivative As A Function
Practicing the chain rule level 2 B!
Find the derivative of the function
To understand why the answer is

you must understand that the derivative of

is actually

Next, you must understand that the derivative of
is actually












This means in order to differentiate the equation, you must use the chain rule. The chain rule tells us that in order to differentiate a composition function, you must differentiate all of the composite functions in the reverse order that they are applied to the variable one step at a time and multiply the results together.
the derivative of
is...
Step 1: Only look at the outermost function f(x) first, then differentiate it
Step 2: Look at the next function g(x), keep it inside the other function f'(x).
Step 3: Differentiate the the next function g(x) but multiply it by f'(g(x))
So, substitute f'(t), g(t) and g'(t) for the expressions you found before:
And now you have found the correct answer.
-------------------------------------------------------------------------------------------
To sum it up, you multiply the derivative of the outermost functions by the derivative if the inner function while keeping the next inner functions the the same in every step until you are out of functions to find the derivative of. This also applies to more complicated functions. For instance,
If we have
then it's derivative would be
Notice how the factors become less complicated as you differentiate it or as you look from left to right.
If you are unsure of the pattern, look at the pattern in these equations

Certified Tutor
Certified Tutor
All AP Calculus AB Resources





![\frac{\mathrm{d}}{\mathrm{d} x} [x^n] = n \cdot x^{n - 1}](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/946872/gif.latex)
![\frac{\mathrm{d} }{\mathrm{d} x}[-8x^3]= -8 \cdot \frac{\mathrm{d} }{\mathrm{d} x}[x^3] = -8 \cdot 3x^2 = -24x^2](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/946873/gif.latex)



![0 = 4 [ x^2(x - 6) - 3(x - 6)]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/946878/gif.latex)
![0 = 4[(x-6)(x^2 - 3)]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/946879/gif.latex)