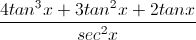Therefore, 

 for any positive , so , and

The instantaneous rate of change of  at  is , so we will find  and evaluate it at .

 for any positive , so 

The derivative of  is. It is probably best to memorize this fact (the proof follows from the difference quotient definition of a derivative).

Our function 

the factor of 3 does not change when we differentiate, therefore the answer is

The derivative of a sine function does not follow the power rule. It is one that should be memorized.

.

The derivatives of trig functions must be memorized. The first derivative is:

.

To find the second derivative, we take the derivative of our result.

.

Therefore, the second derivative will be .

We can use the Chain Rule:

Let , so that .

Use the Chain Rule.

Set  and substitute.

First, let's find out if the graph is increasing or decreasing. For that, we need the first derivative.

To find the first derivative, we can use the power rule. We lower the exponent on all the variables by one and multiply by the original variable.

We're going to treat  as  since anything to the zero power is one.

Notice that  since anything times zero is zero.

Plug in our given point for . If the result is positive, the function is increasing. If the result is negative, the function is decreasing.

Our result is negative, therefore the function is decreasing.

To find the concavity, look at the second derivative. If the function is positive at our given point, it is concave. If the function is negative, it is convex.

To find the second derivative we repeat the process, but using  as our expression.

As you can see, our second derivative is a constant. It doesn't matter what point we plug in for ; our output will always be negative. Therefore our graph will always be convex.

Combine our two pieces of information to see that at the given point, the graph is decreasing and convex.

To find the concavity, we need to look at the first and second derivatives at the given point. 

To take the first derivative of this equation, use the power rule. The power rule says that we lower the exponent of each variable by one and multiply that number by the original exponent:

Simplify:

Remember that anything to the zero power is equal to one.

The first derivative tells us if the function is increasing or decreasing. Plug in the given point, , to see if the result is positive (i.e. increasing) or negative (i.e. decreasing).

Therefore the function is increasing.

To find out if the function is convex, we need to look at the second derivative evaluated at the same point, , and check if it is positive or negative.

We're going to treat  as  since anything to the zero power is equal to one.

Notice that  since anything times zero is zero.

Plug in our given value:

Since the second derivative is positive, the function is convex. 

Therefore, we are looking at a graph that is both increasing and convex at our given point.

To find out if the function is increasing or decreasing, we need to look at the first derivative.

To find the first derivative, we can use the power rule. We lower the exponent on all the variables by one and multiply by the original variable.

Anything to the zero power is one.

Now we plug in our given value and find out if the result is positive or negative. If it is positive, the function is increasing. If it is negative, the function is decreasing.

Therefore, the function is decreasing.

To find out if it is concave or convex, look at the second derivative. If the result is positive, it is convex. If it is negative, then it is concave.

To find the second derivative, we repeat the process using  as our expression.

We're going to treat  as .

Notice that  since anything times zero is zero.

As stated before, anything to the zero power is one.

Since we get a positive constant, it doesn't matter where we look on the graph, as our second derivative will always be positive. That means that this graph is going to be convex at our given point.

Therefore, the function is decreasing and convex at our given point.