To find side length, simply realize that volume is the side length cubed. Thus,

To solve, simply take the cube root of the volume. Thus,

We begin with a picture, noting that the diagonal, labeled as , is the length across the cube from one vertex to the opposite side's vertex.

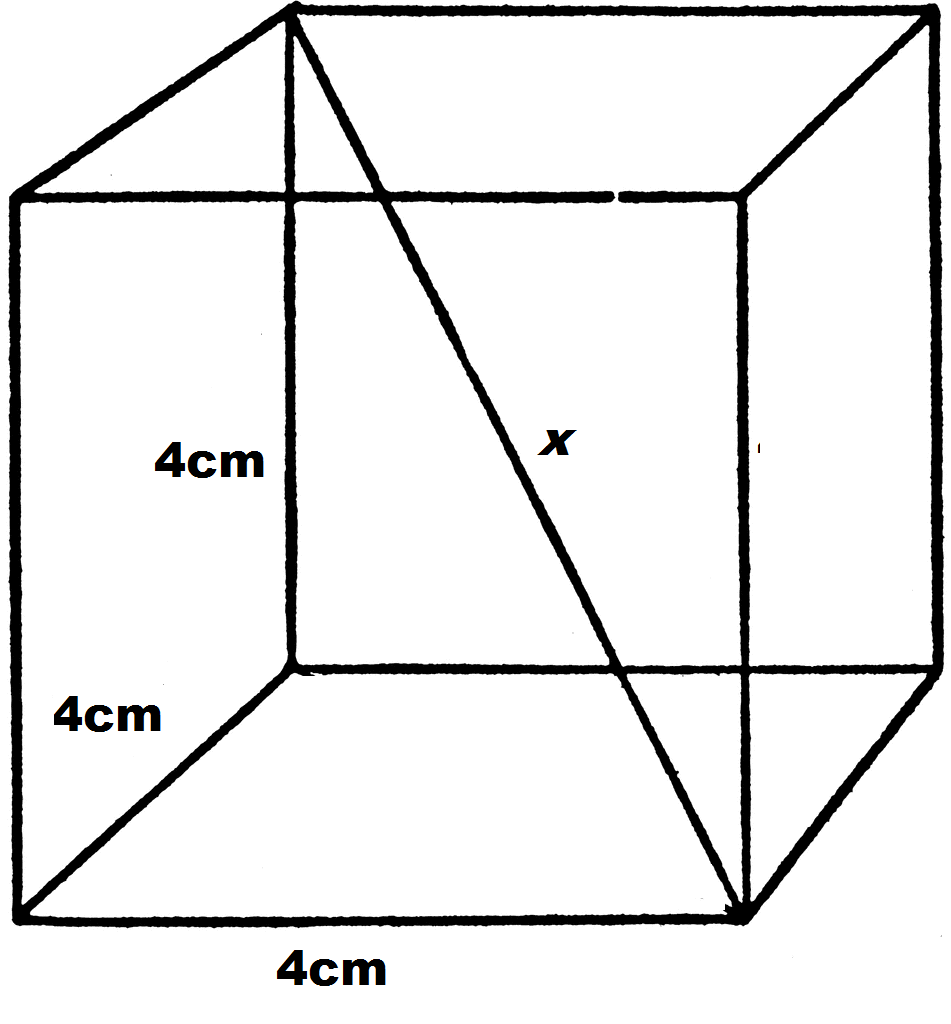
However, the trick to solving the problem is to also draw in the diagonal of the bottom face of the cube, which we labeled .

Note that this creates two right triangles.  Though our end goal is to find , we can begin by looking at the right triangle in the bottom face to find .  Using either the Pythagorean Theorem or the fact that we have a 45-45-90 right traingle, we can calculate the hypotenuse.

Now that we know the value of , we can turn to our second right triangle to find  using the Pythagorean Theorem.

Taking the square root of both sides and simplifying gives the answer.

Recall that the volume of a cube is computed using the equation

, where  is the length of one side of the cube.

So, for our data, we know:

Using your calculator, take the cube root of both sides. You can always do this by raising  to the  power if your calculator does not have a varied-root button.

If you get , the value really should be rounded up to . This is because of calculator estimations. So, if the sides are  , you can find the diagonal by using a variation on the Pythagorean Theorem working for three dimensions:

This is . Round it to .

Recall that the diagonal of a cube is most easily found when you know that cube's dimensions. For the volume of a cube, the pertinent equation is:

, where  represents the length of one side of the cube. For our data, this gives us:

Now, you could factor this by hand or use your calculator. You will see that  is .

Now, we find the diagonal by using a three-dimensional version of the Pythagorean Theorem / distance formula:

 or 

You can rewrite this:

The surface area of a cube = 6a² where a is the length of the side of each edge of the cube. Put another way, since all sides of a cube are equal, a is just the lenght of one side of a cube.

We have 96 = 6a² → a² = 16, so that's the area of one face of the cube.

Solving we get √16, so a = 4

A cube has 6 faces. The area of each face is found by squaring the length of the side.

Multiply the area of one face by the number of faces to get the total surface area of the cube.

The area of one face is given by the length of a side squared.

The area of 6 faces is then given by six times the area of one face: 54 cm².

We must first find the radius of the sphere in order to solve this problem. Since we already know the volume, we will use the volume formula to do this.

With the radius of the sphere in hand, we can now apply it to the cube. The radius of the sphere is half the distance from the top to the bottom of the cube (or half the distance from one side to another). Therefore, the radius represents half of a side length of a square. So in this case

The formula for the surface area of a cube is:

The surface area of the cube is

To answer this question, we need to find the surface area of a cube.

To do this, we must find the area of one face and multiply it by , because a cube has  faces that are square in shape and equal in size. 

To find the area of a square, you multiply its length by its width. (Note that the length and width of a square are the same.) Therefore, for this data:

We now must multiply the area of one face by 6 to get the total surface are of the cube.

Therefore, the surface are of a four-inch cube is .