We are open Saturday and Sunday!

Call Now to Set Up Tutoring:

(888) 888-0446

Trigonometry : Triangles

Study concepts, example questions & explanations for Trigonometry

Create An Account

All Trigonometry Resources

6 Diagnostic Tests 155 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #121 : Triangles

If the two legs of a right triangle are 5 and 7 respectively, what is the length of the hypotenuse?

Possible Answers:

$\sqrt {77}$

$\sqrt{73}$

$\sqrt{74}$

$\sqrt {80}$

Correct answer:

$\sqrt{74}$

Explanation:

Step 1: Recall the Pythagorean Theorem:

a^2+b^2=c^2 , where $a \and\ b$ are the legs of the triangle.

Step 2: Plug in the values that are given for the legs into the theorem:

(5)^2+(7)^2=c^2

Evaluate:

25+49=c^2

Add:

74=c^2

Step 3: To find , take the square root of both sides:

$\sqrt{74}=\sqrt{c^2}$

Reduce (if possible):

$\sqrt{74}=c$

The length of the hypotenuse is $\sqrt {74}$ .

Report an Error

Example Question #6 : Finding Sides

In a right triangle, one of the legs has a length of and a hypotenuse of . Find the length of the other side...

Possible Answers:

Correct answer:

Explanation:

Step 1: To find the missing side of a right triangle using the Pythagorean Theorem:

a^2+b^2=c^2 .

Step 2: Substitute in the known values.

$\\a^2+b^2=c^2 \\8^2+b^2=17^2$

Step 3: Solve for the missing variable by manipulating the equation to isolate the variable.

$\\b=\sqrt{17^2-8^2} \\b=\sqrt{289-64} \\b=\sqrt{225} \\b=15$

Report an Error

Example Question #7 : Finding Sides

Find the length of .

Possible Answers:

7.31

8.44

9.06

8.95

Correct answer:

8.44

Explanation:

Recall the Law of Sines for a generic triangle, as shown above:

$\frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C}$

Plug in the given values into the Law of Sines:

$\frac{14}{\sin 72}=\frac{b}{\sin 35}$

Rearrange the equation to solve for :

$b=(\sin 35)\frac{14}{\sin 72}=8.44$

Make sure to round to two places after the decimal.

Report an Error

Example Question #8 : Finding Sides

Find the length of side .

Possible Answers:

2.73

1.90

5.11

3.08

Correct answer:

2.73

Explanation:

Recall the Law of Sines:

$\frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C}$

Plug in the given values into the Law of Sines:

$\frac{6}{\sin 85}=\frac{b}{\sin 27}$

Rearrange the equation to solve for :

$b=(\sin 27)\frac{6}{\sin 85}=2.73$

Make sure to round to two places after the decimal.

Report an Error

Example Question #11 : Finding Sides

Find the length of side .

Possible Answers:

6.97

4.12

5.09

4.66

Correct answer:

4.66

Explanation:

Recall the Law of Sines:

$\frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C}$

Plug in the given values into the Law of Sines:

$\frac{7}{\sin 62}=\frac{b}{\sin 36}$

Rearrange the equation to solve for :

$b=(\sin 36)\frac{7}{\sin 62}=4.66$

Make sure to round to two places after the decimal.

Report an Error

Example Question #122 : Triangles

Find the length of side .

Possible Answers:

7.26

9.38

8.59

9.17

Correct answer:

9.17

Explanation:

Recall the Law of Sines:

$\frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C}$

Plug in the given values into the Law of Sines:

$\frac{12}{\sin 81}=\frac{b}{\sin 49}$

Rearrange the equation to solve for :

$b=(\sin 49)\frac{12}{\sin 81}=9.17$

Make sure to round to two places after the decimal.

Report an Error

Example Question #13 : Finding Sides

Find the length of side .

Possible Answers:

5.11

3.28

4.08

4.67

Correct answer:

4.08

Explanation:

Recall the Law of Sines:

$\frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C}$

Start by finding the value of angle :

A+47+49=180

A=84

Plug in the given values into the Law of Sines:

$\frac{3}{\sin 47}=\frac{a}{\sin 84}$

Rearrange the equation to solve for :

$a=(\sin 84)\frac{3}{\sin 47}=4.08$

Make sure to round to two places after the decimal.

Report an Error

Example Question #14 : Finding Sides

If is between and degrees, and $\small \cos x = \frac{3}{5}$ , what is $\small \tan x$ ?

Possible Answers:

$\small \frac{5}{3}$

$\small \frac{4}{3}$

$\small \frac{3}{4}$

$\small \frac{3}{5}$

$\small \frac{4}{5}$

Correct answer:

$\small \frac{4}{3}$

Explanation:

Solution

Using Pythagoras theory, you can see that is . If is , than $\small \tan x$ is $\small \frac{4}{3}$ , because $\small \tan x$ is $\small \frac{opposite}{adjacent}$

Report an Error

Example Question #1 : Solving Word Problems With Trigonometry

While waiting for your sister to finish her bungee jump, you decide to figure out how tall the platform she is jumping off is. You are standing 200 feet from the base of the platform, and the angle of elevation from your position to the top of the platform is degrees. How many feet tall is the platform?

Possible Answers:

144.50

376.15

449.71

298.05

Correct answer:

376.15

Explanation:

You can draw the following right triangle using the information given by the question:

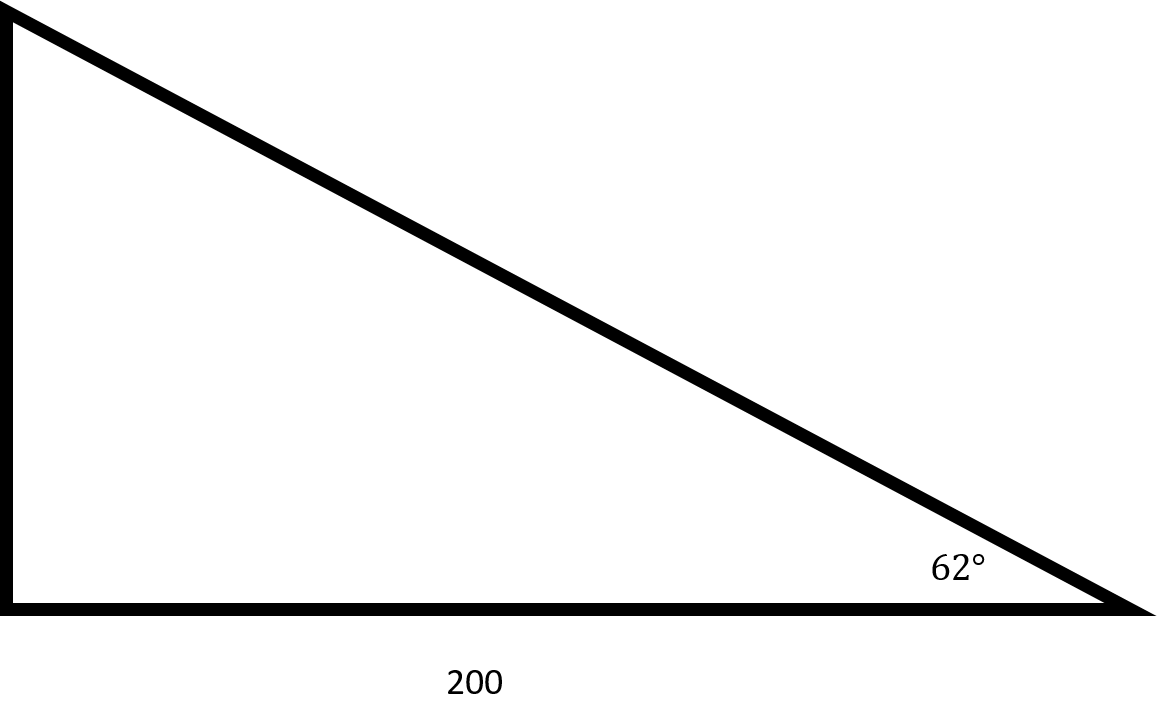
Since you want to find the height of the platform, you will need to use tangent.

$\tan 62=\frac{x}{200}$

x=376.15

Make sure to round to places after the decimal.

Report an Error

Example Question #1 : Solving Word Problems With Trigonometry

When the angle of elevation of the sun is degrees, a flagpole casts a shadow that is feet long. In feet, how tall is the flagpole?

Possible Answers:

6.25

5.75

6.50

7.50

Correct answer:

6.25

Explanation:

You can draw the following right triangle from the information given by the question.

In order to find the height of the flagpole, you will need to use tangent.

$\tan 32=\frac{\text{height}}{10}$

$\text{height}=6.25$

Make sure to round to places after the decimal.

The flagpole is 6.25 feet tall.

Report an Error

View Trigonometry Tutors

Marna
Certified Tutor

Drexel University, Bachelor of Science, Mathematics. Drexel University, Master's/Graduate, Mathematics and Applied Statistics.

View Trigonometry Tutors

Dalton
Certified Tutor

University of Massachusetts Amherst, Doctor of Philosophy, Mathematics.

View Trigonometry Tutors

Sekou
Certified Tutor

University of New Haven, Bachelor in Arts, Mathematics.

All Trigonometry Resources

6 Diagnostic Tests 155 Practice Tests Question of the Day Flashcards Learn by Concept

Trigonometry Tutors in Top Cities:

Popular Courses & Classes

MCAT Courses & Classes in Chicago, ACT Courses & Classes in New York City, ISEE Courses & Classes in Philadelphia, ISEE Courses & Classes in Miami, MCAT Courses & Classes in New York City, Spanish Courses & Classes in Atlanta, SAT Courses & Classes in Houston, SSAT Courses & Classes in Chicago, LSAT Courses & Classes in Boston, SAT Courses & Classes in Los Angeles

Popular Test Prep

ACT Test Prep in New York City, ISEE Test Prep in Denver, LSAT Test Prep in Los Angeles, MCAT Test Prep in Los Angeles, MCAT Test Prep in Philadelphia, LSAT Test Prep in Phoenix, ACT Test Prep in Philadelphia, SSAT Test Prep in Los Angeles, ISEE Test Prep in Seattle, ACT Test Prep in Atlanta

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

Trigonometry : Triangles

Study concepts, example questions & explanations for Trigonometry

All Trigonometry Resources

Example Questions

Example Question #121 : Triangles

Example Question #6 : Finding Sides

Example Question #7 : Finding Sides

Example Question #8 : Finding Sides

Example Question #11 : Finding Sides

Example Question #122 : Triangles

Example Question #13 : Finding Sides

Example Question #14 : Finding Sides

Example Question #1 : Solving Word Problems With Trigonometry

Example Question #1 : Solving Word Problems With Trigonometry

All Trigonometry Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: