All SSAT Upper Level Math Resources
Example Questions
Example Question #33 : Arithmetic Sequences
Find the next term in the following arithmetic sequence:
The common difference for this arithmetic sequence is 
Example Question #551 : Number Concepts And Operations
Find the next term in the following arithmetic sequence:
The sequence is subtracting 


Example Question #11 : How To Find The Next Term In An Arithmetic Sequence
Find the next number in this arithmetic sequence:
The common difference for this arithmetic sequence is 


Example Question #12 : How To Find The Next Term In An Arithmetic Sequence
Find the next term in this arithmetic sequence:
The common difference for this sequence is 

Example Question #1 : How To Interpret Venn Diagrams

Examine the above Venn diagram. The universal set 






Example Question #2 : How To Interpret Venn Diagrams

Examine the above Venn diagram. The universal set 















Example Question #1 : Interpretation Of Tables And Graphs

The above represents a Venn diagram. The universal set 
Let 


The region in which 2,431 appears depends on the sets of which 2,431 is an element, which in turn depends on which of 7, 11, and 13 divides it evenly:
2,431 is a multiple of 11 and 13, but not 7, so 2,431 is in 









However:
2,145 is divisible by 11 and 13 but not 7, so this is the correct choice.
Example Question #2 : Interpretation Of Tables And Graphs

The above Venn diagram represents all of this year's graduating seniors at Rockwell High School, the universal set 



Cathy was inducted into the National Honor Society in her junior year, and is still a member. She turned 18 on January 4 during her senior year, and she is carrying a respectable B average in her school's third-year French course. If her name were to be written in the above diagram in the correct place, in which of the five numbered regions would her name fall?
Cathy is in the Honor Society, meaning that she is in set 



Example Question #5 : Data Analysis / Probablility

The universal set 


The subsets are:



How many elements are in the set represented by the shaded region?
The correct answer is not given among the other responses.
The shaded region is 






To find ![c\left [ \left (A \cap C \right ) \cup \left (B \cap C \right ) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/258895/gif.latex)
![c\left [ \left (A \cap C \right ) \cap \left (B \cap C \right ) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/258896/gif.latex)


Example Question #2 : Interpretation Of Tables And Graphs

The universal set 
The subsets are:



How many elements are in the set represented by the shaded region?
The shaded region is inside 


Examine 

so seventeen elements are in 
We eliminate all elements in 
This leaves twelve elements in 
Certified Tutor
All SSAT Upper Level Math Resources



























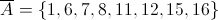

































![c\left [ \left (A \cap C \right ) \cup \left (B \cap C \right ) \right ] = 2](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/265841/gif.latex)
![c\left [ \left (A \cap C \right ) \cup \left (B \cap C \right ) \right ] = c \left (A \cap C \right )+ c \left (B \cap C \right )-c\left [ \left (A \cap C \right ) \cap \left (B \cap C \right ) \right ]](https://vt-vtwa-assets.varsitytutors.com/vt-vtwa/uploads/formula_image/image/258899/gif.latex)














