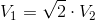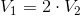Call Now to Set Up Tutoring:

(888) 888-0446

SSAT Upper Level Math : How to find the volume of a cylinder

Study concepts, example questions & explanations for SSAT Upper Level Math

Create An Account

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #723 : Geometry

The height of a cylinder is 3 inches and the radius of the circular end of the cylinder is 3 inches. Give the volume and surface area of the cylinder.

Possible Answers:

V=74.78 in^3, A=113.10in^2

V=74.78 in^3, A=103.04in^2

V=84.78 in^3, A=103.04in^2

V=84.82 in^3, A=113.10in^2

V=84.78 in^3, A=123.04in^2

Correct answer:

V=84.82 in^3, A=113.10in^2

Explanation:

The volume of a cylinder is found by multiplying the area of one end of the cylinder (base) by its height or:

$Volume=\pi r^2h$

where is the radius of the circular end of the cylinder and is the height of the cylinder. So we can write:

$Volume=\pi r^2h=\pi \times 3^2\times 3=84.82in^3$

The surface area of the cylinder is given by:

$A=2\pi r^2+2\pi rh$

where is the surface area of the cylinder, is the radius of the cylinder and is the height of the cylinder. So we can write:

$A=2\pi r^2+2\pi rh$

$A=2\pi (3)^2+2\pi \times 3\times 3$

$A=18\pi+18\pi$

$A=36\pi$

A=113.10

Report an Error

Example Question #1 : How To Find The Volume Of A Cylinder

The height of a cylinder is two times the length of the radius of the circular end of a cylinder. If the volume of the cylinder is $16\pi$ , what is the height of the cylinder?

Possible Answers:

$2\sqrt{2}$

Correct answer:

Explanation:

The volume of a cylinder is:

$Volume=\pi r^2h$

where is the radius of the circular end of the cylinder and is the height of the cylinder.

Since h=2r , we can substitute that into the volume formula. So we can write:

$Volume=\pi r^2h$

$16\pi=\pi r^2\cdot (2r)$

$16\pi =2\pi r^{3}$

$16=2r^{3}$

$8=r^{3}$

r=2

So we get:

$h=2r=2\times 2=4$

Report an Error

Example Question #2 : How To Find The Volume Of A Cylinder

The end (base) of a cylinder has an area of $16\pi$ square inches. If the height of the cylinder is half of the radius of the base of the cylinder, give the volume of the cylinder.

Possible Answers:

$8\pi$

$16\pi$

$32\pi$

Correct answer:

$32\pi$

Explanation:

The area of the end (base) of a cylinder is $\pi r^2$ , so we can write:

$\pi r^2=16\pi\Rightarrow r^2=16\Rightarrow r=4\ inches$

The height of the cylinder is half of the radius of the base of the cylinder, that means:

$h=\frac{r}{2}=\frac{4}{2}=2\ inches$

The volume of a cylinder is found by multiplying the area of one end of the cylinder (base) by its height:

$Volume=Area\times h=16\pi\times 2=32\pi$

$Volume=\pi r^2h=\pi\times 4^2\times 2=32\pi$

Report an Error

Example Question #3 : How To Find The Volume Of A Cylinder

We have two right cylinders. The radius of the base Cylinder 1 is $\sqrt{3}$ times more than that of Cylinder 2, and the height of Cylinder 2 is 4 times more than the height of Cylinder 1. The volume of Cylinder 1 is what fraction of the volume of Cylinder 2?

Possible Answers:

$V_{1}=\frac{2}{3}V_{2}$

$V_{1}=V_{2}$

$V_{1}=\frac{3}{2}V_{2}$

$V_{1}=\frac{3}{4}V_{2}$

$V_{1}=\frac{4}{3}V_{2}$

Correct answer:

$V_{1}=\frac{3}{4}V_{2}$

Explanation:

The volume of a cylinder is:

$V=\pi r^2h$

where is the volume of the cylinder, is the radius of the circular end of the cylinder, and is the height of the cylinder.

So we can write:

$V_{1}=\pi (r_{1})^2h_{1}$

and

$V_{2}=\pi (r_{2})^2h_{2}$

Now we can summarize the given information:

$r_{1}=\sqrt{3}\cdot r_{2}$

$h_{2}=4\cdot h_{1}\Rightarrow h_{1}=\frac{h_{2}}{4}$

Now substitute them in the $V_{1}$ formula:

$V_{1}=\pi (\sqrt{3}r_{2})^2\times \frac{h_{2}}{4}\Rightarrow V_{1}=\frac{3}{4}\pi (r_{2})^2\times h_{2}\Rightarrow V_{1}=\frac{3}{4}V_{2}$

Report an Error

Example Question #4 : How To Find The Volume Of A Cylinder

Two right cylinders have the same height. The radius of the base of the first cylinder is two times more than that of the second cylinder. Compare the volume of the two cylinders.

Possible Answers:

$V_{1}=\sqrt{2}\cdot V_{2}$

$V_{1}=V_{2}$

$V_{1}=3\cdot V_{2}$

$V_{1}=2\cdot V_{2}$

$V_{1}=4\cdot V_{2}$

Correct answer:

$V_{1}=4\cdot V_{2}$

Explanation:

The volume of a cylinder is:

$V=\pi r^2h$

where is the radius of the circular end of the cylinder and is the height of the cylinder. So we can write:

$V_{1}=\pi (r_{1})^2h_{1}$

$V_{2}=\pi (r_{2})^2h_{2}$

We know that

$h_{1}=h_{2}$

and

$r_{1}=2r_{2}$ .

So we can write:

$V_{1}=\pi (r_{1})^2h_{1}=\pi (2r_{2})^2\cdot h_{2}=4\pi (r_{2})^2h_{2}\Rightarrow V_{1}=4V_{2}$

Report an Error

Example Question #1 : How To Find The Volume Of A Cylinder

A cylinder has a diameter of inches and a height of inches. Find the volume, in cubic inches, of this cylinder.

Possible Answers:

98.3 in^3

402.1 in^3

308.9 in^3

100.5 in^3

Correct answer:

100.5 in^3

Explanation:

$\text{Volume of cylinder}=\pi \times \text{radius}^2 \times \text{height}$

Since we are given the diameter, divide that value in half to find the radius.

$\text{radius}=8\div2=4$

Now plug this value into the equation for the volume of a cylinder.

$\text{Volume}=\pi \times 4^4 \times 2$

$\text{Volume}=\pi \times 32=100.5$ in^3

Report an Error

Example Question #1993 : Hspt Mathematics

Find the volume, in cubic inches, of a cylinder that has a radius of inches and a height of inches.

Possible Answers:

2695.3 in^3

737.5 in^3

4456.1 in^3

3308.1 in^3

Correct answer:

3308.1 in^3

Explanation:

The formula to find the volume of a cylinder is $\pi r^2\times height$ .

Now, plug in the given numbers into this equation.

$\text{Volume}=\pi \times 9^2 \times 13$

$\text{Volume}=\pi \times 1053=3308.1$ in^3

Report an Error

Example Question #1 : How To Find The Volume Of A Cylinder

Find the volume of the cylinder if the circular base has an area of $\pi$ , and the height of the cylinder is also $\pi$ .

Possible Answers:

$\pi^3$

$\frac{\pi^3}{2}$

$\frac{\pi^2}{2}$

$\pi^2$

$\frac{\pi^3}{4}$

Correct answer:

$\pi^2$

Explanation:

Write the formula for volume of a cylinder.

$V=\pi r^2 h$

Remember that area of a circle is $A=\pi r^2$

Since the area of the circle is known, substitute the area into the formula.

$V=\pi(\pi)= \pi^2$

Report an Error

View SSAT- Upper Level Tutors

Gabby
Certified Tutor

University of Oregon, Bachelor in Arts, Journalism. University of Oregon, Current Grad Student, Communication, General.

View SSAT- Upper Level Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

View SSAT- Upper Level Tutors

Carneta
Certified Tutor

Langston University, Bachelor in Arts, Theater Arts.

All SSAT Upper Level Math Resources

6 Diagnostic Tests 311 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Calculus Tutors in Houston, GMAT Tutors in Phoenix, English Tutors in Los Angeles, Biology Tutors in Chicago, GMAT Tutors in Chicago, GRE Tutors in Philadelphia, Chemistry Tutors in Miami, LSAT Tutors in Chicago, ACT Tutors in San Francisco-Bay Area, Algebra Tutors in Seattle

Popular Courses & Classes

LSAT Courses & Classes in Dallas Fort Worth, ACT Courses & Classes in Washington DC, SAT Courses & Classes in Denver, Spanish Courses & Classes in Boston, ISEE Courses & Classes in Boston, LSAT Courses & Classes in Houston, ACT Courses & Classes in Atlanta, Spanish Courses & Classes in Miami, GMAT Courses & Classes in Los Angeles, GRE Courses & Classes in Miami

Popular Test Prep

ACT Test Prep in Miami, MCAT Test Prep in Phoenix, GMAT Test Prep in Seattle, MCAT Test Prep in Miami, SSAT Test Prep in Atlanta, LSAT Test Prep in Chicago, MCAT Test Prep in Houston, LSAT Test Prep in Miami, GMAT Test Prep in Los Angeles, SAT Test Prep in Miami

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

SSAT Upper Level Math : How to find the volume of a cylinder

Study concepts, example questions & explanations for SSAT Upper Level Math

All SSAT Upper Level Math Resources

Example Questions

Example Question #723 : Geometry

Example Question #1 : How To Find The Volume Of A Cylinder

Example Question #2 : How To Find The Volume Of A Cylinder

Example Question #3 : How To Find The Volume Of A Cylinder

Example Question #4 : How To Find The Volume Of A Cylinder

Example Question #1 : How To Find The Volume Of A Cylinder

Example Question #1993 : Hspt Mathematics

Example Question #1 : How To Find The Volume Of A Cylinder

All SSAT Upper Level Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: