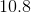All SSAT Middle Level Math Resources
Example Questions
Example Question #12 : How To Find The Decimal Equivalent Of A Fraction
Express 

Example Question #1322 : Numbers And Operations
Write as a decimal:
Divide 

The 6 repeats infinitely, so this can be written as
Example Question #21 : How To Find The Decimal Equivalent Of A Fraction
What decimal is equivalent to 


Example Question #21 : Understand Decimal Notation For Fractions, And Compare Decimal Fractions
What decimal is equivalent to 


Example Question #1322 : Numbers And Operations
What decimal is equivalent to


Example Question #1323 : Numbers And Operations
What decimal is equivalent to


Example Question #1 : Use Decimal Notation For Fractions With Denominators 10 Or 100: Ccss.Math.Content.4.Nf.C.6
What decimal is equivalent to 


Example Question #4 : Use Decimal Notation For Fractions With Denominators 10 Or 100: Ccss.Math.Content.4.Nf.C.6
What decimal is equivalent to 


Example Question #1321 : Numbers And Operations
Select the decimal that is equivalent to


Example Question #841 : Fractions
Select the decimal that is equivalent to


Certified Tutor
Certified Tutor
All SSAT Middle Level Math Resources