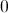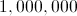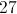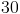The probability is expressed as the circumstance out of the whole number available.

The number of marbles that are red is 7, and the whole number of marbles is 20.

Therefore the probability is 

.

To find the probability of an event happening, we use the following

So, if we look at the number of ways we can draw a Queen, we can come up with

• Queen of Hearts
• Queen of Diamonds
• Queen of Spades
• Queen of Clubs

So, the number of ways we can draw a queen is equal to 4.  So,

Now, to find the total number of possible outcomes, we will think of how many different cards there are within a deck.  We know there are 52 total cards, which means there are 52 total possible outcomes.  So,

Now, we can simplify.

To find the probability of an event happening, we use the following

To calculate the number of ways this can happen it is important to recall that each suit (Hearts, Clubs, Spades, and Diamonds) have one value for numbers 1 through 10, one jack, one queen, one queen, and one ace. Therefore there is only one card that is the 2 of Hearts.

So, the number of ways we can draw a 2 of Hearts is equal to 1.  So,

Now, to find the total number of possible outcomes, we will think of how many different cards there are within a deck.  We know there are 52 total cards, which means there are 52 total possible outcomes.  So,

To find the probability of an event, we will use the following formula:

So, given the event of drawing a 4, we can calculate the following:

Because there are 4 ways to draw a 4 from a deck of cards:

• 4 of Hearts
• 4 of Diamonds
• 4 of Clubs
• 4 of Spades

Now, we can calculate the following:

Because there are 52 cards we could potentially draw from a deck of cards.

Knowing this, we can substitute into the formula.  We get

Therefore, the probability of drawing a 4 from a deck of cards is .

To find the probability of an event, we will use the following formula:

So, given the event of the teacher calling on a girl, we can calculate the following:

Because there are 11 girls in the classroom.

Now, we can calculate the following:

Because there are 26 total students the teacher could potentially call on.

Knowing this, we can substitute into the formula.  We get

Therefore, the probability of the teacher calling on a girl is .

To find the probability of an event, we will use the following formula:

So, given the event of drawing a 3, we can calculate the following:

\text{number of ways event can happen} = 4

Because there are 4 ways we can draw a 3 from the deck:

• 3 of clubs
• 3 of spades
• 3 of diamonds
• 3 of hearts

Now, we can calculate the following:

 \text{total number of possible outcomes} = 52

Because there are 52 cards we could potentially draw from a deck of cards.

Knowing this, we can substitute into the formula.  We get

Therefore, the probability of drawing a 3 from a deck of cards is .

The set of whole numbers comprises the following:

All of the given choices are members of this set.

Each entry in the sequence is obtained by adding to the previous entry a number that increases by 1 each time.

To get the next entry, add 7.

The set is increasing. To figure out by how much, simply subtract any two numbers in the set, but remember to put the greater number first!

Example:

Each number in the set increases by 1.1. To find the missing part of this set, add 1.1 to the number just before the blank.

The complete set is: 1.2, 2.3, 3.4, 4.5, 5.6.

First, you must notice that the set is increasing. When you look at how all the numbers are related, you will notice that this is a “growing” set, meaning the difference between each number in the set is increasing. To figure out by how much, simply subtract any two numbers in the set.

When you subtract the first two numbers in the set, you will get: .

If you subtract the next two numbers, you will get: .

Then the next two: .

Now you can see a pattern. Each number increases by 1, 2, 3, and so on.

To find the missing number in the set, we must add 6 to 21.

Now we can see the complete set.