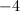SAT Math : SAT Mathematics
Study concepts, example questions & explanations for SAT Math
All SAT Math Resources
Example Questions
Example Question #2 : Linear / Rational / Variable Equations
There is no solution
1
–1/2
3
–3
There is no solution
Example Question #4 : Linear / Rational / Variable Equations
None of the other answers
A fraction is considered undefined when the denominator equals 0. Set the denominator equal to zero and solve for the variable.
Example Question #4 : How To Find Out When An Equation Has No Solution
Solve:
First, distribute, making sure to watch for negatives.
Combine like terms.
Subtract 7x from both sides.
Add 18 on both sides and be careful adding integers.
Example Question #5 : Equations / Inequalities
Solve:
Infinitely Many Solutions
No Solution
No Solution
First, distribute the 
Add 6x to both sides.
This is false for any value of 
Example Question #2 : How To Find Out When An Equation Has No Solution
Solve 
No solutions
No solutions
By definition, the absolute value of an expression can never be less than 0. Therefore, there are no solutions to the above expression.
Example Question #1 : How To Find Out When An Equation Has No Solution
, 
In the above graphic, approximately determine the x values where the graph is neither increasing or decreasing.
We need to find where the graph's slope is approximately zero. There is a straight line between the x values of 
Example Question #1 : Inequalities
|12x + 3y| < 15
What is the range of values for y, expressed in terms of x?
y > 15 – 12x
–5 – 4x < y < 5 – 4x
5 – 4x < y < 5 + 4x
5 + 4x < y < 5 – 4x
y < 5 – 4x
–5 – 4x < y < 5 – 4x
Recall that with absolute values and "less than" inequalities, we have to hold the following:
12x + 3y < 15
AND
12x + 3y > –15
Otherwise written, this is:
–15 < 12x + 3y < 15
In this form, we can solve for y. First, we have to subtract x from all 3 parts of the inequality:
–15 – 12x < 3y < 15 – 12x
Now, we have to divide each element by 3:
(–15 – 12x)/3 < y < (15 – 12x)/3
This simplifies to:
–5 – 4x < y < 5 – 4x
Example Question #1 : Inequalities
|4x + 14| > 30
What is a possible valid value of x?
1
4
7
–11
–3
7
This inequality could be rewritten as:
4x + 14 > 30 OR 4x + 14 < –30
Solve each for x:
4x + 14 > 30; 4x > 16; x > 4
4x + 14 < –30; 4x < –44; x < –11
Therefore, anything between –11 and 4 (inclusive) will not work. Hence, the answer is 7.
Example Question #2 : Inequalities
Given the inequality, |2x – 2| > 20,
what is a possible value for x?
–10
–8
0
11
10
–10
For this problem, we must take into account the absolute value.
First, we solve for 2x – 2 > 20. But we must also solve for 2x – 2 < –20 (please notice that we negate 20 and we also flip the inequality sign).
First step:
2x – 2 > 20
2x > 22
x > 11
Second step:
2x – 2 < –20
2x < –18
x < –9
Therefore, x > 11 and x < –9.
A possible value for x would be –10 since that is less than –9.
Note: the value 11 would not be a possible value for x because the inequality sign given does not include an equal sign.
Example Question #3 : Inequalities
Solve for 
Move +5 using subtraction rule which will give you
Divide both sides by 2 (using division rule) and you will get 
All SAT Math Resources
