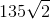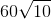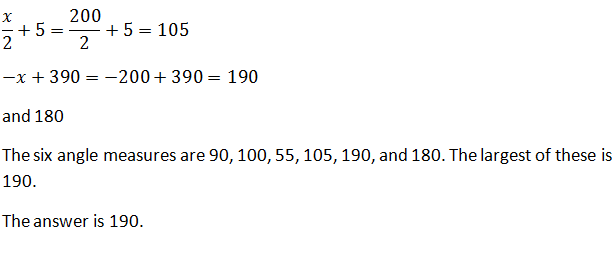Alvin runs at a rate of seven miles an hour for twelve minutes, or 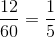\(\displaystyle \frac{12}{60} = \frac{1}{5}\) hours. The distance he runs is equal to his rate multiplied by his time, so, setting\(\displaystyle r = 7 , t = \frac{1}{5}\) in this formula:

\(\displaystyle d = rt\)

\(\displaystyle d = 7 \cdot \frac{1}{5} = \frac{7}{5}\) miles.

One mile comprises 5,280 feet, so this is equal to 

\(\displaystyle \frac{7}{5} \cdot 5,280 = 7,392\) feet

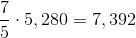
Since each side of the track measures 264 feet, this means that Alvin runs 

\(\displaystyle 7,392 \div 264 = 28\) sidelengths.

\(\displaystyle 28 \div 6 = 4 \textrm{ R }4\),

which means that Alvin runs around the track four complete times, plus four more sides of the track. Alvin stops when he is at Point E.

Below is the figure referenced; note that the hexagon is divided by its diameters, and that an apothem—a perpendicular bisector from the center to one side—has been drawn.

The circle has circumference \(\displaystyle C = 60 \pi\); its radius, which coincides with the apothem of the hexagon, \(\displaystyle OM\) is the circumference divided by \(\displaystyle 2\pi\):

\(\displaystyle r = \frac{C}{2\pi} = \frac{60 \pi }{2\pi} = 30\) 

The hexagon is divided into six equilateral triangles. One, \(\displaystyle \bigtriangleup AOB\), is divided by an apothem of the hexagon \(\displaystyle \overline{OM}\) - a radius of the circle - into two 30-60-90 triangles, one of which is \(\displaystyle \bigtriangleup AMO\). Since \(\displaystyle \overline{OM}\) has length 30, and it is a long leg of \(\displaystyle \bigtriangleup AMO\), then short leg \(\displaystyle \overline{AM}\) has length

\(\displaystyle AM = \frac{OM}{\sqrt{3}} = \frac{30}{\sqrt{3}} = \frac{30 \sqrt{3}}{\sqrt{3} \cdot \sqrt{3}} =\frac{30 \sqrt{3}}{3} = 10 \sqrt{3}\)

\(\displaystyle M\) is the midpoint of \(\displaystyle \overline{AB}\), one of the six congruent sides of the hexagon, so

\(\displaystyle AB = 2 \cdot AM = 2 \cdot 10 \sqrt{3} = 20\sqrt{3}\);

this makes the perimeter of the hexagon six times this, or 

\(\displaystyle P = 6 \cdot 20 \sqrt{3} = 120 \sqrt{3}\).

A diagonal is a line segment joining two non-adjacent vertices of a polygon.  A regular hexagon has six sides and six vertices.  One vertex has three diagonals, so a hexagon would have three diagonals times six vertices, or 18 diagonals.  Divide this number by 2 to account for duplicate diagonals between two vertices. The formula for the number of vertices in a polygon is:

\(\displaystyle \frac{n\cdot (n-3)}{2}\)

where \(\displaystyle n=\#\ of\ sides\).

A diagonal connects two non-consecutive vertices of a polygon.  A hexagon has six sides.  There are 3 diagonals from a single vertex, and there are 6 vertices on a hexagon, which suggests there would be 18 diagonals in a hexagon.  However, we must divide by two as half of the diagonals are common to the same vertices. Thus there are 9 unique diagonals in a hexagon. The formula for the number of diagonals of a polygon is:

\(\displaystyle \frac{n\cdot (n-3)}{2}\)

where n = the number of sides in the polygon.

Thus a pentagon thas 5 diagonals.  An octagon has 20 diagonals.

Below is the referenced hexagon, with some additional segments constructed.

Note that the segments \(\displaystyle \overline{MX}\) and \(\displaystyle \overline{HX}\) have been constructed. Along with \(\displaystyle \overline{MH}\), they form right triangle \(\displaystyle \bigtriangleup MHX\) with hypotenuse \(\displaystyle \overline{MX}\).

\(\displaystyle M\) is the midpoint of \(\displaystyle \overline{OH}\), so

\(\displaystyle MH= \frac{1}{2} \cdot OH = \frac{1}{2} \cdot 10 = 5\).

\(\displaystyle \overline{HX}\) has been divided by drawing the perpendicular from \(\displaystyle E\) to the segment and calling the point of intersection \(\displaystyle Y\). \(\displaystyle \bigtriangleup HYE\) is a 30-60-90 triangle with hypotenuse \(\displaystyle \overline{HE}\), short leg \(\displaystyle \overline{EY}\), and long leg \(\displaystyle \overline{HY}\), so by the 30-60-90 Triangle Theorem,

\(\displaystyle EY = \frac{1}{2} \cdot HE = \frac{1}{2} \cdot 10 = 5\)

and 

\(\displaystyle HY = EY \cdot \sqrt{3} \approx 5 \cdot 1.732 \approx 8.660\)

For the same reason, \(\displaystyle YX = 8.660\), so

\(\displaystyle HX = HY + YX \approx 8.660 + 8.660 \approx 17.320\)

By the Pythagorean Theorem,

\(\displaystyle MX= \sqrt{(MH)^{2}+ (HX)^{2}}\)

\(\displaystyle \approx \sqrt{(5)^{2}+ (17.320 )^{2}}\)

\(\displaystyle \approx \sqrt{25 + 300 }\)

\(\displaystyle \approx \sqrt{325 }\)

\(\displaystyle \approx 18.0\) when rounded to the nearest tenth.

One mile is equal to 5,280 feet; one fourth of a mile is equal to

\(\displaystyle \frac{1}{4} \times 5, 280 = 1,320 \textup{ feet}\) 

Each of the six congruent sides measures one sixth of this, or 

\(\displaystyle \frac{1}{6} \times 1,320 = 220\textup{ feet}\)

Teresa runs clockwise from Point A to halfway between Point E and Point F, so she runs along four and one half sides, for a total of

\(\displaystyle 4 \frac{1}{2} \times 220 = 990\textup{ feet}\) 

The sum of the interior angles of a polygon is given by \(\displaystyle 180(n - 2)\) where \(\displaystyle n\) = number of sides of the polygon.  An octagon has 8 sides, so the formula becomes \(\displaystyle 180(8 -2) = 1080\)

To solve, simply use the formula to find the total degrees inside a polygon, where n is the number of vertices.

In this particular case, a hexagon means a shape with six sides and thus six vertices.

Thus,

\(\displaystyle degrees=(n-2)*180=(6-2)*180=4*180=720\)

Because it can be split into two \(\displaystyle 30-60-90\) triangles, the area of an equilateral triangle can be expressed as...

\(\displaystyle \frac{1}{2}bh = \frac{1}{2}s(\frac{s\sqrt{3}}{2}) = \frac{s^2\sqrt{3}}{4}\)

With that in mind, the equilateral triangle in question has area of \(\displaystyle \frac{16\sqrt{3}}{4} = 4\sqrt{3}\).

Now consider that a regular hexagon can be split into six congruent equilateral triangles with a vertex at the center and the side opposite the center as one of the hexagon's sides (a handy way of finding a hexagon's area if you can't use the regular polygon formula requiring an apothem.) Knowing that, our answer is \(\displaystyle 4\sqrt{3} * 6 = 24\sqrt{3}\).