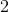SAT Math : How to find the area of a trapezoid
Study concepts, example questions & explanations for SAT Math
All SAT Math Resources
Example Questions
Example Question #1 : How To Find The Area Of A Trapezoid
A trapezoid has a base of length 4, another base of length s, and a height of length s. A square has sides of length s. What is the value of s such that the area of the trapezoid and the area of the square are equal?
In general, the formula for the area of a trapezoid is (1/2)(a + b)(h), where a and b are the lengths of the bases, and h is the length of the height. Thus, we can write the area for the trapezoid given in the problem as follows:
area of trapezoid = (1/2)(4 + s)(s)
Similarly, the area of a square with sides of length a is given by a2. Thus, the area of the square given in the problem is s2.
We now can set the area of the trapezoid equal to the area of the square and solve for s.
(1/2)(4 + s)(s) = s2
Multiply both sides by 2 to eliminate the 1/2.
(4 + s)(s) = 2s2
Distribute the s on the left.
4s + s2 = 2s2
Subtract s2 from both sides.
4s = s2
Because s must be a positive number, we can divide both sides by s.
4 = s
This means the value of s must be 4.
The answer is 4.
Example Question #1 : Quadrilaterals
Find the area of a trapezoid given bases of length 1 and 2 and height of 2.
To solve, simply use the formula for the area of a trapezoid. Thus,
Example Question #2 : Trapezoids
The above figure shows Square 
If Square 
Let 
Construct segment 
and
The area of Rectangle 
The lengths of the legs of Right 
and
The area of this right triangle is half the product of these lengths, or
This is seen below:
The sum of these areas is the area of Quadrilateral
Substituting 
Certified Tutor
All SAT Math Resources
