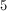All PSAT Math Resources
Example Questions
Example Question #101 : Statistics
The Brenner cousins' heights, in inches, are as follows:
Jeremy: 64
Vanessa: 69
Tracie: 60
Samuel: 70
Raymond: 74
Justin: 72
Patty: 55
Lauren: 52
Keith: 58
What is the median height of the cousins?
52
74
58
64
60
64
To find the median, one must arrange all the heights from the lowest to the highest value and then pick the middle value.
All values: 64 69 60 70 74 72 55 52 58
In order from lowest: 52 55 58 60 64 69 70 72 74
Median: 64
Example Question #2 : How To Find Median
Find the median in this set of numbers
2, 100, 52, 97, 1, 7, 22
97
52
1
22
7
22
in order to find the median, arrange the numbers in ascending order, and find the number in the middle of the list
Example Question #1 : How To Find Median
Find the median from the given set of numbers
1, 4, 8, 17, 8, 8, 15, 21, 32, 17
4
7
8
11.5
15
11.5
In order to find the median, arrange the numbers from smallest to biggest and find the number in the middle of the set.
In this case, both the 8 and 15 are in the middle of the set; so take the average of those 2 numbers (add both and divide by 2)
Example Question #3 : How To Find Median
The number n is to be added to the list {3, 4, 5, 6, 10, 12}. If n is an integer, which of the following could be the median of the new list of seven numbers?
I) 5
II) 5.5
III) 6
I and III only
I and II only
I only
I, II, and III
II and III only
I and III only
Before n is added to the list, the median is 5.5 (the average of 5 and 6). When n is added to the list, the number of elements becomes odd, so the median will be a value directly from the list, not the average of two values. All of the values in the old list are integers and n is an integer, so the new median must be an integer; therefore, 5.5 cannot be the median of the new list.
Considering some possible values of n, we see that in cases where n is less than or equal to 5, the fourth element in the new list would be 5, making the new median 5. In cases where n is greater than or equal to 6, the fourth element in the new list would be 6, making the new median 6. The possible values for the median of the new list are therefore 5 and 6, but not 5.5.
Example Question #11 : How To Find Median
M is a set consisting of a finite number of consecutive integers. If the average (arithmetic mean) of the numbers in set M is equal to one of the numbers in set M, which of the following must be true?
I. The number of numbers in set M is odd.
II. The average (arithmetic mean) of the numbers in set M equals the median.
III. Set M has a unique mode.
Statement I must be true because if M had an even number of consecutive integers, then its average (and median) would be the average of two consecutive integers, which is a decimal value rather than an integer, and therefore cannot be in set M, which contains only integers.
To check Statement II, consider some simple possible sets for M such as {0,1,2}. We see that in a set with an odd number of items, like M, the median is always the middle element. We also see that in a set with an odd number of consecutive integers (such as {0,1,2}), the average of the set will always be the exact middle element, too. Therefore, the average and the median must be equal, and Statement II must be true.
Finally, we can find a counterexample to statement III to show that it does not have to be true. If set M is {0,1,2}, we see that there is no unique mode (in fact, the only time set M could have a unique mode is when it only has one element!)
Example Question #11 : How To Find Median
If x > 0, what is the median of the set of numbers?
{x + 2, x – 4, x + 6, x – 8}
x – 1
x – 4
x + 2
x + 6
x + 1
x – 1
To find the median, you need to find the middle number of the ordered list. Since there are four numbers in the list, you need to average the two middle numbers.
Ordered list = {x – 8, x – 4, x + 2, x + 6}
((x – 4) + (x + 2))/2 = (2x – 2)/2 = x – 1
Example Question #11 : Median
A car travels at 60 miles per hour for 3 hours, 55 miles per hour for 2 hours, and 40 miles per hour for 3 hours. What (to the closest hundreth) is its average speed over the whole course of this trip?
51.67 miles per hour
55 miles per hour
58.13 miles per hour
51.25 miles per hour
19.38 miles per hour
51.25 miles per hour
The easiest way to solve this is to find the total number of miles traveled by the car and divide that by the total time travelled.
Recall that D = rt; therefore, for each of these three periods, we can calculate the distance and sum those products:
Dtotal = 60 * 3 + 55 * 2 + 40 * 3 = 180 + 110 + 120 = 410
The total amount of time travelled is: 3 + 2 + 3 = 8
Therefore, the average rate is 410 / 8 = 51.25 miles per hour.
Example Question #111 : Data Analysis
Set S consists of a set of six positive consecutive odd integers. Set T consists of the squares of each of the six elements in S. If the range of T is 560, then what is the median of T?
841
785
784
788
729
785
Let's let a and b stand for the smallest and largest values in S, respectively. Because T is equal to the square of the elements in S, then the smallest and largest values in T will be equal to a2 and b2 , respectively.
We are told that the range of T is equal to 560. The range of a set of numbers is the difference between the largest and smallest, which in this case we can represent as b2 – a2. We can now write the following equation:
b2 – a2 = 560
Noice that b2 – a2 can be factored as (b – a)(b + a), which is the formula for the difference of squares.
(b – a)(b + a) = 560
Notice that the value b – a represents the range of S, because it is the difference between the largest and smallest values of S.
We are told that S consists of six consecutive odd integers. We can use this information to find the range of S. Because they are consecutive odd integers, each member in S will be two larger than the one before it. Thus, we could represent S as follows:
a, a + 2, a + 4, a + 6, a + 8, a + 10
The range of S is equal (a + 10) – a = 10. Thus, b – a must equal 10.
Let's go back to the equation (b – a)(b + a) = 560. We can replace b – a with 10 and solve for b + a.
(10)(b + a) = 560
Divide both sides by 10.
b + a = 56
So, we know that b – a = 10, and b + a = 56. We can solve this system of equations to find the values of a and b, which will give us the smallest and largest values in S.
Let's solve the first equation in terms of b.
b – a = 10
Add a to both sides.
b = 10 + a
Now we can substitute 10 + a in for b in the second equation.
(10 + a) + a = 56
10 + 2a = 56
Subtract 10 from both sides.
2a = 46
Divide both sides by 2.
a = 23
b = 10 + a = 10 + 23 = 33
The smallest value of S is 23, and the largest value is 33. Thus, S consists of the following set:
23, 25, 27, 29, 31, 33
We are told that T is equal to the squares of the elements of S. Thus, T consists of the following numbers:
529, 625, 729, 841, 961, 1089
We are asked ultimately to find the median of T. The median of any set is the middle number. In this case, T has six elements, so there are actually two numbers in the middle: 729 and 841. To find the median, we will take the average of the two middle numbers. The average of two numbers is equal to their sum divided by two.
median of T = (729 + 841)/2 = 1570/2 = 785.
The answer is 785.
Example Question #3031 : Sat Mathematics
The median of a set of eight consecutive odd integers is 22. What is the largest integer in the set?
31
33
25
29
27
29
The set consists of eight consecutive odd integers. Let's call the integers, in order from least to greatest, A, B, C, D, E, F, G, and H. We are told that the median of the set is 22. The median in a set of numbers is the number in the middle. In the set of A, B, C, D, E, F, G, and H, the numbers represented by D and E would be in the middle. When we have two members in the middle, the median is equal to their average. Thus, 22 is equal to the average of D and E. In other words, 22 is the average of the 4th and 5th numbers in the set. Since 22 is halfway between 21 and 23, it makes sense that the 4th and 5th numbers would have to be 21 and 23, respectively. Thus, our set looks like this:
A, B, C, 21, 23, F, G, H
Beacuse the set consists of consecutive odd integers, each integer is two larger than the one before it. Therefore, this is the set of integers:
15, 17, 19, 21, 23, 25, 27, 29
The problem asks us to find the largest integer in the set, which is 29.
The answer is 29.
Example Question #112 : Data Analysis
Find the median
To find the median, arrange the numbers from lowest to highest then find the middle number.
There are two numbers in the middle in this set (

In this case, the median is 6 but you would typically find the average of the two numbers in the middle.
Certified Tutor
All PSAT Math Resources