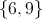All PSAT Math Resources
Example Questions
Example Question #91 : Data Analysis
100 students are in the 10th grade class. 30 are swimmers, 40 are runners, and 20 are swimmers and runners. What is the probability that a student is a swimmer OR a runner?
1/5
2/3
1/2
1/3
1/4
1/2
The formula for intersection is P(a or b) = P(a) + P(b) – P(a and b).
Now, 30 students out of 100 swim, so P(swim) = 30/100 = 3/10.
40 students run out of 100, so P(run) = 40/100 = 4/10. Notice how we are keeping 10 as the common denominator even though we could simplify this further. Keeping all of the fractions similar will make the addition and subtraction easier later on.
Finally, 20 students swim AND run, so P(swim AND run) = 20/100 = 2/10. (Again, we keep this as 2/10 instead of 1/5 so that we can combine the three fractions more easily.)
P(swim OR run) = P(swim) + P(run) – P(swim and run)
= 3/10 + 4/10 – 2/10 = 5/10 = 1/2.
Example Question #1 : Intersection
100 students are in the 10th grade class. 30 are swimmers, 40 are runners, and 20 are swimmers and runners. What is the probability that a student is a swimmer OR a runner?
2/3
1/5
1/3
1/4
1/2
1/2
The formula for intersection is P(a or b) = P(a) + P(b) – P(a and b).
Now, 30 students out of 100 swim, so P(swim) = 30/100 = 3/10.
40 students run out of 100, so P(run) = 40/100 = 4/10. Notice how we are keeping 10 as the common denominator even though we could simplify this further. Keeping all of the fractions similar will make the addition and subtraction easier later on.
Finally, 20 students swim AND run, so P(swim AND run) = 20/100 = 2/10. (Again, we keep this as 2/10 instead of 1/5 so that we can combine the three fractions more easily.)
P(swim OR run) = P(swim) + P(run) – P(swim and run)
= 3/10 + 4/10 – 2/10 = 5/10 = 1/2.
Example Question #11 : Venn Diagrams


Find 
The intersection of two sets contains every element that is present in both sets, so 
Example Question #11 : Venn Diagrams
We have two sports clubs offered to a class of 100 students. 70 students joined the basketball club, 40 students joined the swimming club, and 10 students joined neither. How many students joined both the swimming club and the basketball club?
The idea is to draw a Venn Diagram and find the intersection. We have one circle of 70 and another with 40. When we add the two circles plus the 10 students who joined neither, we should get 100 students. However, when adding the two circles, we are adding the intersections twice, therefore we need to subtract the intersection once.
We get 
Example Question #93 : Data Analysis
Find 
This question is asking you to find the intersection of Set A and Set B. The intersection of two sets contains only the things which are already in both Set A and Set B. Because the numbers 5 and 9 are the only numbers that are shared in common between Set A and Set B, the intersection consists of only those two numbers. 
Example Question #1 : How To Find The Intersection Of A Venn Diagram
Fifty 6th graders were asked what their favorite school subjects were. Three students like math, science and English. Five students liked math and science. Seven students liked math and English. Eight people liked science and English. Twenty students liked science. Twenty-eight students liked English. Fourteen students liked math. How many students didn’t like any of these classes?
None of the answers are correct
7
3
5
10
5
Draw a Venn diagram with three subsets: Math, Science, and English. Start in the center with students that like all three subjects. Next, look at students that liked two subjects. Be sure to subtract out the ones already counted in the middle. Then, look at the students that only like one subject. Be sure to subtract out the students already accounted for. Once all of the subsets are filled, look at those students who don’t like any of these subjects. To find the students who don’t like any of these subjects add all of the students who like at least one subject from the total number of students surveyed, which is 50.
M = math
S = science
E = English
M∩S∩E = 3
M∩S = 5 (but 3 are already accounted for) so 2 for M and S ONLY
M∩E = 7 (but 3 are already accounted for) so 4 for M and E ONLY
S∩E = 8 (but 3 are already accounted for) so 5 for S and E ONLY
M = 14 (but 3 + 2 + 4 are already accounted for) so 5 for M ONLY
S = 20 (but 3 + 2 + 5 are already accounted for) so 10 for S ONLY
E = 28 (but 3 + 4 + 5 are already accounted for) so 16 for E ONLY
Therefore, the students already accounted for is 3 + 2 +4 + 5 + 5 + 10 + 16 = 45 students
So, those students who don’t like any of these subjects are 50 – 45 = 5 students
Example Question #1 : How To Find The Intersection Of A Venn Diagram
Set A contains the positive even integers less than 14. Set B contains the positive multiples of three less than 20. What is the intersection of the two sets?
A∩B = {4, 6, 8}
A∩B = {6, 12}
A∩B = { }
A∩B = {6, 12, 18}
A∩B = {6}
A∩B = {6, 12}
A = {2, 4, 6, 8, 10, 12}
B = {3, 6, 9, 12, 15, 18}
The intersection of a set means that the elements are in both sets: A∩B = {6, 12}
Example Question #1 : How To Find The Intersection Of A Venn Diagram
Students at a local high school are given the option to take one gym class, one music class or one of each. Out of 100 students, 60 say that they are currently taking a gym class and 70 say that they are taking a music class. How many students are taking both?
This problem can be solved two ways, with a formula or with reason.
Using the formula, the intersection of the Venn diagram for which classes students take is:
By using reason, it is clear that 60 + 70 is greater than 100 by 30. It is assumed that this extra 30 students come from students who were counted twice because they took both classes.
Example Question #2 : Intersection
There are 75 juniors at a high school. 15 of the students are enrolled in Physics and 40 students are enrolled in Chemistry. 30 students are not enrolled in either Physics or Chemistry. How many students are enrolled in both Physics and Chemistry?
25
10
15
30
5
10
First, subtract the students that are in neither class; 75 – 30 = 45 students.
Thus, 45 students are enrolled in Chemistry, Physics, or both. Of these 45 students, we know 40 are in Chemistry, so that leaves 5 students who are enrolled in Physics only; with 15 total students in Physics, that means 10 must be in Chemistry as well. So 10 students are in both Physics and Chemistry.
Example Question #1 : Data Analysis
In a group of 83 gym members, 51 are taking kickboxing and 25 are taking yoga. Of the students taking kickboxing or yoga, 11 are taking both classes. How many members are not taking either course?
18
39
7
24
18
If 11 people are taking both courses, this means 51-11 or 40 are taking kickboxing only and 25-11 or 14 are taking yoga only. The number of people taking at least one course, therefore, is 40 + 14 + 11 = 65. The 83 members minus the 65 that are taking courses leaves 18 who are not taking any courses.
Certified Tutor
All PSAT Math Resources