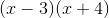Call Now to Set Up Tutoring:

(888) 888-0446

PSAT Math : Algebra

Study concepts, example questions & explanations for PSAT Math

Create An Account

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Example Questions

Example Question #3 : How To Find The Value Of The Coefficient

Give the coefficient of $x^{5}$ in the binomial expansion of $\left ( 0.2x+ 5 \right )^{7}$ .

Possible Answers:

20.16

2,625

315,000

0.168

Correct answer:

0.168

Explanation:

If the expression $\left ( A x + B\right )^{n}$ is expanded, then by the binomial theorem, the $x^{k}$ term is

$C(n, k) \cdot\left ( Ax \right )^{k} \cdot B ^{n - k}$

$= C(n, k) \cdot A ^{k} \cdot B ^{n - k} \cdot x^{k}$

or, equivalently, the coefficient of $x^{k}$ is

$C(n, k) \cdot A ^{k} \cdot B ^{n - k}$

Therefore, the $x^{5}$ coefficient can be determined by setting

A = 0.2, B =5, k=5, n = 7

$C(7,5) \cdot 0.2^{5} \cdot 5 ^{7 - 5}$

$= C(7,5) \cdot 0.2 ^{5} \cdot 5 ^{2}$

$= 21\cdot 0.00032 \cdot 25$

= 0.168

Report an Error

Example Question #2 : How To Find The Value Of The Coefficient

Give the coefficient of $x^{2}$ in the product

$\left (3x- 7 \right ) \left ( 4x+3\right )\left ( 2x-7\right )$ .

Possible Answers:

158

Correct answer:

Explanation:

While this problem can be answered by multiplying the three binomials, it is not necessary. There are three ways to multiply one term from each binomial such that two terms and one constant are multiplied; find the three products and add them, as follows:

$\left (\underline{3x}- 7 \right ) \left ( \underline{4x}+3\right )\left ( 2x\underline{-7}\right )$

$3x \cdot 4x \cdot (-7) = -84x^{2}$

$\left (\underline{3x}- 7 \right ) \left ( 4x\underline{+3}\right )\left ( \underline{2x}-7\right )$

$3x \cdot 3 \cdot 2x = 18x^{2}$

$\left (3x\underline{- 7} \right ) \left ( \underline{4x}+3\right )\left ( \underline{2x}-7\right )$

$-7 \cdot 4x \cdot 2x = -56x^{2}$

Add: $-84x^{2} + 18x^{2} - 56x^{2} = -122x^{2}$

The correct response is -122.

Report an Error

Example Question #4521 : Algebra 1

Give the coefficient of $x^{2}$ in the product

$\left ( x+ 0.4\right ) (x - 0.2) (3x-0.7)$ .

Possible Answers:

1.3

2.5

0.5

0.7

-0.1

Correct answer:

-0.1

Explanation:

$\left (\underline{ x}+ 0.4\right ) (\underline{x} - 0.2) (3x\underline{-0.7})$

$x \cdot x \cdot (-0.7) = -0.7x^{2}$

$\left (\underline{ x}+ 0.4\right ) (x \underline{-0.2})(\underline{3x}-0.7)$

$x \cdot (-0.2) \cdot 3x= -0.6x^{2}$

$\left (x+ \underline{0.4}\right ) (\underline{x} - 0.2)(\underline{3x}-0.7)$

$0.4 \cdot x \cdot 3x = 1.2 x^{2}$

Add: $-0.7x^{2}+ (-0.6x^{2})+ 1.2x^{2} = -0.1x^{2}$ .

The correct response is -0.1 .

Report an Error

Example Question #1 : How To Find The Solution To A Binomial Problem

Multiply the binomial.

(x-3)(x+4)

Possible Answers:

$x^{2}-12$

$x^{2}-x-12$

$x^{2}+x+12$

$x^{2}+x-12$

Correct answer:

$x^{2}+x-12$

Explanation:

By multiplying with the foil method, we multiply our first values giving x^2 , our outside values giving . our inside values which gives -3x , and out last values giving .

Report an Error

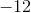
Example Question #1 : Trinomials

Factor the following expression completely:

x^4-8x^3+12x^2

Possible Answers:

x^2(x-6)(x-2)

x^2(x+6)(x+2)

x^2(x^2-8x+12)

x^2(x+3)(x+4)

x^2(x-3)(x-4)

Correct answer:

x^2(x-6)(x-2)

Explanation:

We must begin by factoring out x^2 from each term.

x^2(x^2-8x+12)

Next, we must find two numbers that sum to and multiply to .

(-6)+(-2)=-8

-6*-2=12

Thus, our final answer is:

x^2(x-6)(x-2)

Report an Error

Example Question #2 : Trinomials

Factor the following trinomial:

x^2-x-12

Possible Answers:

(x+3)(x-4)

(x-3)(x-4)

(x-1)(x-12)

(x-6)(x+2)

(x+4)(x-3)

Correct answer:

(x+3)(x-4)

Explanation:

x^2-x-12

To trinomial is in ax+bx+c form

In order to factor, find two numbers whose procuct is , in this case , and whose sum is , in this case

Factors of :

-1, 12; 1, -12; -3,4; 3,-4;-2,6; 2,-6

Which of these pairs has a sum of ?

and

Therefore the factored form of x^2-x-12 is:

(x+3)(x-4)

Report an Error

Example Question #31 : Polynomials

Factor the trinomial.

$x^{2}-5x-14$

Possible Answers:

(x-8)(x+3)

(x-7)(x+2)

(x+7)(x-2)

(x-3)(x-2)

Correct answer:

(x-7)(x+2)

Explanation:

Our factors will need to have a product of (-14) , and a sum of (-5) , so our factors must be (-7) and .

Report an Error

Example Question #41 : Algebra

(x^2+y^2+z^2)(x^2+y^2+z^2)

Possible Answers:

x^4+y^4+z^4+2x^2y^2+2y^2z^2+2x^2z^2

x^4+x^2y^2+y^2z^2+z^4

2x^2y^2+z^4+y^4+2z^2y^2

x^4+y^4+z^4

x^2y^2+y^2z^2+x^2z^2

Correct answer:

x^4+y^4+z^4+2x^2y^2+2y^2z^2+2x^2z^2

Explanation:

(x^2+y^2+z^2)(x^2+y^2+z^2)

Use the distributive property:

x^2(x^2+y^2+z^2)+y^2(x^2+y^2+z^2)+z^2(x^2+y^2+z^2)

x^4+x^2y^2+x^2z^2 + x^2y^2+y^4+y^2z^2+x^2z^2+y^2z^2+z^4

Combine like terms:

x^4+y^4+z^4+2x^2y^2+2y^2z^2+2x^2z^2

Report an Error

Example Question #33 : Polynomials

Find the product:

(x^2+7x+4)(x^2-3x-2)

Possible Answers:

2x^2-4x-2

x^4+4x^3-19x^2-26x-8

x^4-10x^3+27x^2-14x-4

x^4-21x-8

2x^2+4x+2

Correct answer:

x^4+4x^3-19x^2-26x-8

Explanation:

Find the product:

(x^2+7x+4)(x^2-3x-2)

Step 1: Use the Distributive Property

x^2(x^2-3x-2)+7x(x^2-3x-2)+4(x^2-3x-2)

x^4-3x^3-2x^2+7x^3-21x^2-14x+4x^2-12x-8

Step 2: Combine like terms

x^4+4x^3-19x^2-26x-8

Report an Error

Example Question #3 : Trinomials

Find the product:

(2x^2+3y+6)(7x^2+4y+1)

Possible Answers:

21x^2y+12y^2+3y

14x^2+29x^2y+44x^2+12y^2+27y+6

14x^4+8x^2y+2x^2

14x^2+8x^2y+12y^2+8

42x^2+24y+6

Correct answer:

14x^2+29x^2y+44x^2+12y^2+27y+6

Explanation:

Find the product:

(2x^2+3y+6)(7x^2+4y+1)

Use the distributive property:

2x^2(7x^2+4y+1)+3y(7x^2+4y+1)+6(7x^2+4y+1)

14x^4+8x^2y+2x^2+21x^2y+12y^2+3y+42x^2+24y+6

14x^4+29x^2y+44x^2+12y^2+27y+6

Report an Error

View PSAT Mathematics Tutors

Sumit
Certified Tutor

Calcutta University, Bachelor of Science, Mathematics. Indian Statistical Institute, Master of Science, Mathematics.

View PSAT Mathematics Tutors

Trenton
Certified Tutor

United States Military Academy, Bachelor of Science, Mathematics.

View PSAT Mathematics Tutors

Liban
Certified Tutor

Emory University, Bachelor of Science, Mathematics/Economics.

All PSAT Math Resources

10 Diagnostic Tests 421 Practice Tests Question of the Day Flashcards Learn by Concept

Popular Subjects

Reading Tutors in Philadelphia, English Tutors in Houston, English Tutors in Denver, Algebra Tutors in Los Angeles, GRE Tutors in Miami, SAT Tutors in Denver, SSAT Tutors in Dallas Fort Worth, LSAT Tutors in Dallas Fort Worth, French Tutors in Atlanta, Calculus Tutors in Washington DC

Popular Courses & Classes

SAT Courses & Classes in Chicago, MCAT Courses & Classes in Miami, SSAT Courses & Classes in Seattle, SAT Courses & Classes in Denver, GMAT Courses & Classes in San Francisco-Bay Area, ACT Courses & Classes in Miami, LSAT Courses & Classes in Los Angeles, LSAT Courses & Classes in Dallas Fort Worth, GMAT Courses & Classes in Denver, LSAT Courses & Classes in San Francisco-Bay Area

Popular Test Prep

ISEE Test Prep in Denver, SSAT Test Prep in San Diego, MCAT Test Prep in Denver, LSAT Test Prep in Washington DC, GRE Test Prep in Washington DC, SSAT Test Prep in Atlanta, MCAT Test Prep in Seattle, SAT Test Prep in Miami, GMAT Test Prep in Denver, SAT Test Prep in Houston

DMCA Complaint

If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing the information described below to the designated agent listed below. If Varsity Tutors takes action in response to an Infringement Notice, it will make a good faith attempt to contact the party that made such content available by means of the most recent email address, if any, provided by such party to Varsity Tutors.

Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects.org.

Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially misrepresent that a product or activity is infringing your copyrights. Thus, if you are not sure content located on or linked-to by the Website infringes your copyright, you should consider first contacting an attorney.

Please follow these steps to file a notice:

You must include the following:

A physical or electronic signature of the copyright owner or a person authorized to act on their behalf; An identification of the copyright claimed to have been infringed; A description of the nature and exact location of the content that you claim to infringe your copyright, in \ sufficient detail to permit Varsity Tutors to find and positively identify that content; for example we require a link to the specific question (not just the name of the question) that contains the content and a description of which specific portion of the question – an image, a link, the text, etc – your complaint refers to; Your name, address, telephone number and email address; and A statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe your copyright is not authorized by law, or by the copyright owner or such owner’s agent; (b) that all of the information contained in your Infringement Notice is accurate, and (c) under penalty of perjury, that you are either the copyright owner or a person authorized to act on their behalf.

Send your complaint to our designated agent at:

Charles Cohn Varsity Tutors LLC
101 S. Hanley Rd, Suite 300
St. Louis, MO 63105

Or fill out the form below:

Do not fill in this field

Contact Information

* Full legal name

Company name

* Phone number

* Email address

Address

* Address1

Address2

* City

* State

* Zipcode

* Country

Complaint Details

* URL of copyrighted work (where your original material is located)

* Please describe the copyrighted work so that it may be easily identified

I am the owner, or an agent authorized to act on behalf of the owner of an exclusive right that is allegedly infringed.

I have a good faith belief that the use of the material in the manner complained of is not authorized by the copyright owner, its agent, or the law.

This notification is accurate.

I acknowledge that there may be adverse legal consequences for making false or bad faith allegations of copyright infringement by using this process.

* Signature (your digital signature is legally binding)

HIGH SCHOOL

GRADUATE SCHOOL

K-8

Search 50+ Tests

math tutoring

science tutoring

foreign languages

elementary tutoring

other

Search 350+ Subjects

PSAT Math : Algebra

Study concepts, example questions & explanations for PSAT Math

All PSAT Math Resources

Example Questions

Example Question #3 : How To Find The Value Of The Coefficient

Example Question #2 : How To Find The Value Of The Coefficient

Example Question #4521 : Algebra 1

Example Question #1 : How To Find The Solution To A Binomial Problem

Example Question #1 : Trinomials

Example Question #2 : Trinomials

Example Question #31 : Polynomials

Example Question #41 : Algebra

Example Question #33 : Polynomials

Example Question #3 : Trinomials

All PSAT Math Resources

Report an issue with this question

DMCA Complaint

Contact Information

Address

Complaint Details

Email address:
Your name:
Feedback: